a, b, c, d, e এবং f গুণোত্তর পদ্ধতিতে থাকলে [ [a², d², x] , [b², e², y] , [c², f²,z] ] নির্ণায়কের মান -
x ও y-এর ওপর নির্ভর করে
y ও z-এর ওপর নির্ভর করে
z ও x-এর ওপর নির্ভর করে
x, y ও z-কারোর ওপরই নির্ভর করে না
The correct Answer is:D
Step by step video, text & image solution for a, b, c, d, e এবং f গুণোত্তর পদ্ধতিতে থাকলে [ [a², d², x] , [b², e², y] , [c², f²,z] ] নির্ণায়কের মান - by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
∫sec6xdx সমাকলন নির্ণয় করতে হলে বসাতে হবে -
02:10View Solution[0, 2] বিস্তারে f(x) ও g(x) অন্তরকলনযােগ্য অপেক্ষক দুটি এমন যে, f''(x)−g''(x)=0, f'(1)=2,g'(1)=4, f(2)=3 এবং g(2) = 9, তাহলে x= 3/2 বিন্দুতে [f(x)−g(x)]-এর মান হবে—
03:25View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
02:07View SolutionD, E, F are the mid-point of the sides BC, CA and AB respectively of ABC. Then DEF is congruent to triangle. ABC (b) AEF (c) BFD, CDE (d) AFE, BFD, CDE
Given : D, E, F are the mid-point of the sides BC, CA and AB .
05:38View SolutionIn Figure, AB⊥BEandFE⊥BE.If BC=DEandAB=EF, then ABD is congruent to:
(a)EFC
(b) ECF
(c) CEF
(d) FEC
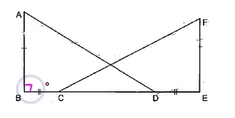 01:14View Solution
01:14View SolutionIf ABC and DEF are two triangles such that AB, BC are respectively equal and parallel to DE, EF, then show that Quadrilateral ABED is a parallelogram Quadrilateral BCFE is a parallelogram AC=DF (iv) ABC ≅ DEF
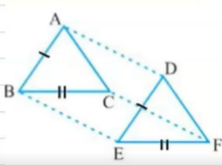 01:53View Solution
01:53View SolutionThe H.C.F. of ab,cd,ef is equal to (a) LCMof a, c, eHCFof b, d, f (b) HCFof a, c, eLCMof b, d, f (c) HCFof a, c, eHCFof b, d, f (d) acebdf
01:52View Solutiona d c e b e d f e h e ?
03:01View Solutionমনে করো, A={a,b,c,d,e} এবং f:A→A চিত্রণ নিম্নরূপে সংজ্ঞাত: f(a)=d,f(b)=a,f(c)=d,f(d)=b এবং f(e)=d,f−1(e) নির্ণয় করো
02:56View Solutionf(b)-f(a)=(b-a)f'(c)(a
03:59View SolutionIf a,b,c,d,e, and fare in G.P. then the value of ∣∣ ∣ ∣∣(a2)(d2)x(b2)(e2)y(c2)(f2)z∣∣ ∣ ∣∣ depends on (A) x and y (B) x and z (C) y and z (D) independent of x ,y ,and z
02:19View SolutionIn Figure, DE∣∣AC and DF∣∣AE . Prove that EFBF=ECBE .
04:50View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=34 , then write Area (ABC):Area(DEF) .
02:06View SolutionIf ABC and DEF are two triangles such that ABDE=BCEF=CAFD=25 , then Area(ABC):Area(DEF)=
01:56View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
04:58View Solution

