A→B+C+D என்ற ஒரு படித்தான வினையில், துவக்க அழுத்தம் P0. 't' நேரத்திற்குப் பின் 'P'. P0, P மற்றும் t ஆகியவற்றைப் பொருத்து வினைவேக மாறிலி
k=(2.303t)log(2p03p0−P)
k=(2.303t)log(2P0P0−P)
k=(2.303t)log(3P0−P2P0)
k=(2.303t)log(2P03P0−2P)
Topper's Solved these Questions
Similar Questions
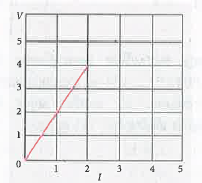
பின்வரும் வரைபடத்தில் ஒரு பெயர் தெரியாத கடத்திக்கு அளிக்கப்பட்ட மின்னழுத்த வேறுபாடு மற்றும் மின்னோட்ட மதிப்புகளின் தொடர்பு காட்டப்பட்டுள்ளது. இந்த கடத்தியின் மின்தடை என்ன?
View Solution273 K மற்றும் 1 atm அழுத்தத்தில் X என்ற ஒரு ஆல்கஹால் விக்டர்மேயர் சோதனையில் நீலநிறத்தினைத் தருகிறது. 3.7g 'X' ஐ உலோக சோடியத்துடன் வினைப்படுத்தும் போது 560 mL ஹைட்ரஜன் வாயு வெளியேறுகிறது. X ன் வடிவ வாய்ப்பாடு என்னவாக இருக்கும்?
View SolutionWhich of the following graphs represents zero order if
A→P
At t=0⇒[A]0
At t=t⇒[A]t
View SolutionP=P0e−(αt2)
find dimensions of α, where P = pressure, t = time.
View SolutionIn a homogenous reaction A rarr B+C+D the initial pressure was P_(0) and after time t it was P. Expression for rate constant k in terms of P_(0) , P and t will be
View SolutionLet A, B and C be three mutually exclusive events such that P(A) = p1,P(B)=p2 and P(C)=p3. Then,
Let p1,p2, and p3 are roots of 27x3−27x2+ax−1=0, then value of a is
View SolutionIn a homogeneous reaction A rarr B + C + D , the initial pressure was P_(0) and after time t it was P. expression for rate constant in terms of P_(0) , P and t will be
View SolutionA→B+C Time t ∝
Total pressure of (B + C) P2 P3 Find k.
View SolutionThe time dependance of a physical quantity 'P' is given by P=P_(0)exp(-at^(2)) , where a is a constant and 't' is time . The constant a is
View Solutionआदर्श विलयन से ऋणात्मक विचलन दर्शाने वाले एक विलयन का कुल वाष्प दाब P हो तथा अवयवों के आंशिक दाब PA और PB हो तो
(अ) P≠PA+PB (ब ) P<PA+PB (स) P<PA+PB (द) P=PA+PB
View SolutionIn a homogeneous reaction
A→B+C+D, the initial pressure was P0 and after time t it was P. Expression for rate constant in terms of P0, P and t will be ………………………
View Solution(COLUMN-I,COLUMN-II),((A)P0A=200,P0B=300,P=250,XA=0.5),((B)P0A=300,P0B=200,P=275,XA=0.25),((C)P0A=400,P0B=400,P=264,XA=0.33),((D)P0A=400,P0B=100,P=290,XA=0.1)
View Solutionदो द्रवों A और B के एक विलयन के लिए यह सिध्द किया गया है कि P=XA(P0A–P0B)+P0B विलयन है।
View SolutionA and B are two events such that P(A)=p1,P(B)=p2,P(A∩B)=p3 then find
(a)P(AC∩B)(b)P(AC∪B)(c)P(AC∩BC)(d)P(AC∪BC)
View SolutionFor ideal binary solution, p=x_(A)*p_(A)^(0)+x_(B)*p_(B)^(0) This equation reflects
View Solution
