If a ray of light in a denser medium strikes a rarer medium at an angle of incidence i, the angle of reflection and refraction are respectively r and r', if the reflected and refracted rays are at right angles to each other, the critical angle for the given pair of media isSolution in Kannada
sin−1(tanr')
sin−1(tanr)
tan−1(sini)
cot−1(tani)
The correct Answer is:A
r+r.=90∘ (given)
∴i+r.=90∘⇒tani=cotr.
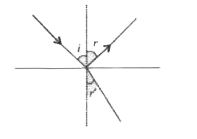
From Brewster.s law tani=μ=1sinC
sinC=1tani=1cotr.=tanr.
⇒C=sin−1(tanr.)
Topper's Solved these Questions
Similar Questions
A ray of light travels from an opticaly denser to a rarer medium. The critical angle for the two media is c. the maximum possible deviation of the ray will be
View SolutionIf M is the mass of the earth and R its radius, the ratio of the gravitational acceleration and the gravitational constant is
View Solution
A ray of light from a denser medium strike a rarer medium at an angle of incidence I (see Fig). The reflected and refracted rays make as angle of 90degrees with each other. The angles of reflection and refraction are r and r The critical angle is
View SolutionA ray of light from a denser medium strikes a rarer medium at an angle of incidenc i. The reflected and refracted rays make an angle of π/2 with each other. If the angles of reflection and refraction are r and r′ , then the criticall angle will be
View Solution
A ray of light from a denser medium strike a rarer medium at an angle of incidence I (see Fig). The reflected and refracted rays make as angle of 90degrees with each other. The angles of reflection and refraction are r and r The critical angle is
View SolutionIf a ray of light in a denser medium strikes a rarer medium at an angle of incidence i, the angles of reflection and refraction are respectively, r and r' If the reflected and refraction rays are at right angles to each other, the critical angle for the given pair of media is
View SolutionA ray of light is incident at an angle i from denser to rare medium. The reflected and the refracted rays are mutually perpendicular. The angle of reflection and the angle of refraction are respectively r and r'. Then the critical angle will be:
View SolutionA ray of light from a rarer medium strikes a denser medium at angle of incidence 60°. The reflected and refracted rays are perpendicular to each other. The refractive index of denser medium and angle of deviation respectively are
View SolutionA ray of light from a denser medium strikes a rarer medium. The reflected and refracted rays make an angle of 90^(@) with each other. The angle of reflection and refraction are r and r^(1) . The critical angle would be
View SolutionA ray of light from a denser medium strikes a rarer medium. The reflected and refracted rays make an angle of 90∘ with each other. The angles of reflection and refraction are r and t1. The critical angle would be
View SolutionA ray of light from a denser medium strikes a rarer medium. The reflected and refracted rays make an angle of 90∘ with each other. The angles of reflection and refraction are r and r'. The critical angle would be
View SolutionIf a ray of light in a denser medium strikes a rarer medium at an angle of incidence i, the angles of reflection and refraction are respectively, r and r' If the reflected and refraction rays are at right angles to each other, the critical angle for the given pair of media is
View SolutionA ray of light from a denser medium strike a rarer medium at an angle of incidence I (see Fig). The reflected and refracted rays make as angle of 90degrees with each other. The angles of reflection and refraction are r and r The critical angle is
View SolutionA ray of light from a denser medium strikes a rare medium as shown in figure. The reflected and refracted rays make an angle of 90∘ with each other. The angles of reflection and refraction are r and r'. The critical angle would be

View SolutionA ray of light is incident at an angle i from denser to rare medium. The reflected and the refracted rays are mutually perpendicular. The angle of reflection and the angle of refraction are respectively r and r'. Then the critical angle will be:
View Solution
