If a ray of light in a denser medium strikes a rarer medium at an angle of incidence i, the angles of reflection and refraction are respectively, r and r' If the reflected and refraction rays are at right angles to each other, the critical angle for the given pair of media is
sin−1(tanr')
sin−1(tanr)
tan−1(sini)
cot−1(tani)
The correct Answer is:B
To solve the problem, we need to find the critical angle (θc) for a ray of light transitioning from a denser medium to a rarer medium, given that the reflected and refracted rays are at right angles to each other.
1. Understanding the Situation:
- We have a ray of light moving from a denser medium (n1) to a rarer medium (n2).
- The angle of incidence is denoted as i, the angle of reflection as r, and the angle of refraction as r′.
- According to the problem, the reflected ray and the refracted ray are at right angles to each other, meaning r+r′=90∘.
2. Using Snell's Law:
- Snell's Law states that:
n1sin(i)=n2sin(r′)
- Since we are moving from a denser medium (n1) to a rarer medium (n2), we can express n2 in terms of n1:
n2=n1n
- Rearranging gives us:
sin(i)=n2n1sin(r′)
3. Relating Angles:
- Since r′=90∘−r, we can substitute this into our equation:
sin(i)=n2n1sin(90∘−r)=n2n1cos(r)
4. Expressing n2 in terms of n1:
- From Snell's Law, we can express n2 as:
n2=n1sin(i)/cos(r)
- This can be simplified to:
n2=n1tan(i)
5. Finding the Critical Angle:
- The critical angle θc occurs when r′=90∘. Thus:
n1sin(θc)=n2
- Substituting n2 gives:
n1sin(θc)=n1tan(i)
- Dividing both sides by n1 (assuming n1≠0):
sin(θc)=tan(i)
6. Final Expression for Critical Angle:
- The critical angle can be expressed as:
θc=sin−1(tan(i))
Conclusion:
Thus, the critical angle for the given pair of media is:
θc=sin−1(tan(i))
- We have a ray of light moving from a denser medium (n1) to a rarer medium (n2).
- The angle of incidence is denoted as i, the angle of reflection as r, and the angle of refraction as r′.
- According to the problem, the reflected ray and the refracted ray are at right angles to each other, meaning r+r′=90∘.
- Snell's Law states that:
n1sin(i)=n2sin(r′)
- Since we are moving from a denser medium (n1) to a rarer medium (n2), we can express n2 in terms of n1:
n2=n1n
- Rearranging gives us:
sin(i)=n2n1sin(r′)
- Since r′=90∘−r, we can substitute this into our equation:
sin(i)=n2n1sin(90∘−r)=n2n1cos(r)
- From Snell's Law, we can express n2 as:
n2=n1sin(i)/cos(r)
- This can be simplified to:
n2=n1tan(i)
- The critical angle θc occurs when r′=90∘. Thus:
n1sin(θc)=n2
- Substituting n2 gives:
n1sin(θc)=n1tan(i)
- Dividing both sides by n1 (assuming n1≠0):
sin(θc)=tan(i)
- The critical angle can be expressed as:
θc=sin−1(tan(i))
Thus, the critical angle for the given pair of media is:
θc=sin−1(tan(i))
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - medical entrance special format question23videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Medical entrance gallary76videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Checkpoint 9.710videos
RAY OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - NUCLEIEXERCISE - C MADICAL ENTRANCES GALLERY46videos
NUCLEI
- BOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective9videos
REFLECTION OF LIGHT
Similar Questions
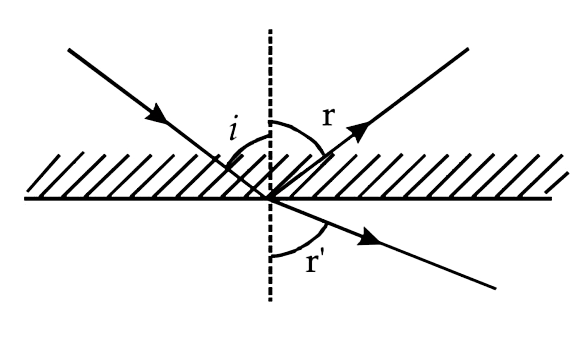
A ray of light from a denser medium strike a rarer medium at an angle of incidence I (see Fig). The reflected and refracted rays make as angle of 90degrees with each other. The angles of reflection and refraction are r and r The critical angle isView SolutionA ray of light from a denser medium strikes a rarer medium at an angle of incidence i=tan−1(1/2). If the reflected and the refracted rays are mutually perpendicular to each-other, what is the value of the critical angle in degree?
 View Solution
View SolutionA ray of light entering from air to glas (μ=1.5) is partly reflected and partly refracted. lf the reflected and refracted rays are at right angles to each other, the angle of refraction is
View SolutionA ray of light from a denser medium strikes a rare medium as shown in figure. The reflected and refracted rays make an angle of 90∘ with each other. The angles of reflection and refraction are r and r'. The critical angle would be
 View Solution
View SolutionLight from a denser medium 1 passes to a rarer medium 2. When the angle of incidence is θ the partially reflected and refracted rays are mutually perpendicular. The critical angle will be
 View Solution
View SolutionWrite a relationship between angle of incidence and angle of refraction for a given pair of media.
View SolutionA ray of light is incident on a transparent medium at polarizing angle. What is the angle between the reflected ray and the refracted ray .
View SolutionA ray is reflected in turn by three plain mirrors mutually at right angles to each other. The angle between the incident and the reflected rays is
View SolutionIf the angle of reflection is 45∘, what is the angle between the incident ray and the reflected ray?
View SolutionA light ray is incident fromt a denser medium on the boundary separating it from a rarer medium at an angle of incidence equal to the critical angle. What is the angle of refraction for the ray ?
View Solution

