Question

The area enclosed by the curves$y=\sin x+\cos x\text{and}y=|\cos x-\sin x|$ over the interval $[0,\frac{\pi }{2}]$

Options

A. `4(sqrt(2)-1)`
B. `2sqrt(2)(sqrt(2)-1)`
C. `2(sqrt(2)+1)`
D. `2sqrt(2)(sqrt(2)+1)`

Answer

B

Explanation

PLAN To find the bounded area between `y=f(x) " and " y=g(x)` between x = a to x = b.

` therefore " Area bounded " =int_(a)^(c)[g(x)-f(x)]dx+int_(c)^(b)[f(x)-g(x)]dx `
`=int_(a)^(b)|f(x)-g(x)|dx`
Here, `f(x)=y=sinx+cosx," when " 0 le x le (pi)/(2) `
and `g(x)=y=|cosx-sinx|`
`={(cosx-sinx ",", 0 le x le (pi)/(4) ),(sinx-cosx ",", (pi)/(4) le x le (pi)/(4)):} `
could be shown as

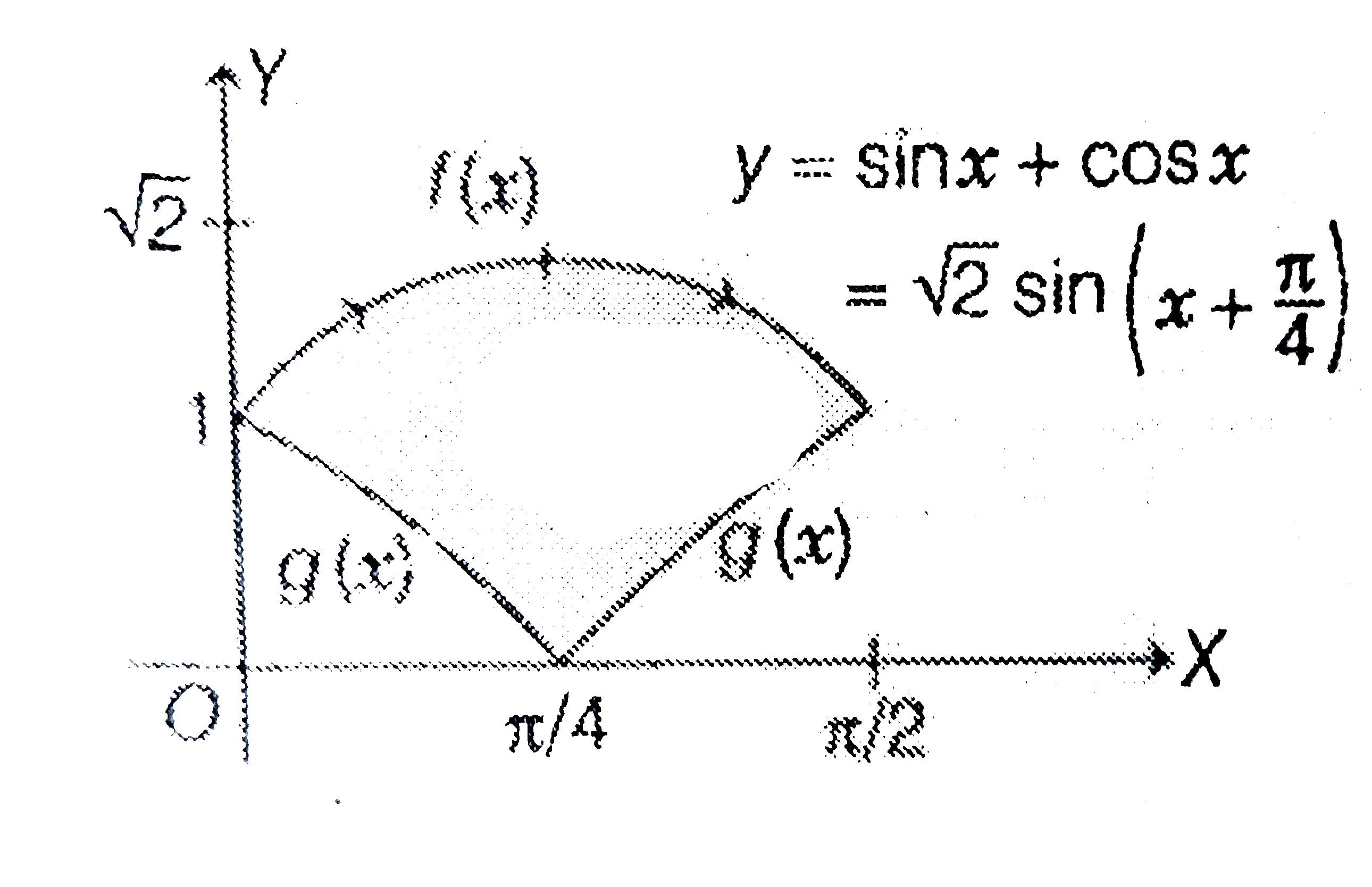
` therefore " Area bounded " = int_(0)^(pi//4){(sinx+cosx)-(cosx-sinx)}dx +int_(pi//4)^(pi//2){(sinx+cosx)-(sinx-cosx)}dx `
` = int_(0)^(pi//4)2sinx dx + int_(pi//4)^(pi//2) 2 cosxdx `
`=-2[cosx]_(0)^(pi//4)+2[sinx*n]_(pi//4)^(pi//2)`
`=4-2sqrt(2)=2sqrt(2)(sqrt(2)-1)` sq units