A metal bar of length l is well lagged with some non-conducting material to prevent losses of heat from its surface and the two ends are maintained at steady temperatures θ1 and θ2 with θ1>θ2, then in the steady state the temperature θ of s section of the bar at distance x from the hot end varies as shown in the graph




The correct Answer is:B
Topper's Solved these Questions
- THERMAL PROPERTIES OF MATTER BOOK - DISHA PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTER EXERCISE - Exercise-1: Concept Builder (Topicwise)
69 Videos
- SYSTEM OF PARTICLES & ROTATIONAL MOTION BOOK - DISHA PUBLICATIONCHAPTER - SYSTEM OF PARTICLES & ROTATIONAL MOTION EXERCISE - EXERCISE-2 : CONCEPT APPLICATOR
28 Videos
- THERMODYNAMICS BOOK - DISHA PUBLICATIONCHAPTER - THERMODYNAMICS EXERCISE - EXERCISE -2 : CONCEPT APPLICATOR
29 Videos
Similar Questions
The ends of a metal rod are kept at temperature θ1andθ2 with θ2>θ1. At steady state the rate of flow of heat along the rod is directly proportional to
View SolutionThe ends of a metal rod are kept at temperatures θ1 and θ2 with θ2>θ1. The rate of flow of heat along the rod is directly proportional to
View Solution
Knowledge Check
The ends of a metal rod are kept at temperature θ1andθ2 with θ2>θ1. At steady state the rate of flow of heat along the rod is directly proportional to
Athe length of the rodBthe diameter of the rodCthe cross-sectional area of the rodDthe temperature difference (θ2−θ1) between the ends of the rodThe ends of a metal rod are kept at temperatures θ1 and θ2 with θ2>θ1. The rate of flow of heat along the rod is directly proportional to
Athe length of the rodBthe diameter of the rodCthe cross sectional area of the rodDthe temperature difference (θ2−θ1) between the ends of the rodTwo different metal rods of the same length have their ends kept at the same temperature θ1, and θ2with θ2>θ1. If A1 and A2 are their cross-sectional areas and K1 and K2 their thermal conductivities, the rate of flow of heat in the two rods will be the same it:
AA1A2=k1k2BA1A2=k2k1CA1A2=k1θ1k2θ2DA1A2=k2θ2k1θ1
A thin rod, length L0 at 0∘C and coefficient of linear expansion α has its two ends mintained at temperatures θ1 and θ2 respectively Find its new length .
View SolutionTwo conducting slabs of heat conductivity K1 and K2 are joined as shown in fig. The temp. at ends of the slabs are θ1 and θ2(θ1>θ2) the, final temp. (θm) of junction is :

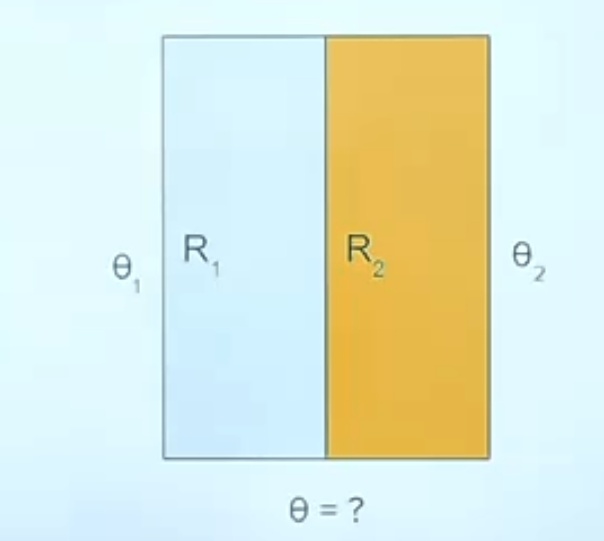
View SolutionTwo slabs are placed adjacently having thermal resistance R1&R2, their free ends maintained at temperature of θ1, & and θ2, respectively. Find the temperature of junction.
View SolutionA long metallic bar is carrying heat from one of its ends to the other end under steady-state. The variation of temperature θ along the length x of the bar from its hot end is best described by which of the following figure.
View SolutionA thin rod, length L0 at 0∘C and coefficient of linear expansion α has its two ends mintained at temperatures θ1 and θ2 respectively Find its new length .
View Solution
