Question

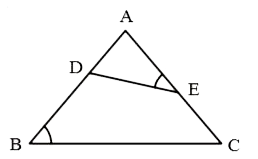
D and E are points on sides AB and AC of $△ABC$ DE is parallel to BC if AD: DB =1 :2 and area of $△ABC$ is 45 sq cm what is the area of quadrialteral BDEC

Options

A. 20
B. 40
C. 15
D. 30

Answer

2

Explanation

To solve the problem, we need to find the area of quadrilateral BDEC in triangle ABC, given that DE is parallel to BC, AD:DB = 1:2, and the area of triangle ABC is 45 sq cm.

1. Understanding the Ratios:
- We have AD:DB = 1:2. This means if we let AD = 1x, then DB = 2x. Therefore, AB (which is AD + DB) = 1x + 2x = 3x.

2. Finding the Area of Triangle ADE:
- Since DE is parallel to BC, triangles ADE and ABC are similar by the Basic Proportionality Theorem (also known as Thales' theorem).
- The ratio of the areas of similar triangles is equal to the square of the ratio of their corresponding sides.
- The ratio of the sides AD to AB is given by:
\( \frac{AD}{AB} = \frac{1}{3} \)
- Therefore, the ratio of the areas of triangle ADE to triangle ABC is:
\( \left(\frac{AD}{AB}\right)^2 = \left(\frac{1}{3}\right)^2 = \frac{1}{9} \)

3. Calculating the Area of Triangle ADE:
- The area of triangle ABC is given as 45 sq cm.
- Using the area ratio, we can find the area of triangle ADE:
\( \text{Area of } \triangle ADE = \text{Area of } \triangle ABC \times \frac{1}{9} = 45 \times \frac{1}{9} = 5 \text{ sq cm} \)

4. Finding the Area of Quadrilateral BDEC:
- The area of quadrilateral BDEC can be found by subtracting the area of triangle ADE from the area of triangle ABC:
\( \text{Area of } BDEC = \text{Area of } \triangle ABC - \text{Area of } \triangle ADE = 45 - 5 = 40 \text{ sq cm} \)

Final Answer:
The area of quadrilateral BDEC is 40 sq cm.