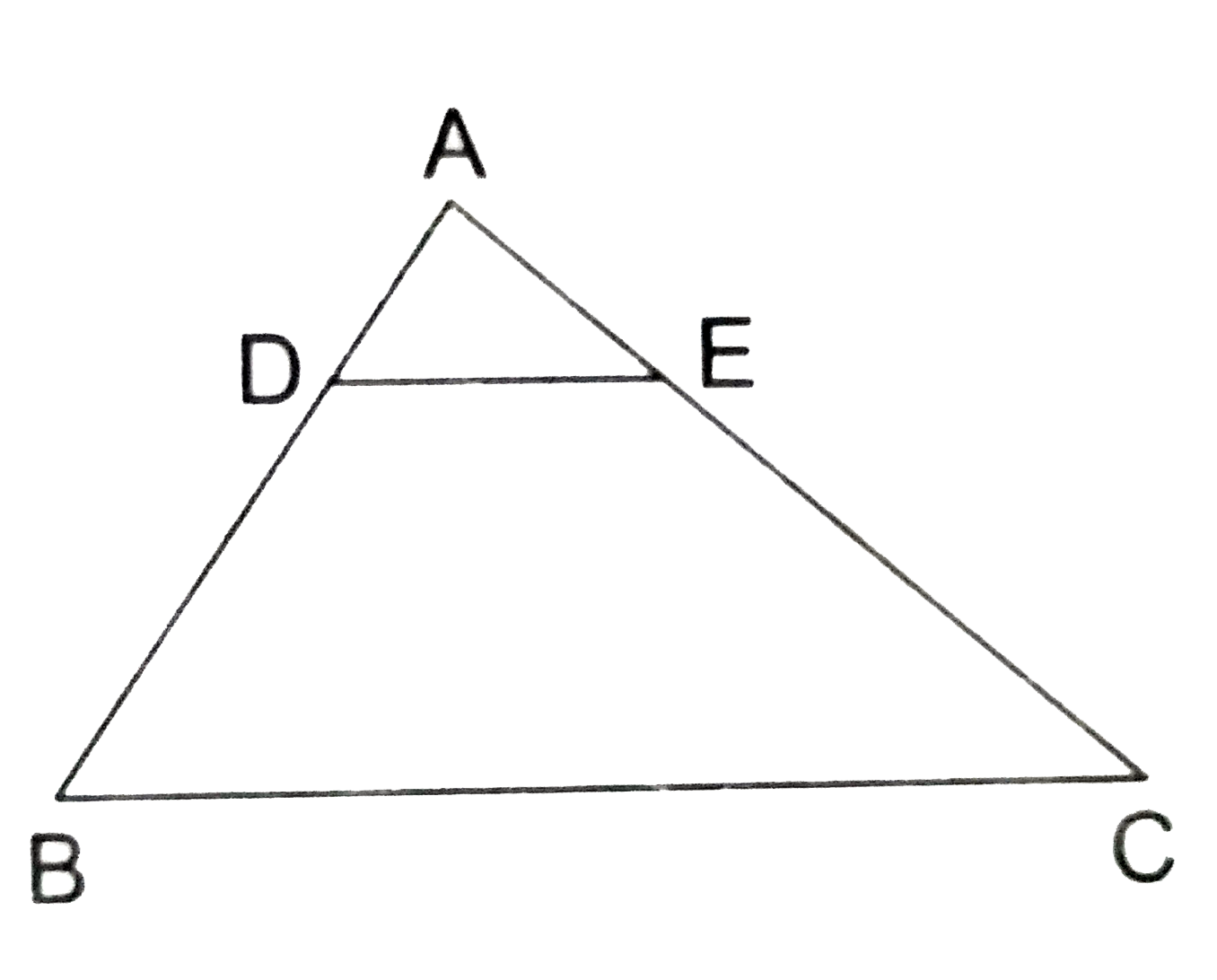
Let D, E be the points on sides AB and AC respectively of a triangle ABC such that DE is parallel to BC. Let AD = 2 cm, DB = 1 cm, AE = 3 cm and area of triangle ADE = 3 cm2 What is EC equal to ?
1.5 cm
1.6 cm
18 cm
2.1 cm
To solve the problem step-by-step, we start by analyzing the given information and applying the properties of similar triangles.
Step 1: Understand the Triangle and Given Information
We have triangle ABC with points D and E on sides AB and AC respectively such that DE is parallel to BC. We are given:
- AD=2cm
- DB=1cm
- AE=3cm
- Area of triangle ADE = 3cm2
We need to find the length of EC, which we will denote as x.
Step 2: Calculate the Length of AB and AC
First, we can find the total lengths of sides AB and AC:
- AB=AD+DB=2+1=3cm
- AC=AE+EC=3+x
Step 3: Use the Property of Similar Triangles
Since DE is parallel to BC, triangles ADE and ABC are similar. Therefore, the ratios of their corresponding sides are equal:
ADAB=AEAC
Substituting the known values:
23=33+x
Step 4: Cross-Multiply to Solve for x
Cross-multiplying gives us:
2(3+x)=3⋅3
2(3+x)=9
Step 5: Expand and Simplify
Expanding the left side:
6+2x=9
Step 6: Isolate x
Now, isolate x by subtracting 6 from both sides:
2x=9−6
2x=3
Step 7: Divide to Find x
Now, divide both sides by 2:
x=32=1.5cm
Conclusion
Thus, the length of EC is 1.5cm.
---
We have triangle ABC with points D and E on sides AB and AC respectively such that DE is parallel to BC. We are given:
- AD=2cm
- DB=1cm
- AE=3cm
- Area of triangle ADE = 3cm2
First, we can find the total lengths of sides AB and AC:
- AB=AD+DB=2+1=3cm
- AC=AE+EC=3+x
Since DE is parallel to BC, triangles ADE and ABC are similar. Therefore, the ratios of their corresponding sides are equal:
ADAB=AEAC
23=33+x
Cross-multiplying gives us:
2(3+x)=3⋅3
2(3+x)=9
Expanding the left side:
6+2x=9
Now, isolate x by subtracting 6 from both sides:
2x=9−6
2x=3
Now, divide both sides by 2:
x=32=1.5cm
Thus, the length of EC is 1.5cm.
Similar Questions
Knowledge Check
If D and E are points on the sides AB and AC respectively of a triangle ABC such that DE || BC. If AD =x cm, DB =(x-3) cm, AE = ( x+ 3 ) cm and EC = ( x -2) cm, what is the value (in cm. ) of x ?
A3B3.5C4D4.5If D and E are points on the sides AB and AC respectively of a triangle ABC such that DE || BC. If AD =x cm, DB =(x-3) cm, AE = ( x+ 3 ) cm and EC = ( x -2) cm, what is the value (in cm. ) of x ?
A3B3.5C4D4.5In ΔABC, D and E are points on AB and AC respectively such that DE is parallel to BC. If AD= 2 cm, BD = 3 cm, then ar(ΔADE)ar(ΔABC) is
A49B1681C425D25
If D and E are points on the sides AB and AC respectively of DetlaABC such that AB=5.6cm,AD=1.4cm,AC=7.2cmandAE=1.8cm. Show that DE∣∣BC.
View SolutionLet D, E and F be the middle points of the sides BC,CA and AB respectively of a triangle ABC. Then, AD + BE + CF equals to
View SolutionD and E are points on sides AB and AC of ΔABC. DE is parallel to BC. If AD : DB = 2 : 3, what is the ratio of area ΔADE and area of quadrilateral BDEC ?
View SolutionIn ΔABC D and E are the points on sides AB and AC, respectively, such that DE || BC. If AD = 4 cm, BC = 5 cm. DC = 10 cm and AB = 15 cm. then the sum of the lengths of DE and EC is:
View SolutionD and E are points on sides AB and AC of △ABC DE is parallel to BC if AD: DB =1 :2 and area of △ABC is 45 sq cm what is the area of quadrialteral BDEC
View Solution
