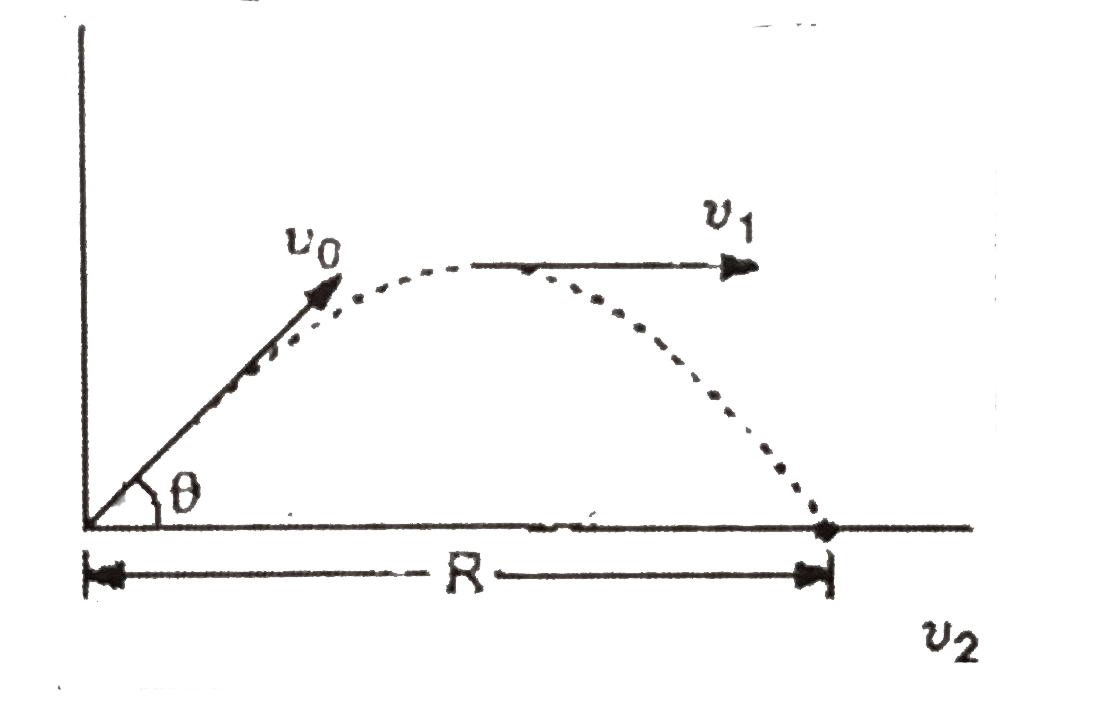
A stone is projected at a speed $v_{0}$ and it makes an angle $θ$ with the horizontal. Find the angular velocity of the projectile at the time when it reaches the same level with respect to the point of projection at the time it touches the ground.

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is: $7.0$

To find the angular velocity of a projectile at the moment it reaches the same level as the point of projection, we can follow these steps:
1. Understand the Problem:
- A stone is projected with an initial speed $v_{0}$ at an angle $θ$ with the horizontal. We need to find the angular velocity $ω$ when the stone returns to the same height from which it was projected.
2. Identify Components of Velocity:
- The initial velocity can be broken down into horizontal and vertical components:
- Horizontal component: $v_{0 x} = v_{0} cos θ$
- Vertical component: $v_{0 y} = v_{0} sin θ$
3. Determine the Velocity at the Same Level:
- When the stone returns to the same level, the horizontal component of the velocity remains unchanged: $v_{x} = v_{0} cos θ$ .
- The vertical component of the velocity just before hitting the ground will be $v_{y} = - v_{0} sin θ$ (the negative sign indicates that it is directed downwards).
4. Calculate the Perpendicular Velocity:
- The perpendicular component of the velocity with respect to the point of projection at the moment of impact is the vertical component:
$v_{⊥} = v_{0} sin θ$
5. Determine the Distance $r$ :
- The distance $r$ from the point of projection to the point where the stone hits the ground is the range of the projectile, given by:
$R = \frac{v_{0}^{2} sin 2 θ}{g}$
6. Calculate Angular Velocity $ω$ :
- The formula for angular velocity $ω$ is given by:
$ω = \frac{v_{⊥}}{r}$
- Substituting the values we have:
$ω = \frac{v_{0} sin θ}{\frac{v_{0}^{2} sin 2 θ}{g}}$
- Simplifying this expression:
$ω = \frac{v_{0} sin θ \cdot g}{v_{0}^{2} sin 2 θ}$
- Since $sin 2 θ = 2 sin θ cos θ$ , we can rewrite:
$ω = \frac{g}{2 v_{0} cos θ}$
7. Final Result:
- Thus, the angular velocity $ω$ of the projectile at the moment it touches the ground is:
$ω = \frac{g}{2 v_{0} cos θ}$

Updated on:18/9/2024

|

Class 12 PHYSICS CIRCULAR MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

A projectile is projected with speed u at an angle $θ$ with the horizontal . The average velocity of the projectile between the instants it crosses the same level is
Au cos $θ$
Bu sin $θ$
Cu cot $θ$
Du tan $θ$
A projectile is projected with a velocity $v_{0}$ at an angle $θ$ with the horizontal as shown in figure. The angular momentum of particle about the origin:

Ais zero when particle is at the origin
Bis $\frac{- m v^{3} {sin}^{2} θ cos θ}{2 g} ˆ k$ when particle is at the highest point of trajectory
Cis $\frac{- 2 m v^{2} {sin}^{2} θ cos θ}{g} ˆ k$ when particle is just about to hit ground
Ddownward force of gravity exerts a torque in $- z$ direction.
A particle I projected at an angle of elevation $α$ after time $t$ it makes an angle $β$ with horizontal. The velocity of projection is
A $\frac{2 g t cos β}{sin (α - β)}$
B $\frac{g t cos β}{sin (α - β)}$
C $\frac{g t}{2 sin (α - β)}$
D $\frac{4 g t}{sin (α - β)}$

RESNICK AND HALLIDAY-CIRCULAR MOTION-PRACTICE QUESTIONS(INTEGER TYPE)

Home
Ask Doubt
Profile