In Fig. , block 1 of mass m1 slides from rest along a frictionless ramp from height h = 3.00 m and then collides with stationary block 2, which has mass m2=2.00m1. After the collision, block 2 slides into a region where the coefficient of kinetic friction μk is 0.450 and comes to a stop in distance d within that region. What is the value of distance d if the collision is (a) elastic and (b) completely inelastic?

The correct Answer is:(a)2.96 m ; (b) 0.741 m
Step by step video & image solution for In Fig. , block 1 of mass m_1 slides from rest along a frictionless ramp from height h = 3.00 m and then collides with stationary block 2, which has mass m_2 = 2.00m_1. After the collision, block 2 slides into a region where the coefficient of kinetic friction mu_k is 0.450 and comes to a stop in distance d within that region. What is the value of distance d if the collision is (a) elastic and (b) completely inelastic? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (Single Correct Choice )
47 Videos
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (More than One Correct Choice)
5 Videos
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (Integer )
4 Videos
- CAPACITANCEBOOK - RESNICK AND HALLIDAYCHAPTER - CAPACITANCEEXERCISE - PRACTICE QUESTION (INTEGER TYPE)
3 Videos
- CIRCUITSBOOK - RESNICK AND HALLIDAYCHAPTER - CIRCUITSEXERCISE - Practice Questions (Integer Type)
3 Videos
Similar Questions
Block 1, with mass m1 and speed 3.0 m/s, slides along an x axis on a frictionless floor and then undergoes a one dimensional elastic collision with stationary block 2, with mass m2=0.40m1. The two blocks then slide into a region where the coefficient of kinetic friction is 0.50, there they stop. How far into that region do (a) block 1 and (b) block 2 slide?
View SolutionA block of mass m moving at speed υ collides wilth another block of mass 2 m at rest. The lighter block comes to rest after the collision. Find the coefficient of restitution.
View Solution
Knowledge Check
A block of mass M is sliding down the plane. Coefficient of static friction is μs and kinetic friction is μk. Then friction force acting on the block is :
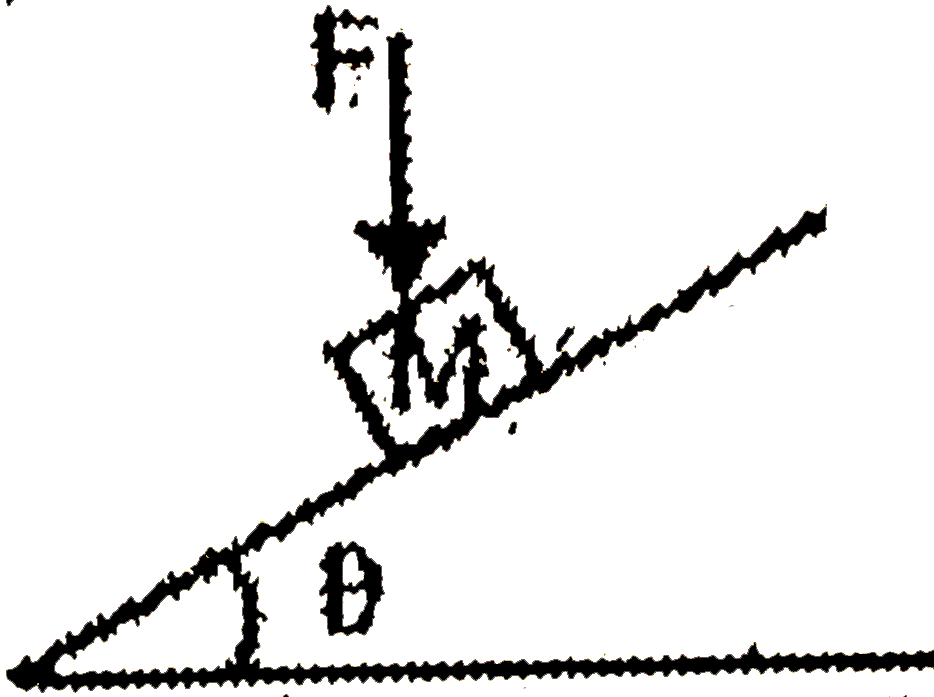 A(F+Mg)sinθBμk(F+Mg)cosθCμsMgcosθD(Mg+F)sinθ
A(F+Mg)sinθBμk(F+Mg)cosθCμsMgcosθD(Mg+F)sinθTwo blocks of mass 2m and m slide down the slope AB having same friction coefficient Then:
 Athe heavier mass reaches the bottom in a shorter timeBboth the masses have the same kinetic energy on arriving at BCthe heavier mass has twice the momentum than that of the other when reaching BDthe lighter mass reaches the bottom in a shorter time
Athe heavier mass reaches the bottom in a shorter timeBboth the masses have the same kinetic energy on arriving at BCthe heavier mass has twice the momentum than that of the other when reaching BDthe lighter mass reaches the bottom in a shorter timeA block having mass m collides with an another stationary block having mass 2m. The lighter block comes to rest after collision. If the velocity of first block is v, then the value is coefficient of restitution will must be
A0.5B0.4C0.6D0.8
Block 1 of mass m1 slides along a frictionless floor and into a one-dimensional elastic collision with stationary block 2 of mass m2=3m1. Prior to the collision, the center of mass of the two block system had a speed of 3.00 m/s. Afterward, what are the speeds of (a) the center of mass and (b) block 2 ?
View SolutionIn Fig. a stationary block explodes into two pieces L and R that slide across a frictionless floor and then into regions with friction, where they stop. Piece L. with a mass of 2.0 kg, encounters a coefficient of kinetic friction μL = 0.35 and slides to a stop in distance dL =0.15 m. Piece R encounters a coefficient of kinetic friction μR=0.50 and slides to a stop in distance dR =0.30 m. What was the mass of the block?
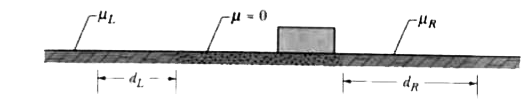
View SolutionA block of mass m slides from the top of an inclined plane of angle of inclination θ & length l. The coefficient of friction between the plane and the block is μ. Then it is observed over a distance d along the horizontal surface having the same coefficient of friction μ, before it comes to a stop. Find the value of d.
View SolutionA block having mass m collides with an another stationary block having mass 2m. The lighter block comes to rest after collision. If the velocity of first block is v, then the value is coefficient of restitution will must be
View SolutionA block of mass m slides from rest at a height H on a frictionless inclined plane as shown in the figure. It travels a distance d across a rough horizontal surface with coefficient of kinetic friction μ , and compresses a spring of spring k by a distance x before coming to rest momentarily. Then the spring extends and the block travels back attaining a final height of h. Then
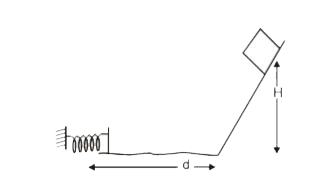
View Solution
