A tow truck is pulling a car out of a ditch by means of steel cable that is 9.1 m long and has a radius of 0.50 cm. When the car just begins to move, the tension in the cable is 890 N. How much has the cable stretched ?
2.6×10−6m
2.3×10−2m
1.7×10−4m
5.2×10−4m
The correct Answer is:D
To solve the problem of how much the steel cable has stretched when the tow truck pulls the car out of the ditch, we can use the formula derived from Young's modulus. Here’s the step-by-step solution:
Step 1: Identify the given values
- Length of the cable (L) = 9.1 m
- Radius of the cable (r) = 0.50 cm = 0.005 m (conversion from cm to m)
- Tension in the cable (F) = 890 N
- Young's modulus for steel (Y) = 2×1011N/m2
Step 2: Calculate the cross-sectional area (A) of the cable
The cross-sectional area of a circular cable is given by the formula:
A=πr2
Substituting the radius:
A=π(0.005)2=π(0.000025)≈7.85×10−5m2
Step 3: Use Young's modulus to find the extension (ΔL)
Young's modulus is defined as:
Y=StressStrain=F/AΔL/L
Rearranging this gives:
ΔL=FLAY
Substituting the known values:
ΔL=890×9.17.85×10−5×2×1011
Step 4: Calculate ΔL
Calculating the numerator:
890×9.1=8099
Calculating the denominator:
7.85×10−5×2×1011=1.57×107
Now substituting these values into the equation:
ΔL=80991.57×107≈0.000515m
Step 5: Convert ΔL to a more convenient unit
ΔL≈5.15×10−4m=0.515mm
Step 6: Round off the answer
Rounding off gives:
ΔL≈5.2×10−4m
Final Answer
The cable has stretched approximately 5.2×10−4m or 0.52mm.
---
- Length of the cable (L) = 9.1 m
- Radius of the cable (r) = 0.50 cm = 0.005 m (conversion from cm to m)
- Tension in the cable (F) = 890 N
- Young's modulus for steel (Y) = 2×1011N/m2
The cross-sectional area of a circular cable is given by the formula:
A=πr2
Substituting the radius:
A=π(0.005)2=π(0.000025)≈7.85×10−5m2
Young's modulus is defined as:
Y=StressStrain=F/AΔL/L
Rearranging this gives:
ΔL=FLAY
Substituting the known values:
ΔL=890×9.17.85×10−5×2×1011
Calculating the numerator:
890×9.1=8099
Calculating the denominator:
7.85×10−5×2×1011=1.57×107
Now substituting these values into the equation:
ΔL=80991.57×107≈0.000515m
ΔL≈5.15×10−4m=0.515mm
Rounding off gives:
ΔL≈5.2×10−4m
The cable has stretched approximately 5.2×10−4m or 0.52mm.
Topper's Solved these Questions
- ELASTICITYBOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)
8 Videos
- ELASTICITYBOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - PRACTICE QUESTIONS (Linked Comprehension)
6 Videos
- ELASTICITYBOOK - RESNICK AND HALLIDAYCHAPTER - ELASTICITYEXERCISE - CHECKPOINT
1 Videos
- CURRENT AND RESISTANCEBOOK - RESNICK AND HALLIDAYCHAPTER - CURRENT AND RESISTANCEEXERCISE - Practice Questions (Integer Type)
3 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )
3 Videos
Similar Questions
A 1500 kg cable car moves vertically by means of a cable that connects the ground and the top of a hill. What is the tension in the supporting cable when the cab, originally moving downard at a speed of 9.0 m/s, is brought to rest with constant acceleration in a distance of 38 m.
View SolutionThe drum of a winch has mass M and radius R. A cable wound aroung the drum suspends a load of mass m. The entire cable has a length L and mass per unit length λ, with a total mass mc=Lλ. The load begins to fall all toward the ground, unwinding the cable as it goes. How fast is the load moving after it has falled a distance d?
View Solution
Knowledge Check
A truck is pulling a car out of a ditch by means fo a steel cable that is 9.1m long and has a radius of 5mm. When the car just beings to move, the tension in the cable is 800N. If Young's modulus for steel is 2×1011N/m2 then the strecth in the cable is (neraly)
A5×10−3mB0.5×10−3mC3×10−3mD0.3×10−3mThe cross section of a cable is shown in fig. The inner conductor has a radius of 10mm and the dielectric has a thickness of 5mm. The cable is 8km. Long. Then the capacitance of the cable is [loge1.5=0.4].
 .A3.8μFB1.1μFC4.8×10−10μFD3.3μF
.A3.8μFB1.1μFC4.8×10−10μFD3.3μFA helicopter of mass M is lowering a truck of mass m onto the deck of a ship. In first case the helicopter and the truck move downward together (the length of the cable remaine constant). Tension in the cable is T1 when their downward speed is decreasing at a rate of (g)/(10). In second case when the truck gets close to the deck, the helicopter stops moving downward. While it hovers stationary, it lets out the cabel so that the truck is still moving downward. If the truck is moving downward with a speed decreasing at rate of g10, tension in string is now T2, What is ratio T1/T2
A1011B911C1DNone
An amusement park ride consists of airplane shaped cars attached to steel rods. Each rod has a length of 20.0m and a cross-sectional area of 8.00cm2. Young's modulus for steel is 2×1011N/m2.
a. How much is the rod stretched (in mm) when the ride is at rest ? (Assume that each car plus two people seated in it has a total weight of 2000N.)
b. When operating, the ride has a maximum angular speed of √195rad/s. How much is the rod stretched (in mm) then?

View SolutionA steel wire 0.72 m long has a mass of 5.0×10−3 kg . If the wire is under a tension of 60 N, what is the speed of transverse waves on the wire ?
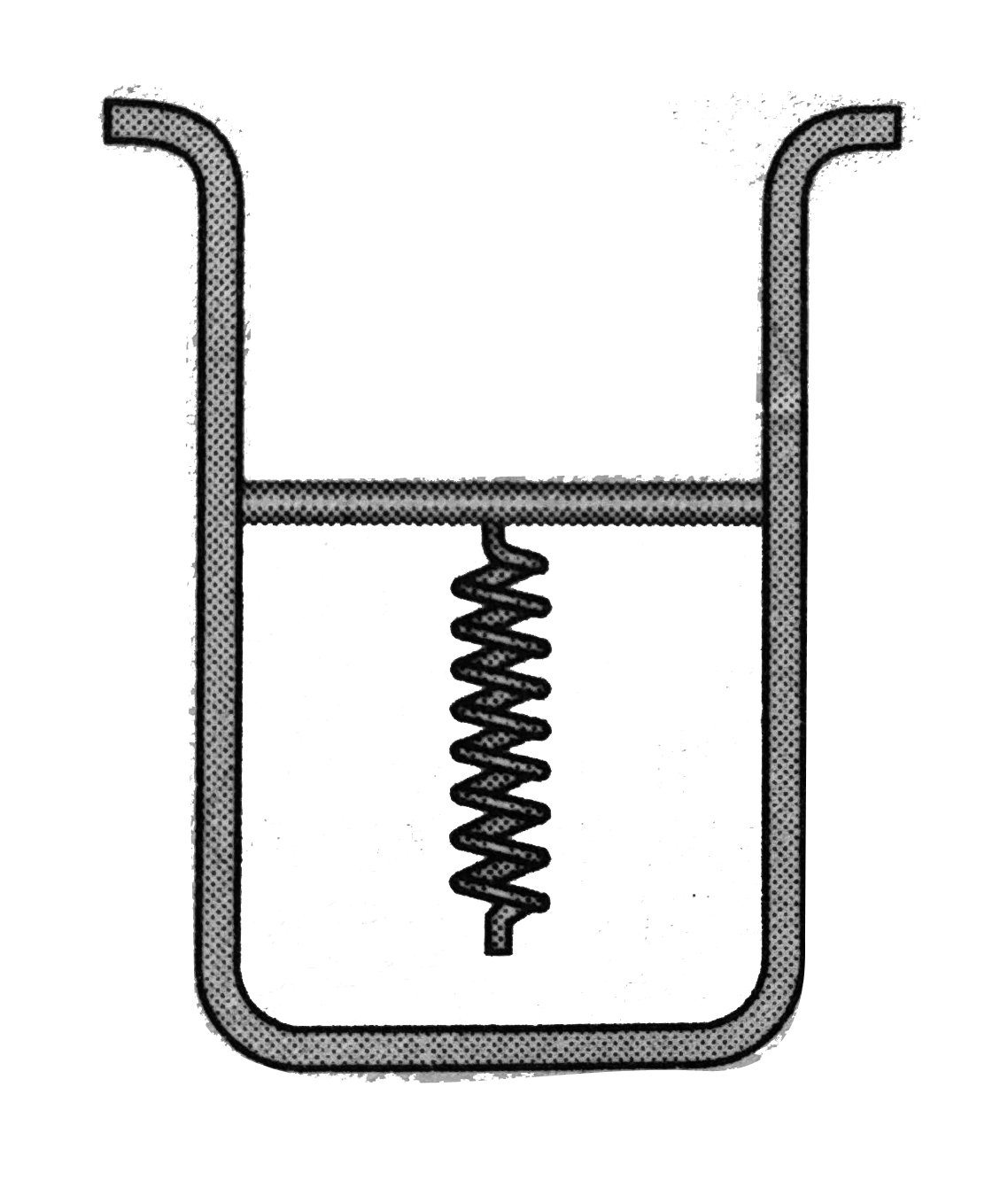
View SolutionA cylinder is fitted with a piston, beneath which is a spring, as in the figure. The cylinder is open at the top. Friction is absent. The spring is 3600N/m. The piston has a negligible mass and a radius of 0.025m (a) when air beneath the piston is conpletely pumped out, how much does the atmospheric pressure cause the spring to compress? (b) How much work does the atmospheric pressure do in compressing the spring?
View SolutionIn Fig. 10-64, block 1 has mass m1=460g, block 2 has mass m2=500g, and the pulley, which is mounted on a horizontal axle with negligible friction, has radius R = 5.00 cm. When released from rest, block 2 falls 75.0 cm in 5.00 s without the cord slipping on the pulley. (a) What is the magnitude of the acceleration of the blocks? What are (b) tension T2 and ( c) tension T1? (d) What is the magnitude of the pulley's angular acceleration? (e) What is its rotational inertia?

View SolutionA steel piano wire 0.5 m long has a total mass of 0.01 kg and is stretched with a tension of 800 N. The frequency, when it vibrates in its fundamental mode, is
View Solution
