Two large parallel metal plates are 1.5 cm apart and have charges of equal magnitudes but opposite signs on their facing surfaces. Take the potential of the negative plate to be zero. If the potential halfway between the plates is then , what is the electric field in the region between the plates?
To find the electric field between two parallel plates with given potentials, we can follow these steps:
Step 1: Understand the Problem
We have two large parallel plates that are 1.5 cm apart. The negative plate has a potential of 0 V, and the potential halfway between the plates is +10.0 V. We need to find the electric field (E) in the region between the plates.
Step 2: Identify the Relevant Formula
The electric field (E) between two parallel plates can be calculated using the formula:
E=Vd
where:
- V is the potential difference between the plates,
- d is the distance between the plates.
Step 3: Determine the Potential Difference
Since the negative plate is at 0 V and the potential halfway between the plates is +10.0 V, the potential difference (V) between the negative plate and the halfway point is:
V=10.0V
Step 4: Calculate the Distance
The total distance between the plates is given as 1.5 cm. To use this in our formula, we need to convert it to meters:
d=1.5cm=0.015m
Step 5: Calculate the Electric Field
Since we are considering the potential difference from the negative plate to the halfway point, we need to take half the distance between the plates for our calculation:
d=1.5cm2=0.0075m
Now we can substitute the values into the electric field formula:
E=10.0V0.0075m
Step 6: Perform the Calculation
Calculating the electric field:
E=10.00.0075=1333.33V/m
Final Answer
The electric field in the region between the plates is:
E=1333.33V/m
---
We have two large parallel plates that are 1.5 cm apart. The negative plate has a potential of 0 V, and the potential halfway between the plates is +10.0 V. We need to find the electric field (E) in the region between the plates.
The electric field (E) between two parallel plates can be calculated using the formula:
E=Vd
where:
- V is the potential difference between the plates,
- d is the distance between the plates.
Since the negative plate is at 0 V and the potential halfway between the plates is +10.0 V, the potential difference (V) between the negative plate and the halfway point is:
V=10.0V
The total distance between the plates is given as 1.5 cm. To use this in our formula, we need to convert it to meters:
d=1.5cm=0.015m
Since we are considering the potential difference from the negative plate to the halfway point, we need to take half the distance between the plates for our calculation:
d=1.5cm2=0.0075m
E=10.0V0.0075m
Calculating the electric field:
E=10.00.0075=1333.33V/m
The electric field in the region between the plates is:
E=1333.33V/m
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Single Correct Choice Type21videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) More than One Correct Choice Type8videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - CHECKPOINT6videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )3videos
ELECTRIC CHARGES AND FIELDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Integer Type)14videos
ELECTROMAGNETIC INDUCTION
Similar Questions
Two large metal plates of area 6.0m2 face each other . The plates are 3 cm apart and carry equal and opposite charges on their inner surfaces. If electric field at a point between the plates is 5×104NC−1, then calculate the magnitude of charge on each plates.
View SolutionIn a parallel plate capacitor the potential difference of 100 V is manitianed between the plates. If distance between the plates be 5mm, what will be the electric field at points A and B ?
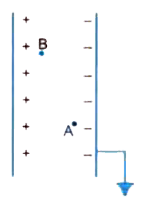 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
For uniform electric field, potential difference between the plate is
A1ε0QAdB1ε0AdQCε0QAdD1ε0QdA - Question 2 - Select One
The surface charge densities on two square plates of a parallel plate capacitor are to +σand−σ respectively. What is the magnitude of the electric field in the region between the plates?
Aσ2ε0Bσε0C0DNone of these - Question 3 - Select One
When a metal slab is placed between the charged identical , parallel plates , the potential difference between the plates
AdecreasesBincreasesCremains unchanged.Dmay increase or decrease
A parallel plate capacitor of plate area A and plates separation distance d is charged by applying a potential V0 between the plates. The dielectric constant of the medium between the plates is K. What is the uniform electric field E between the plates of the capacitor ?
View SolutionA uniformly distributed space charge fills up the space between two large parallel plates separated by a distance d. The potential difference between the plates is equal to zero ? What will then be the field strength near the other plate ?
View SolutionTwo plates are at potentials −10V and +30V. If the separation between the plates be 2cm. The electric filed between them is
View SolutionTwo plates are 2cm apart, a potential difference of 10 volt is applied between them, the electric field between the plates is
View SolutionTwo large metal plates are placed parallel to each other The inner surfaces of plates are charge by +σand−σ(Cm−2) The outer surfaces are neutral .The electric field in the negion between the plates and outside the plates is
View Solution

