A point object with a charge +Q is placed at the center of a conducting shell of inner radius R, outer radius 2R, and a charge of −4Q. A thin - walled conducting shell of radius 3 R and a charge of +4Q is concentric with the point object and the first shell. Defining V = 0 at infinity, find all the distances from the center at which the electric potential is zero .
To solve the problem, we need to analyze the electric potential due to the charges and find the distances from the center where the potential is zero.
1. Understanding the System:
- We have a point charge +Q at the center of a conducting shell with inner radius R and outer radius 2R, which has a charge of −4Q.
- There is another conducting shell with radius 3R and charge +4Q that is concentric with the first shell.
2. Charge Induction:
- The point charge +Q will induce a charge of −Q on the inner surface of the first conducting shell (since the shell must remain neutral).
- This means the outer surface of the first shell will have a charge of −4Q+Q=−3Q.
3. Electric Field and Potential:
- Inside the conducting shell (from 0 to R), the electric field E=0 (since it's a conductor in electrostatic equilibrium). Thus, the potential V is constant and equal to the potential at the surface of the inner shell.
- The potential V at the inner surface (radius R) due to the point charge +Q is given by:
V(R)=kQR
where k is Coulomb's constant.
4. Potential in the Region Between the Shells:
- Between R and 2R (inside the first shell), the potential remains constant and equal to V(R).
- At the surface of the outer shell (radius 2R), the potential is:
V(2R)=kQ2R+k(−3Q)2R=kQ2R−3kQ2R=−2kQ2R=−kQR
5. Potential Outside the Outer Shell:
- For r>2R, the total charge is +Q−4Q+4Q=+Q. Therefore, the potential at a distance r is:
V(r)=kQr
6. Finding Points Where Potential is Zero:
- We need to find distances r where V(r)=0.
- The potential is zero when:
kQr=0
This does not occur for any finite r since kQ is a constant and r cannot be infinite.
- However, we also need to consider the regions:
- Inside the inner shell: V=kQR (not zero)
- Between the shells: V=kQR (not zero)
- At the outer shell: V=−kQR (not zero)
- Outside the outer shell: V(r)=kQr (approaches zero as r→∞)
7. Conclusion:
- The electric potential is zero at infinity. There are no other points where the potential is zero in the defined regions.
Summary of Distances Where Electric Potential is Zero:
- The only distance from the center where the electric potential is zero is at r=∞.
- We have a point charge +Q at the center of a conducting shell with inner radius R and outer radius 2R, which has a charge of −4Q.
- There is another conducting shell with radius 3R and charge +4Q that is concentric with the first shell.
- The point charge +Q will induce a charge of −Q on the inner surface of the first conducting shell (since the shell must remain neutral).
- This means the outer surface of the first shell will have a charge of −4Q+Q=−3Q.
- Inside the conducting shell (from 0 to R), the electric field E=0 (since it's a conductor in electrostatic equilibrium). Thus, the potential V is constant and equal to the potential at the surface of the inner shell.
- The potential V at the inner surface (radius R) due to the point charge +Q is given by:
V(R)=kQR
where k is Coulomb's constant.
- Between R and 2R (inside the first shell), the potential remains constant and equal to V(R).
- At the surface of the outer shell (radius 2R), the potential is:
V(2R)=kQ2R+k(−3Q)2R=kQ2R−3kQ2R=−2kQ2R=−kQR
- For r>2R, the total charge is +Q−4Q+4Q=+Q. Therefore, the potential at a distance r is:
V(r)=kQr
- We need to find distances r where V(r)=0.
- The potential is zero when:
kQr=0
This does not occur for any finite r since kQ is a constant and r cannot be infinite.
- However, we also need to consider the regions:
- Inside the inner shell: V=kQR (not zero)
- Between the shells: V=kQR (not zero)
- At the outer shell: V=−kQR (not zero)
- Outside the outer shell: V(r)=kQr (approaches zero as r→∞)
- The electric potential is zero at infinity. There are no other points where the potential is zero in the defined regions.
- The only distance from the center where the electric potential is zero is at r=∞.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) Single Correct Choice Type21videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - (PRACTICE QUESTIONS) More than One Correct Choice Type8videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC POTENTIALEXERCISE - CHECKPOINT6videos
ELECTRIC POTENTIAL
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Questions (Integer Type )3videos
ELECTRIC CHARGES AND FIELDS
- BOOK - RESNICK AND HALLIDAYCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - PRACTICE QUESTIONS (Integer Type)14videos
ELECTROMAGNETIC INDUCTION
Similar Questions
A point charge +q is placed at the centre of a conducting spherical shell of inner radius a and outer radius b. Find the charge appearing on the inner and outer surface of the shell.
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?View Solution
Knowledge Check
- Question 1 - Select One or More
A conducting sphere of radius R, carrying charge Q, lies inside uncharged conducting shell of radius 2R. If they are joined by a metal wire,
AQ/3 amount of charge will flow from the sphere to the shellB20/3 amount of charge will flow from the sphere to the shellCQ amount of charge will flow from the sphere to the shellDkQ24R amount of heat will be produced - Question 2 - Select One
A thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
A(a) 2Q4πε0RB(b) 2Q4πε0R−2q4πε0RC(c) 2Q4πε0R+q4πε0RD(d) (q+Q)24πε0R - Question 3 - Select One
A thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
A2Q4πε0R−2q4πε0RB2Q4πε0R+q4πε0RC(q+Q)4πε02RD2Q4πε0R
A point charge Q is placed inside a conducting spherical shell of inner radius 3R and outer radius 5R at a distance R from the centre of the shell. The electric potential at the centre of the shell will be
View SolutionA point charge q is brought slowly from infinity and is placed at the centre of a conducting neutral spherical shell of inner radius a and outer radius b, then find work done by external agent :
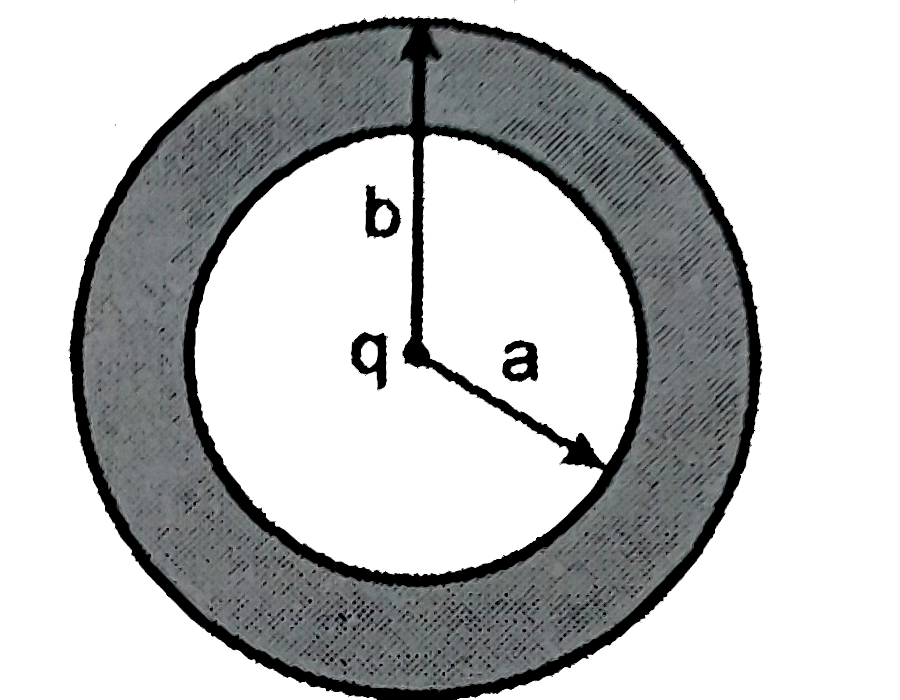 View Solution
View SolutionInside a charged thin conducting shell of radius R, a point charge +Q is placed as shown in figure potential of shell may be
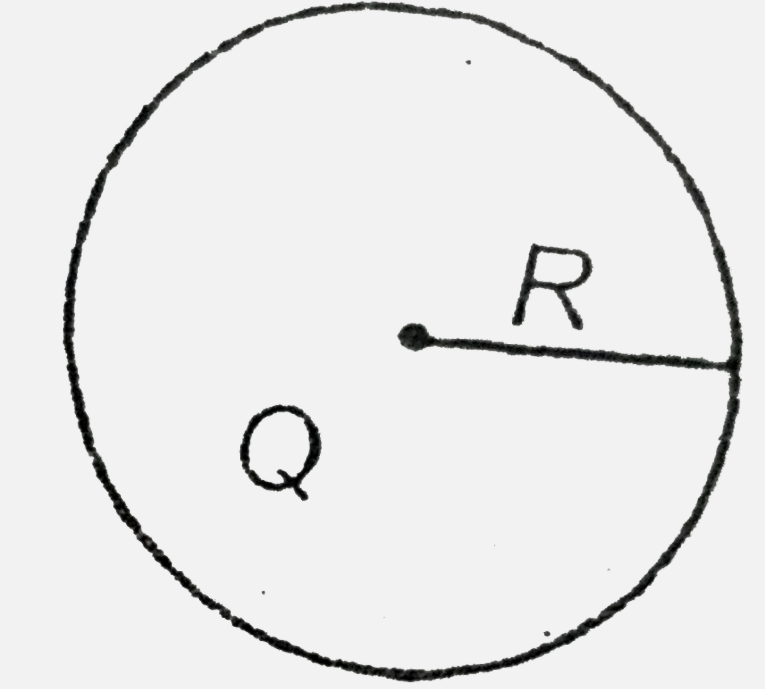 View Solution
View SolutionA point charge q is placed inside a conducting spherical shell of inner radius 2R and outer radius 3R at a distance of R from the center of the shell. Find the electric potential at the center of the shell.
View SolutionA point charge q is placed inside a conducting spherical shell of inner radius 2R and outer radius 3R at a distance of R fro the centre of the shell. The electric potential at the centre of shell will (potential at infinity is zero).
View Solution

