An equilateral triangle of side 6 cm has its corners cut off to form a regular hexagon. Area (in cm2) of this regular hexagon will be
3√3
3√6
6√3
5√32
The correct Answer is:C
To find the area of the regular hexagon formed by cutting off the corners of an equilateral triangle with a side length of 6 cm, we can follow these steps:
1. Calculate the Area of the Equilateral Triangle:
The formula for the area A of an equilateral triangle with side length a is given by:
A=√34a2
Substituting a=6 cm:
A=√34×62=√34×36=9√3 cm2
2. Determine the Area of the Corners Cut Off:
When the corners of the triangle are cut off to form a regular hexagon, each corner removed is an equilateral triangle with a side length of 2 cm (since the original triangle's side length is 6 cm, and each corner cut removes 2 cm from each side).
The area of one small equilateral triangle (corner) is:
Acorner=√34×22=√34×4=√3 cm2
Since there are 3 corners cut off:
Atotal corners=3×√3=3√3 cm2
3. Calculate the Area of the Hexagon:
The area of the hexagon can be found by subtracting the total area of the corners from the area of the original triangle:
Ahexagon=Atriangle−Atotal corners=9√3−3√3=6√3 cm2
Final Answer:
The area of the regular hexagon is:
6√3 cm2
The formula for the area A of an equilateral triangle with side length a is given by:
A=√34a2
Substituting a=6 cm:
A=√34×62=√34×36=9√3 cm2
When the corners of the triangle are cut off to form a regular hexagon, each corner removed is an equilateral triangle with a side length of 2 cm (since the original triangle's side length is 6 cm, and each corner cut removes 2 cm from each side).
The area of one small equilateral triangle (corner) is:
Acorner=√34×22=√34×4=√3 cm2
Since there are 3 corners cut off:
Atotal corners=3×√3=3√3 cm2
The area of the hexagon can be found by subtracting the total area of the corners from the area of the original triangle:
Ahexagon=Atriangle−Atotal corners=9√3−3√3=6√3 cm2
The area of the regular hexagon is:
6√3 cm2
Similar Questions
Knowledge Check
What is the area (in sq cm.) of the regular hexagon side 14 cm ?
A147√3B441√3C196√3D294√3If the apothem of a regular hexagon is a, find each side of the hexagon.
AA)3a√2BB)√3aCC)0.67aDD)2√3aAn equilateral triangle is cut from its three vertices to form a regular hexagon . What is the percentage of area wasted ?
AA)20%BB)50%CC)33.33%DD)66.66%
An equilateral triangle of area 300cm2 is cut from its three vertices to form a regular hexagon. Area of hexagon is what percent of the area of triangle?
View Solutiona and b form the consecutive sides of a regular hexagon ABCDEF.
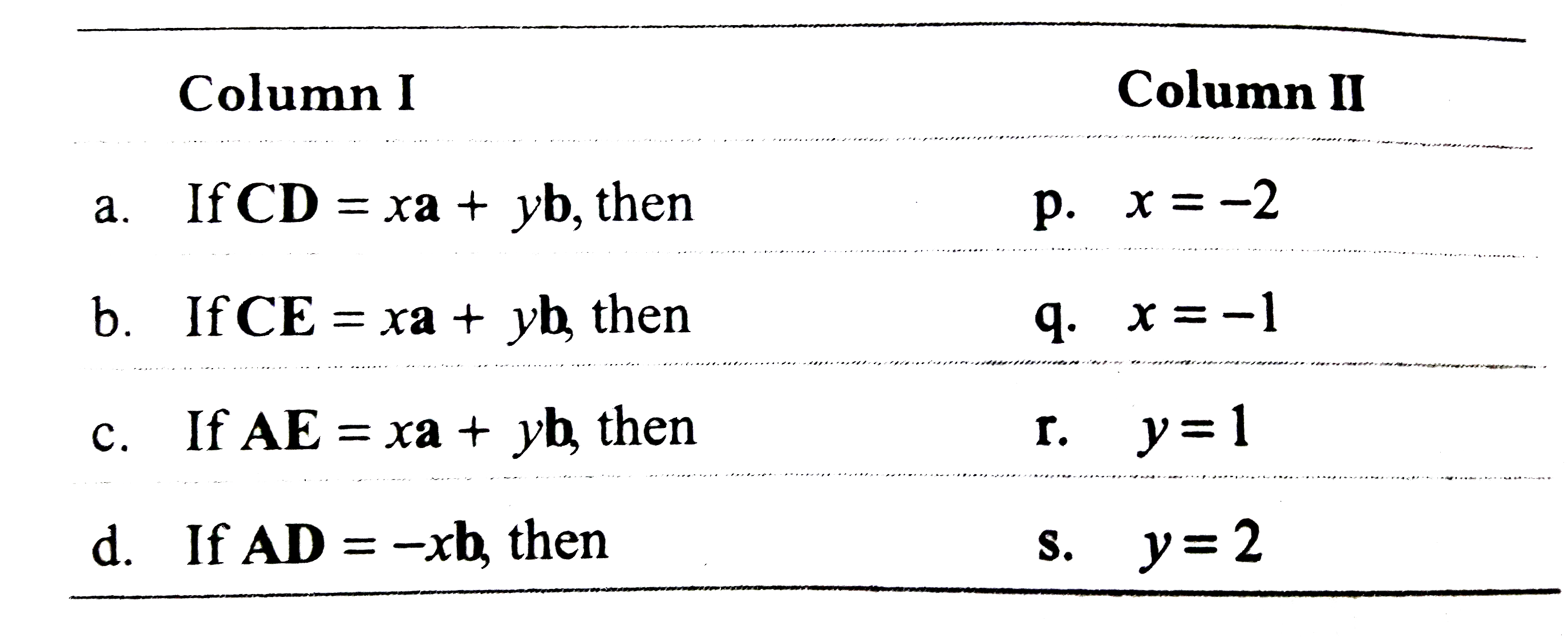
View SolutionFind the area of a regular hexagon of side 6 cm .
View SolutionWhat is the area (in sq cm) of a regular hexagon of side 14 cm?
View SolutionAn equilateral triangle is cut from its three vertices to form a regular hexagon . What is the percentage of area wasted ?
View Solution
