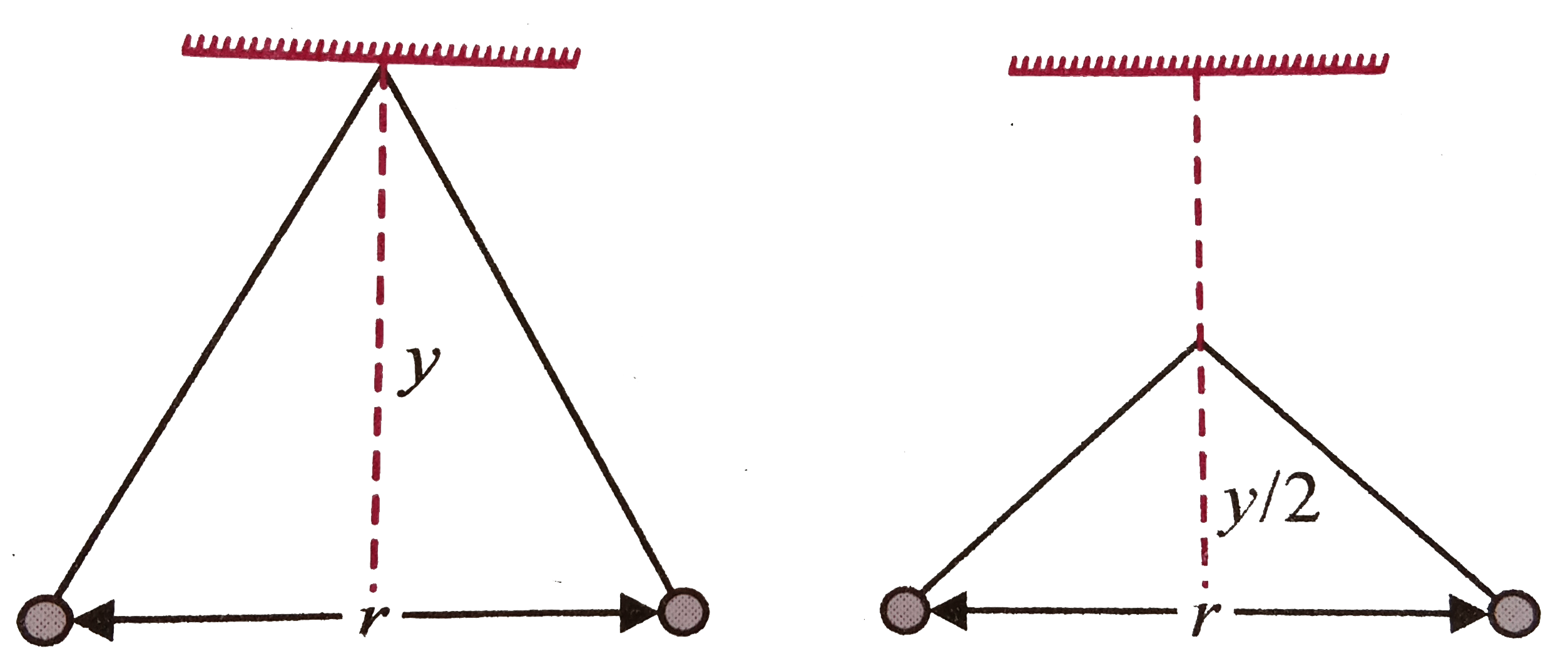
Two pith balls carrying equal charges are suspended from a common point by strings of equal length. The equilibrium separation between them is r. Now the strings are rigidly clamped at half the height . The equilibrium separation between the balls now become .

A

$(\frac{r}{3 \sqrt{2}})$

B

$(\frac{2 r}{\sqrt{3}})$

C

$(\frac{2 r}{3})$

D

${(\frac{r}{\sqrt{2}})}^{2}$

Text Solution

Verified by Experts

The correct Answer is:A

from figure , $tan θ = \frac{F_{e}}{m g} \Rightarrow \frac{r / 2}{y} = \frac{\frac{k q^{2}}{r^{2}}}{m g}$
[ $∴ F = \frac{k q^{2}}{r^{2}}$ from coulomb.s law]
$\Rightarrow r^{3} \propto y \Rightarrow r .^{3} \propto \frac{Y}{2} \Rightarrow \frac{r .}{r} = \frac{1}{2^{1 / 3}} \Rightarrow r . = \frac{r}{3 \sqrt{2}}$

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two path balls carrying eqaul chareges are suspended froom a common point by strings of equal length, the strings are rightly clamped at half the height. The equilibrium separation between the balls, now becomes :

A $(\frac{2 r}{3})$
B ${(\frac{1}{\sqrt{2}})}^{2}$
C $(\frac{r}{\sqrt[3]{2}})$
D $(\frac{2 r}{\sqrt{3}})$
Question 2 - Select One
Two identical tennis balls each having mass 'm' and charge 'q' are suspended from a fixed point by threads of length 'l'. What is the equilibrium separation when each thread makes a small angle $' θ'$ with the vertical ?
A $x = {(\frac{q^{2} l}{2 π ε_{0} m g})}^{1 / 2}$
B $x = {(\frac{q^{2} l}{2 π ε_{0} m g})}^{1 / 3}$
C $x = {(\frac{q^{2} l^{2}}{2 π ε_{0} m^{2} g})}^{1 / 3}$
D $x = {(\frac{q^{2} l^{2}}{2 π ε_{0} m^{2} g^{2}})}^{1 / 3}$

DISHA PUBLICATION-ELECTRIC CHARGES AND FIELDS -Exercise - 1 Concept builder (Topicwise)

Home
Ask Doubt
Profile