Two identical pith balls are charged by rubbing against each other. They are suspended from a horizontal rod through two strings of length 20 cm each, the separation between the suspension points being 5 cm. in equilibrium, the separation between the balls in 3 cm. Find the mass of each ball and the tension in the strings. the charge on each ball a magnitude
To solve the problem, we will follow these steps:
Step 1: Understand the Setup
We have two identical pith balls, each with a charge of q=2.0×10−8C. They are suspended from a horizontal rod by strings of length L=20cm=0.2m. The separation between the suspension points is d=5cm=0.05m, and the equilibrium separation between the balls is s=3cm=0.03m.
Step 2: Calculate the Electrostatic Force
The electrostatic force F between the two charged balls can be calculated using Coulomb's law:
F=kq1q2r2
where k=9×109N m2/C2 is Coulomb's constant, q1=q2=q, and r=s=0.03m.
Substituting the values:
F=9×109(2.0×10−8)2(0.03)2
Calculating:
F=9×1094.0×10−160.0009=9×109×4.0×10−169×10−4=4.0×10−3N
Step 3: Analyze Forces Acting on Each Ball
In equilibrium, the forces acting on each ball are:
1. The tension T in the string acting at an angle θ to the vertical.
2. The weight of the ball mg acting downwards.
3. The electrostatic force F acting horizontally.
From the equilibrium conditions, we can write two equations:
1. In the vertical direction:
Tcosθ=mg(1)
2. In the horizontal direction:
Tsinθ=F(2)
Step 4: Determine the Geometry
Since the balls are displaced, we can find θ using the geometry of the setup. The horizontal displacement of each ball from the vertical line is x=d−s2=5cm−3cm2=1cm=0.01m.
Using the right triangle formed:
sinθ=xL=0.010.2=0.05
cosθ=√1−sin2θ=√1−(0.05)2≈√0.9975≈0.99875
Step 5: Substitute and Solve for Mass m
From equation (2):
T=Fsinθ=4.0×10−30.05=0.08N
Now substituting T into equation (1):
0.08cosθ=mg
0.08×0.99875=mg
mg≈0.0799N
Using g≈9.81m/s2:
m=0.07999.81≈0.00814kg≈8.14g
Final Results
- The mass of each ball is approximately 8.14g.
- The tension in the strings is 0.08N.
We have two identical pith balls, each with a charge of q=2.0×10−8C. They are suspended from a horizontal rod by strings of length L=20cm=0.2m. The separation between the suspension points is d=5cm=0.05m, and the equilibrium separation between the balls is s=3cm=0.03m.
The electrostatic force F between the two charged balls can be calculated using Coulomb's law:
F=kq1q2r2
where k=9×109N m2/C2 is Coulomb's constant, q1=q2=q, and r=s=0.03m.
F=9×109(2.0×10−8)2(0.03)2
Calculating:
F=9×1094.0×10−160.0009=9×109×4.0×10−169×10−4=4.0×10−3N
In equilibrium, the forces acting on each ball are:
1. The tension T in the string acting at an angle θ to the vertical.
2. The weight of the ball mg acting downwards.
3. The electrostatic force F acting horizontally.
1. In the vertical direction:
Tcosθ=mg(1)
2. In the horizontal direction:
Tsinθ=F(2)
Since the balls are displaced, we can find θ using the geometry of the setup. The horizontal displacement of each ball from the vertical line is x=d−s2=5cm−3cm2=1cm=0.01m.
sinθ=xL=0.010.2=0.05
cosθ=√1−sin2θ=√1−(0.05)2≈√0.9975≈0.99875
From equation (2):
T=Fsinθ=4.0×10−30.05=0.08N
0.08cosθ=mg
0.08×0.99875=mg
mg≈0.0799N
m=0.07999.81≈0.00814kg≈8.14g
- The mass of each ball is approximately 8.14g.
- The tension in the strings is 0.08N.
Topper's Solved these Questions
- ELECTRIC FIELD AND POTENTIALBOOK - HC VERMACHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 2
8 Videos
- ELECTRIC CURRENT THROUGH GASESBOOK - HC VERMACHAPTER - ELECTRIC CURRENT THROUGH GASESEXERCISE - Exercises
23 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - HC VERMACHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE
9 Videos
Similar Questions
Two identical pith balls are charged by rubbing against each other. They are suspended from a horizontal rod through two strings of length 20 cm each, the separation between the suspension points being 5 cm. in equilibrium, the separation between the balls in 3 cm. Find the mass of each ball and the tension in the strings. the charge on each ball a magnitude 2.0×10−8C.
View SolutionTwo indentical pith balls, each carrying charge q, are suspended from a common point by two strings of equal length l. Find the mass of each ball if the angle between the strings is 2θ in equilibrium.
View Solution
Knowledge Check
Two path balls carrying eqaul chareges are suspended froom a common point by strings of equal length, the strings are rightly clamped at half the height. The equilibrium separation between the balls, now becomes :
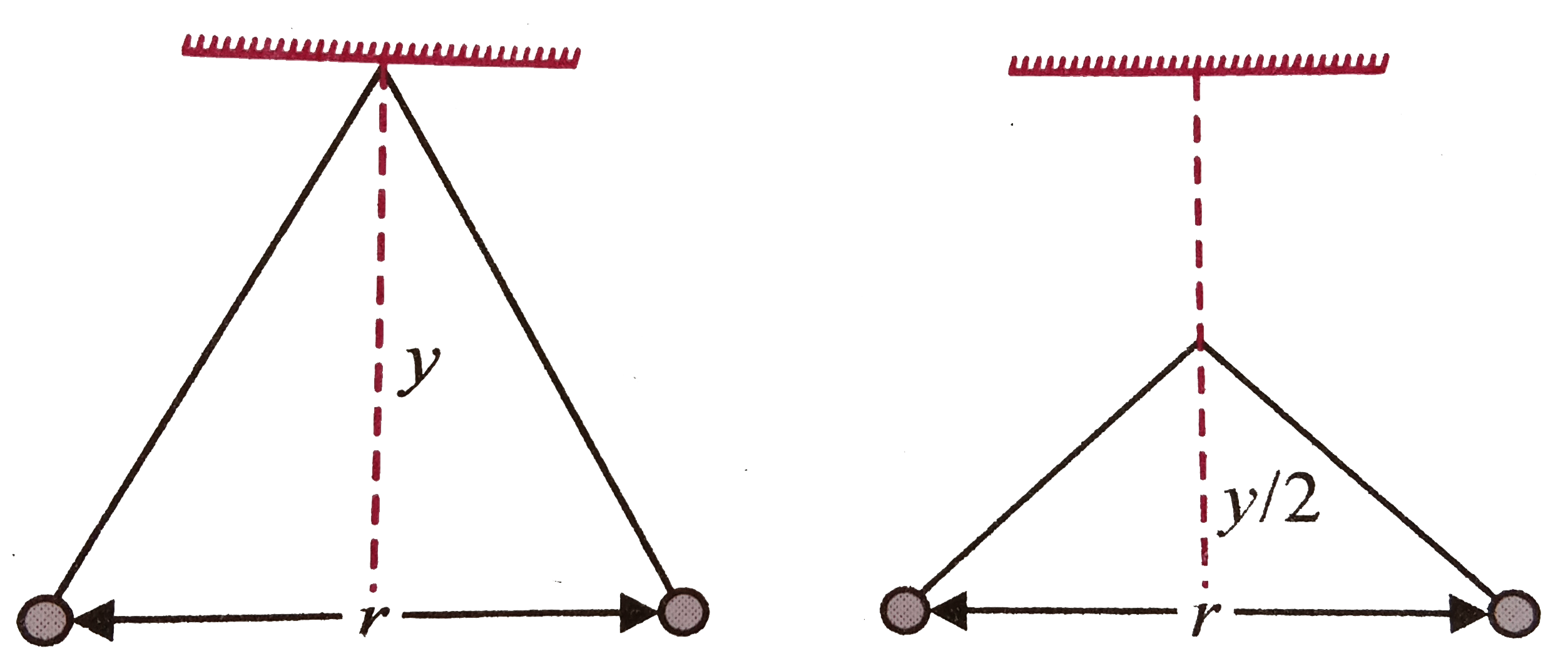 A(2r3)B(1√2)2C(r3√2)D(2r√3)
A(2r3)B(1√2)2C(r3√2)D(2r√3)Assertion : Two identical balls are charged by q. They are suspended from a common point by two insulating threads l each. In equilibrium, the angle between the tension in the threads is 180∘. (Ignore gravity).
Reason : In equilibrium tension in the spring is
T=14πε0q.ql2AIf both Assertion and Reason are correct and Reason is the correct explanation of AssertionBIf both Assertion and Reason are correct but Reason is not the correct explanation of AssertionCIf Assertion is true but Reason is falseDIf Assertion is false but Reason is trueTwo identical tennis balls each having mass 'm' and charge 'q' are suspended from a fixed point by threads of length 'l'. What is the equilibrium separation when each thread makes a small angle 'θ' with the vertical ?
Ax=(q2l2πε0mg)1/2Bx=(q2l2πε0mg)1/3Cx=(q2l22πε0m2g)1/3Dx=(q2l22πε0m2g2)1/3
Two pitch balls carrying equal charges are suspended from a common point by strings of equal length, the equilibrium separation between them is r. Now the strings are rigidly clamped at half the height. The equilibrium separation between the balls now become
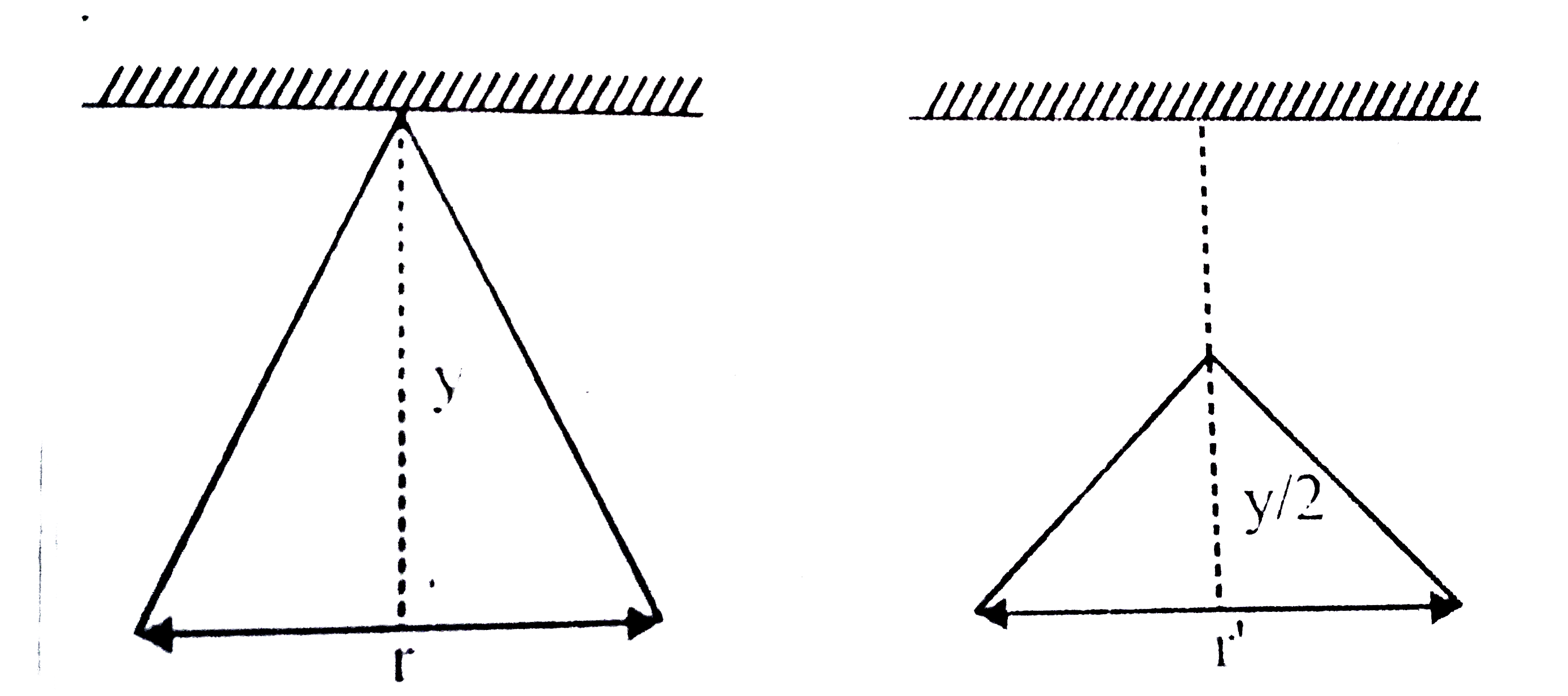
View SolutionTwo small spheres, each having a mass of 20 g, are suspended form a common point by two insulating strings of length 40 cm each. The spheres are identically charged and the speration between the balls at equilibrium is found to be 4 cm . Find the charge on each sphere.
View SolutionTwo idential pith balls , each of mass m and charge +q. are suspended from a point with threads of length l each. If in equiilbrium state θ be the angle which each thread makes with vertical in equilibrium , find value of charge on each ball.
View SolutionTwo small identical balls P and Q, each of mass 0.866 g, carry identical charges and are suspended by two threads of equal length 50 cm each from the same point. At equilibrium, they are separated by a distance of 50 cm. Find the charge on each ball (in nearest integer in nC).
View SolutionTwo identical balls , each having a charge q and mass m , are suspended from a common point by two insultating strings each of length L. The balls are held at a separation x and then released. Find
(a) the electric force on each ball
(b) the component of the resultant force on a ball along and perpendicular to string
(c ) the tension in the string
(d) the acceleration of one of the balls. Consider the situation only for the instant just after the release.
View Solution
