Three coherent, equal intensity light rays arrive at a point Pon a screen to produce an interference minimum of zero intensity. If any two of the rays are blocked, the intensity of the light at P is I1. What is the intensity of the light at P if only one of the rays is blocked?
0
I1/2
I1
2I1
The correct Answer is:C
To solve the problem, we need to analyze the situation involving three coherent light rays arriving at point P on a screen. The conditions given are that these rays produce an interference minimum of zero intensity when all three are present, and that if any two of the rays are blocked, the intensity at P is I1.
1. Understanding the Interference Condition:
- When three coherent light rays interfere at point P and produce a minimum of zero intensity, it implies that the rays are out of phase in such a way that they cancel each other out. This means that the resultant intensity from the three rays is zero.
2. Intensity of Individual Rays:
- Let the intensity of each individual ray be I0. Since the rays are coherent and of equal intensity, we can denote the intensity of each ray as I0.
3. Condition with Two Rays Blocked:
- When any two of the rays are blocked, the intensity at P is I1. This indicates that the intensity from the remaining ray is I1. Therefore, if one ray is left, it contributes its full intensity I0 to the point P.
4. Analyzing the Case with One Ray Blocked:
- Now, if only one ray is blocked, two rays remain. Since we know that the original combination of three rays results in zero intensity, the two remaining rays must also interfere destructively.
- The two remaining rays will have a phase difference that causes them to partially cancel each other out.
5. Calculating the Resultant Intensity:
- When two rays are present, the resultant intensity can be calculated using the formula for the resultant intensity of two coherent sources:
I=I1+I2+2√I1I2cos(ϕ)
- Here, I1 and I2 are the intensities of the two rays, and ϕ is the phase difference between them. Since both rays have equal intensity, we can set I1=I2=I0.
- Given that the resultant intensity when all three rays are present is zero, we can conclude that the phase difference between the two remaining rays must be such that they interfere destructively.
6. Final Result:
- When only one ray is blocked, the intensity at P will be 2I0 (from the two remaining rays), but since they cancel each other out partially, the resultant intensity will be I1 when one ray is blocked.
- Therefore, the intensity at P when only one ray is blocked is 2I1.
Conclusion:
The intensity of the light at point P, if only one of the rays is blocked, is 2I1.
- When three coherent light rays interfere at point P and produce a minimum of zero intensity, it implies that the rays are out of phase in such a way that they cancel each other out. This means that the resultant intensity from the three rays is zero.
- Let the intensity of each individual ray be I0. Since the rays are coherent and of equal intensity, we can denote the intensity of each ray as I0.
- When any two of the rays are blocked, the intensity at P is I1. This indicates that the intensity from the remaining ray is I1. Therefore, if one ray is left, it contributes its full intensity I0 to the point P.
- Now, if only one ray is blocked, two rays remain. Since we know that the original combination of three rays results in zero intensity, the two remaining rays must also interfere destructively.
- The two remaining rays will have a phase difference that causes them to partially cancel each other out.
- When two rays are present, the resultant intensity can be calculated using the formula for the resultant intensity of two coherent sources:
I=I1+I2+2√I1I2cos(ϕ)
- Here, I1 and I2 are the intensities of the two rays, and ϕ is the phase difference between them. Since both rays have equal intensity, we can set I1=I2=I0.
- Given that the resultant intensity when all three rays are present is zero, we can conclude that the phase difference between the two remaining rays must be such that they interfere destructively.
- When only one ray is blocked, the intensity at P will be 2I0 (from the two remaining rays), but since they cancel each other out partially, the resultant intensity will be I1 when one ray is blocked.
- Therefore, the intensity at P when only one ray is blocked is 2I1.
The intensity of the light at point P, if only one of the rays is blocked, is 2I1.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - INTERFERENCE AND DIFFRACTION EXERCISE - PRACTICE QUESTIONS (More than One Correct Choice Type)23videos
INTERFERENCE AND DIFFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - INTERFERENCE AND DIFFRACTION EXERCISE - PRACTICE QUESTIONS (Linked Comprehension)21videos
INTERFERENCE AND DIFFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - INTERFERENCE AND DIFFRACTION EXERCISE - PROBLEMS61videos
INTERFERENCE AND DIFFRACTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - HYDROGEN ATOMEXERCISE - PRACTICE QUESTIONS(Integer Type)6videos
HYDROGEN ATOM
- BOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FIELDS DUE TO CURRENTSEXERCISE - Practice Question (Integer Type)4videos
MAGNETIC FIELDS DUE TO CURRENTS
Similar Questions
The light waves from two coherent sources have same intensity I1=I2=I0. In interference pattern the intensity of light at minima is zero. What will be the intensity of light at maxima ?
View SolutionUnpolarised light of intensity I is incidnet of the polaroid. What will be the intensity of light transmitted by the polaroid?
View Solution
Knowledge Check
- Question 1 - Select One
In the interference pattern produced by two identical slits, the intensity of central maximum is l. What will the intensity of light at the same spot, if one of the slits is closed?
AlBl/2Cl/4Dl/8 - Question 2 - Select One
Two waves having the intensities in the ratio of 9:1 produce interference. The ratio of maximum to minimum intensity is equal to
A10:8B9:1C4:1D2:1 - Question 3 - Select One
A light source is located at P1 as shown in the figure. All sides of the polygon are equal. The intensity of illumination at P2 is I0 . What will be the intensity of illumination at P3
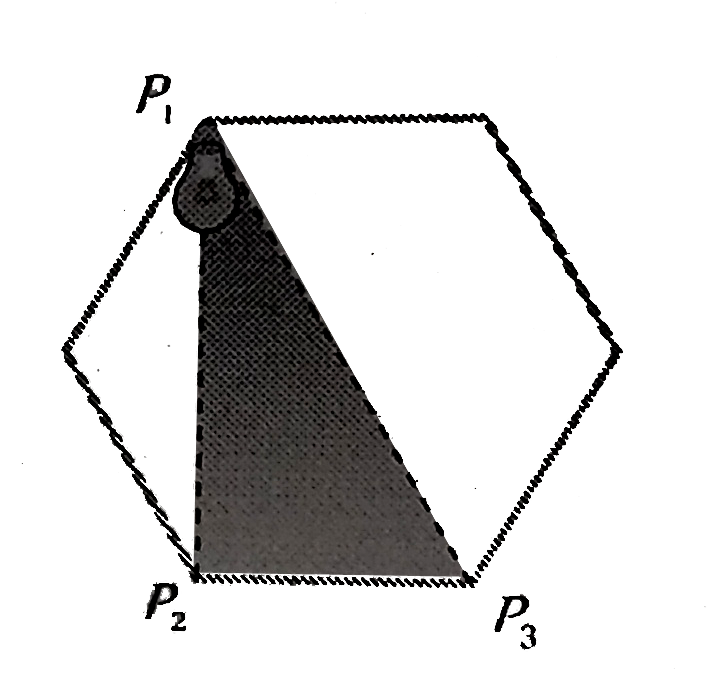 A3√28I0BI08C38I0D√38I0
A3√28I0BI08C38I0D√38I0
Unpolarised light of intensity I is passed through a polaroid. What is the intensity of emerging polaroised light ?
View SolutionThe intensity of scattered light I in Ray leigh scattering is proportional to ?
View SolutionInterference is produced with two coherent sources of same intensity. If one of the sources is covered with a thin film so as to reduce the intensity of light coming out of it to half, then
View SolutionTwo waves of same intensity produce interference. If intensity at maximum is 4I, then intensity at the minimum will be:
View SolutionTwo coherent light beams of intensities I and 4I produce interference pattern. The intensity at a point where the phase difference is zero, will b:
View Solution

