A straight wire carrying a current of 13 A is bent into a semi-circular arc of radius 2 cm as shown in figure. The magnetic field is 1.5×10−4T at the centre of arc, then the magnetic field due to straight segment is

We can find the magnetic field →B at point C by applying the Biot-Savart law of Eq. 29-3 to the wire, point by point along the full length of the wire. However, the application of Eq. 29-3 can be simplified by evaluating →B separately for the three distinguishable sections of the wire namely (1) the straight section at the left (2) the straight section at the right, and (3) the circular arc.
Straight sections : For any current-length element in section1, the angle θ between d→s and ˆr is zero (Fig. 29-14b) , so Eq. 29-1 gives as
dB1=μ04πsinθr2=μ04πsinθr2=0
Thus, the current along the entire length of straight section 1 contributes no magnetic field at C
B1=0
The same situation prevails in straight section 2, where the angle θ between d→s and ˆr for any current length element is 180∘. Thus,
B2=0
Circular arc : Application of the Biot-Savart law to evaluate the magnetic field at the center of a circular arc leads to Eq. 29-9 (B=μ0iϕ/4πR). Here the central angle ϕ of the arc is π/2 rad . Thus from Eq. 29-9, the magnitude of the magnetic field →B3 at the arc .s center C is
B3=μ0i(π/2)4πR=μ0i8R
To find the direction of →B3, we apply the right-hand rule displayed in Fig. 29-5. Mentally grasp the circular arc with your right hand as in Fig. 29-14c, with your thumb in the direction of the current. The direction in which your fingers curl around the wire indicates the direction of the magnetic field lines around the wire. They form circles around the wire, coming out of the page above the arc and going into the page inside the arc. In the region
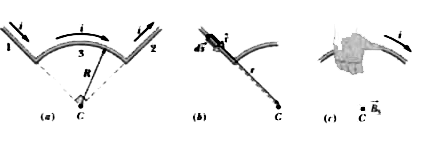
of point C (inside the arc), your fingertips point into the plane of the page. Thus , →B3 is directed into that plane.
Net field : Generally we combine multiple magnetic fields as vectors. Here, however, only the circular arc produces a magnetic field at point C. Thus , we can write the magnitude of the net field →B as
B=B1+B2+B3=0+0+μ0i8R=μ0i8R
The direction of →B is the direction of →B3 namely into the plabe of Fig 29-14
Topper's Solved these Questions
- MAGNETIC FIELDS DUE TO CURRENTSBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FIELDS DUE TO CURRENTSEXERCISE - Check point
4 Videos
- MAGNETIC FIELDS DUE TO CURRENTSBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FIELDS DUE TO CURRENTSEXERCISE - Problems
45 Videos
- INTERFERENCE AND DIFFRACTION BOOK - RESNICK AND HALLIDAYCHAPTER - INTERFERENCE AND DIFFRACTION EXERCISE - PRACTICE QUESTIONS (Integer Type)
6 Videos
- MAGNETIC FORCEBOOK - RESNICK AND HALLIDAYCHAPTER - MAGNETIC FORCEEXERCISE - Practice Questions (Integer type )
7 Videos
Similar Questions
A straight wire carrying a current of 12A is bent into a semicircular arc of radius 2⋅0cm as shown in figure. Consider the magnetic field →B at the centre of arc.
(a) What is the magnetic field due to the staight segments?
(b) In what way the contribution to →B from the semicircle differs from that of a circular loop and in what way does it resemble?
(c) Would your answer be different if the wire were bent into a semicircle arc of the same radius but in the opposite way as shown in figure
View SolutionA straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View Solution
Knowledge Check
A straight wire carrying a current 10A is bent into a semicircular arc of radius 5cm. The magnitude of magnetic field at the center is
A1.5×10−5TB3.14×10−5TC6.28×10−5TD19.6×10−5TA part of a long wire carrying a current i is bent into a circle of radius r as shown in figure. The net magnetic field at the centre O of the circular loop is
 Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)
Aμ0i4rBμ0i2rCμ0i2πr(π+1)Dμ0i2πr(π−1)A long wire carrying current 'I' is bent into the shape as shown in the figure. The net magnetic field intensity at the centre 'O' is
 Aμ0I4πR√1+π2Bμ0I4πR√2+π2Cμ0I4πR√4+π2Dμ0I4πR√9+π2
Aμ0I4πR√1+π2Bμ0I4πR√2+π2Cμ0I4πR√4+π2Dμ0I4πR√9+π2
A straight wire carrying a current of 12 A is bent into a semi-circular are of radius of2.0 cm as shown in the adjoining figure (a). Consider the magnetic field B at the centre of the arc. Would your answer be different if the wire were bent into a semi-circular arc of the same radius but in the opposite way a shown in figure (b)?

View SolutionA thick straight copper wire, carrying a current of 10 A is bent into a semicircular arc of radius 7.0 cm as a shown in Fig (a). (i) State the direction and calculate the magnitude of magnetic field at the centre of arc. (ii) How would your answer change if the same wire were bent into a semicircular arc of the same radius but in opposite way as shown in Fig. (b)?

View Solution(a) Derive the expression for the magnetic field due to a current carrying coil of radius r at a distance x from the centre along the X-axis.
(b) A straight wire carrying a current of 5 A is bent into a semicular arc of radius 2 cm as shown in the fig. FInd th magnitude and direction of the magnetic field at the centre of the arc.

View SolutionA piece of wire carrying a current of 6.00 A is bent in the form of a circular arc of radius 10.0 cm, and it subtends an angle of 120∘ at the centre. Find the magnetic field B due to this piece of wire at the centre.
View SolutionA long straight conductor carrying a current i is bent to form an almost complete circular loop of radius r on it. The magnetic field at the centre of the loop
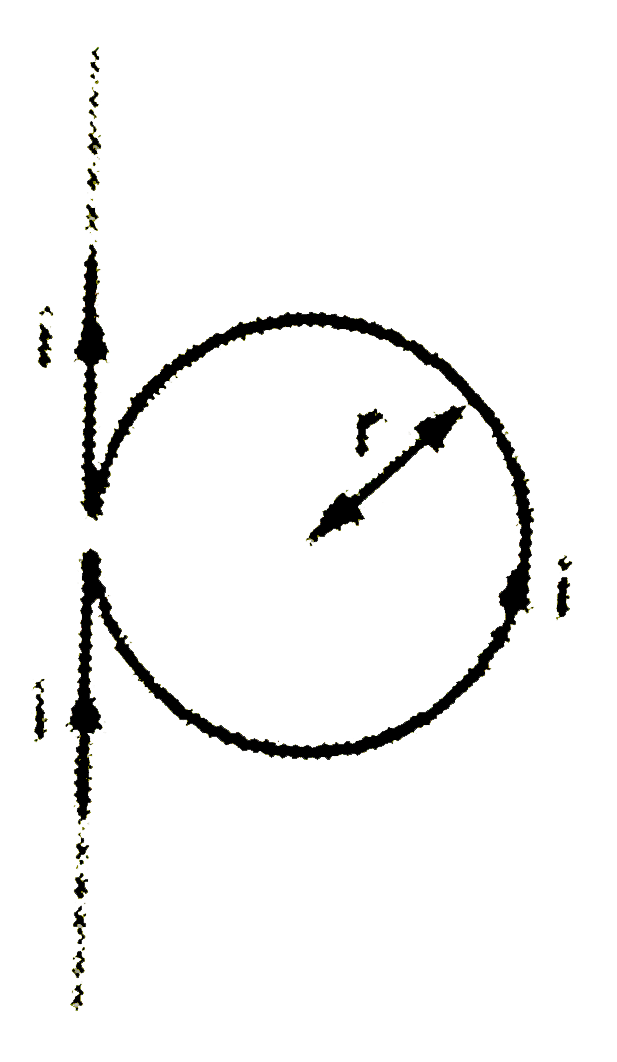
View Solution
