A piece of wire carrying a current of 6.00 A is bent in the form of a circular arc of radius 10.0 cm, and it subtends an angle of 120∘ at the centre. Find the magnetic field B due to this piece of wire at the centre.
To find the magnetic field B due to a piece of wire bent in the form of a circular arc at the center, we can follow these steps:
Step 1: Understand the Problem
We have a wire carrying a current I=6.00A bent into a circular arc with a radius r=10.0cm (which is 0.1m) and it subtends an angle of 120∘ at the center.
Step 2: Convert the Angle to Radians
To use the formula for the magnetic field, we need to convert the angle from degrees to radians. The conversion formula is:
radians=degrees×π180
For 120∘:
θ=120×π180=2π3radians
Step 3: Use the Formula for Magnetic Field
The magnetic field B at the center of a circular arc is given by the formula:
B=μ0Iθ4πr
where:
- μ0=4π×10−7T m/A (permeability of free space)
- I is the current in amperes
- r is the radius in meters
- θ is the angle in radians
Step 4: Substitute the Values into the Formula
Substituting the known values into the formula:
B=(4π×10−7)×6×2π34π×0.1
Step 5: Simplify the Expression
We can simplify the expression:
B=(4π×10−7)×6×2π34π×0.1
The 4π cancels out:
B=(10−7)×6×2π30.1
B=(10−7)×6×2π310−1
B=(10−6)×6×2π3
Step 6: Calculate the Final Value
Calculating the numerical value:
B=10−6×6×2π3
B=10−6×4π
Using π≈3.14:
B≈10−6×4×3.14≈12.56×10−6T
Thus,
B≈1.256×10−5T
Final Answer
The magnetic field B at the center due to the piece of wire is approximately:
B≈1.256×10−5T(Tesla)
---
We have a wire carrying a current I=6.00A bent into a circular arc with a radius r=10.0cm (which is 0.1m) and it subtends an angle of 120∘ at the center.
To use the formula for the magnetic field, we need to convert the angle from degrees to radians. The conversion formula is:
radians=degrees×π180
For 120∘:
θ=120×π180=2π3radians
The magnetic field B at the center of a circular arc is given by the formula:
B=μ0Iθ4πr
where:
- μ0=4π×10−7T m/A (permeability of free space)
- I is the current in amperes
- r is the radius in meters
- θ is the angle in radians
Substituting the known values into the formula:
B=(4π×10−7)×6×2π34π×0.1
We can simplify the expression:
B=(4π×10−7)×6×2π34π×0.1
The 4π cancels out:
B=(10−7)×6×2π30.1
B=(10−7)×6×2π310−1
B=(10−6)×6×2π3
Calculating the numerical value:
B=10−6×6×2π3
B=10−6×4π
Using π≈3.14:
B≈10−6×4×3.14≈12.56×10−6T
Thus,
B≈1.256×10−5T
The magnetic field B at the center due to the piece of wire is approximately:
B≈1.256×10−5T(Tesla)
Topper's Solved these Questions
- MAGNETIC FIELD DUE TO CURRENTBOOK - HC VERMACHAPTER - MAGNETIC FIELD DUE TO CURRENTEXERCISE - Objective 2
7 Videos
- MAGNETIC FIELDBOOK - HC VERMACHAPTER - MAGNETIC FIELDEXERCISE - Exercises
61 Videos
- MAGNETIC PROPERTIES OF MATTERBOOK - HC VERMACHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Exercises
9 Videos
Similar Questions
Knowledge Check
A straight wire carrying a current 10A is bent into a semicircular arc of radius 5cm. The magnitude of magnetic field at the center is
A1.5×10−5TB3.14×10−5TC6.28×10−5TD19.6×10−5T
Find the length of an arc of a circle of radius 10cm subtending an angle of 300 at the centre
View SolutionIn a circle of radius 28 cm, an arc subtends an angle of 108∘ at the centre .
(a) Find the area of the sector.
(b) Find the length of the arc.
View SolutionA straight wire carrying a current of 13 A is bent into a semi-circular arc of radius 2 cm as shown in figure. The magnetic field is 1.5×10−4T at the centre of arc, then the magnetic field due to straight segment is

View SolutionA straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View SolutionA straight wire carrying a current of 12A is bent into a semicircular arc of radius 2⋅0cm as shown in figure. Consider the magnetic field →B at the centre of arc.
(a) What is the magnetic field due to the staight segments?
(b) In what way the contribution to →B from the semicircle differs from that of a circular loop and in what way does it resemble?
(c) Would your answer be different if the wire were bent into a semicircle arc of the same radius but in the opposite way as shown in figure
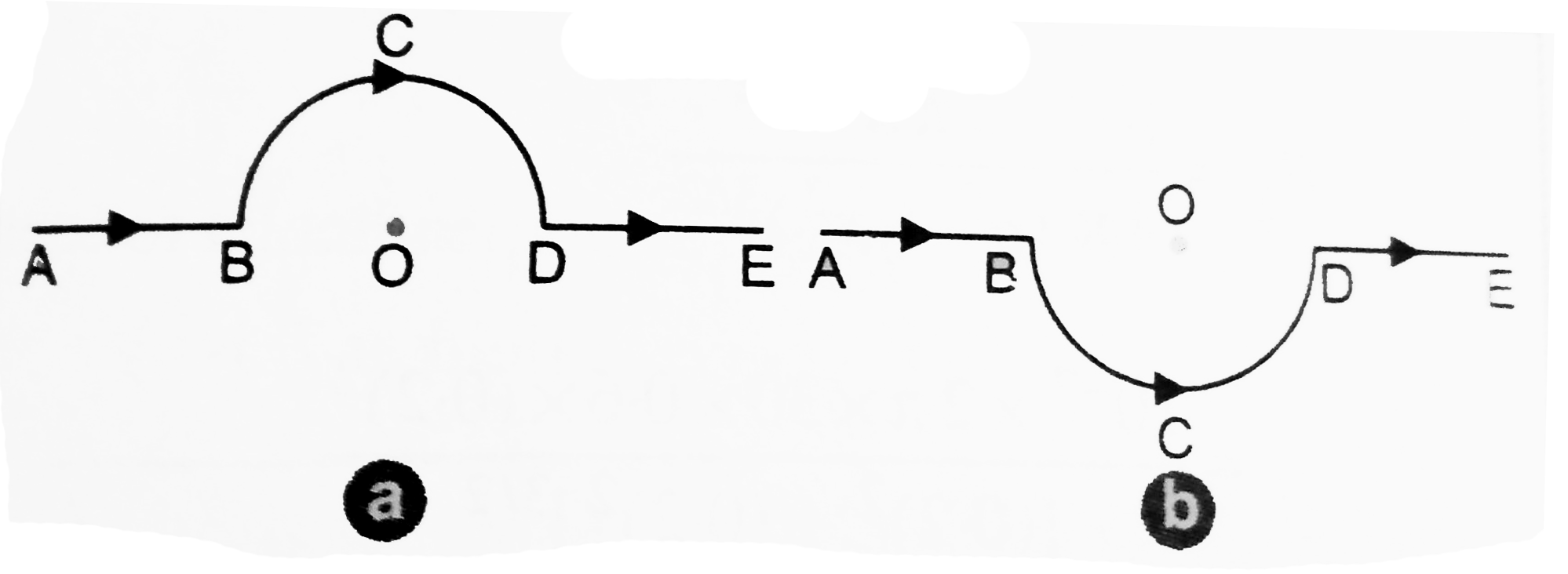
View SolutionA piece of wire 11 cm long is bent into the form of an arc of a circle subtending an angle of 45∘ at its centre. Find the radius of the circle.
View SolutionA piece of wire 20 cm long is bent into the from of an arc of a circle, subtending an angle of 60∘ at its centre. Find the radius of the circle.
View Solution
