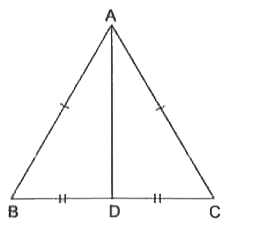
In the adjoining figure,AB=AC and BD=DC Prove that ΔADB≡ΔADC and hence show that (i) ∠ADB≡∠ADC=90∘ , (ii) ∠BAD=∠CAD
Step by step video & image solution for In the adjoining figure,AB=AC and BD=DC Prove that Delta ADB-=Delta ADC and hence show that (i) /_ADB-=/_ADC=90^(@) , (ii) /_BAD=/_CAD by Maths experts to help you in doubts & scoring excellent marks in Class 7 exams.
Updated on:21/07/2023Similar Questions
In the adjoining figure, AB = AC and BD = DC. Prove that △ADB=△ADC and hence show that
(i) ∠ADB=∠ADC=90∘, (ii) ∠BAD=∠CAD.
View Solutionin the adjoining figure, AB=AC and AD=AE. Prove that 1. ∠ADB=∠AEC 2. ΔABD≅ΔACE 3. BE=DC
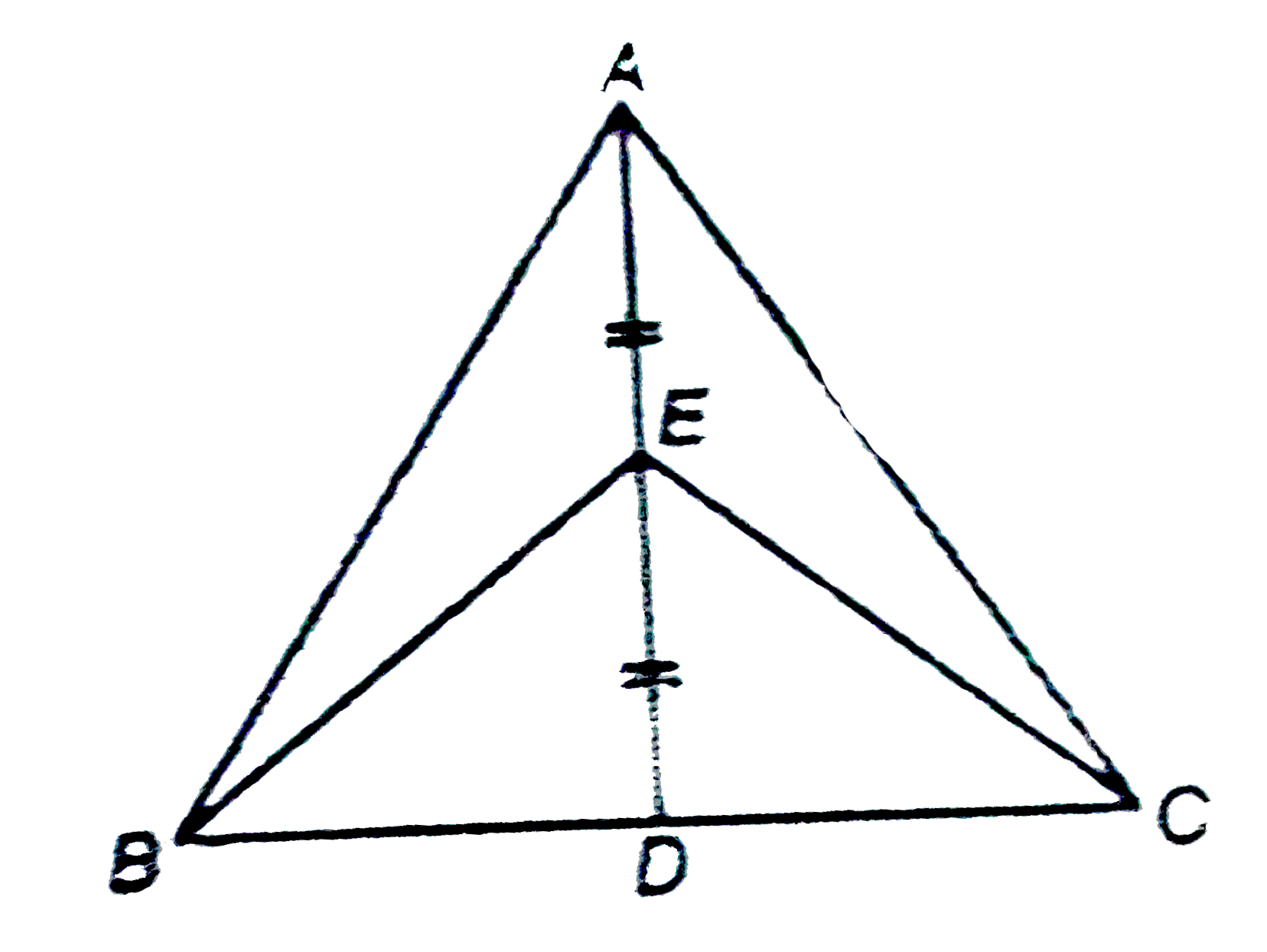
View SolutionIn the adjoining figure, BD = DC and AE = ED. Prove that
area of ΔACE=14 area of ΔABC
View SolutionIn Figure, it is given that AE=AD and BD=CE. Prove that AEB ≅ADC
View SolutionIn the given figure , AC>AB and AD bisects ∠BAC Prove that ∠ADC>ADB
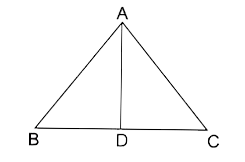
View SolutionIn the adjoining figure, ABC is a triangle and D is any point in its interior. Show that (BD+DC)<(AB+AC).
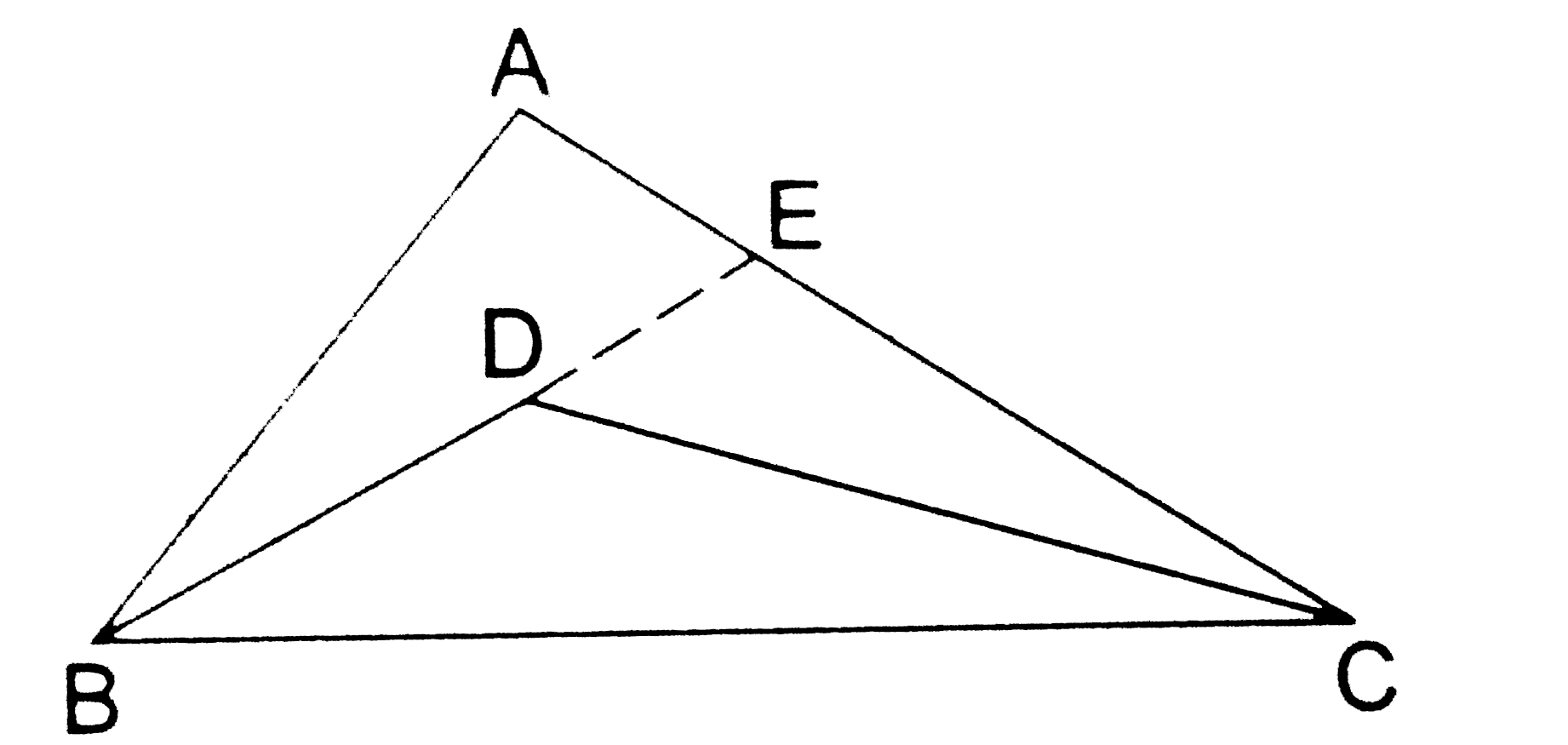
View SolutionIn the adjoining figure, ABC is a triangle and D is any point in its interior. Show that BD+DC<AB+AC.
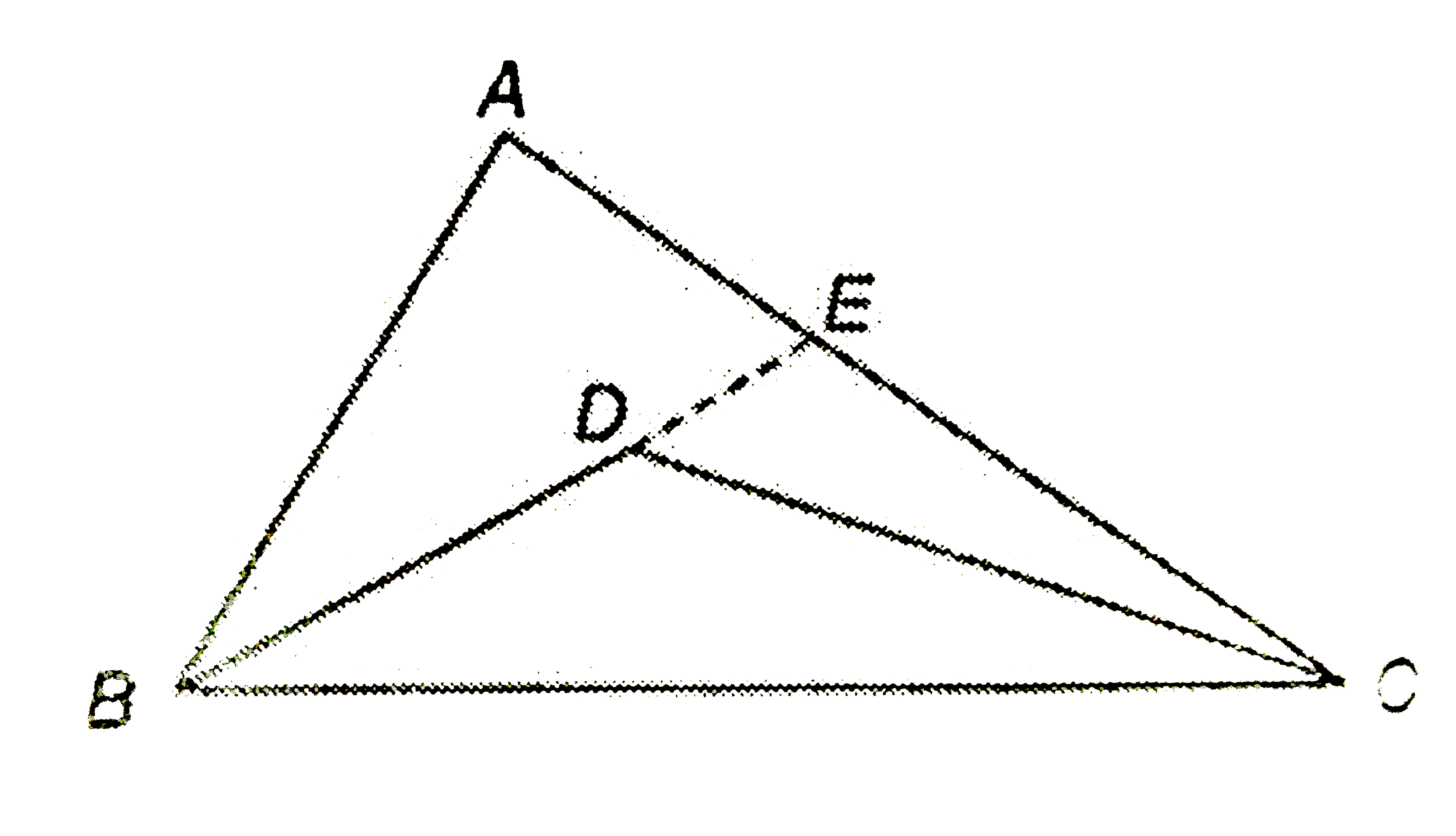
View SolutionIn the adjoining figure, ABC is a triangle and D is any point in its interior. Show that BD+DC<AB+AC.

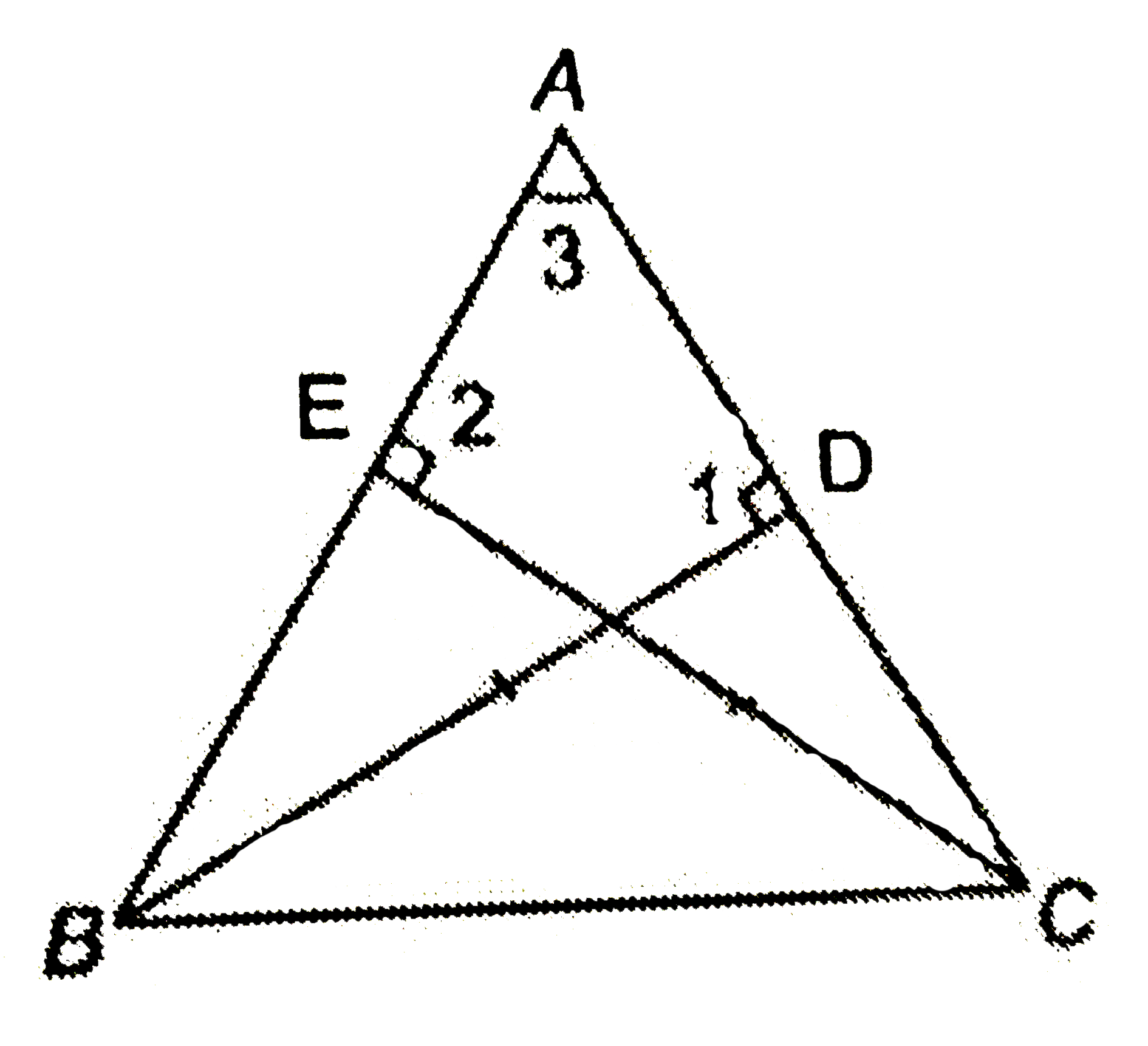
View SolutionIn the adjoining figure, BD = CE and ∠ADB=∠AEC=90∘, prove that
(i) ΔABD≅ΔACE (ii) ABC is an isosceles triangle.
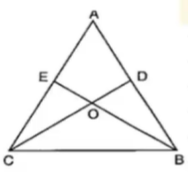
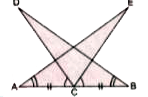
View SolutionUse the information given in the adjoining figure, to prove :
(i) ΔDBC≅ΔEAC.
(ii) DC = EC.
View Solution
