In ΔABC,∠ABC=70∘,∠BCA=40∘. O is the point of intersection of the perpendicular bisectors of the sides, then the angle ∠BOC is
100∘
120∘
130∘
140∘
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Identify the angles in triangle ABC
We know that:
- Angle ABC = 70°
- Angle BCA = 40°
Step 2: Calculate angle A
Using the property that the sum of angles in a triangle is 180°, we can find angle A:
Angle A=180°−Angle ABC−Angle BCA
Substituting the known values:
Angle A=180°−70°−40°=70°
Step 3: Identify the circumcenter O
Since O is the point of intersection of the perpendicular bisectors of the sides of triangle ABC, it is the circumcenter of the triangle.
Step 4: Use the property of angles subtended by an arc
We know that the angle subtended by an arc at the center (angle BOC) is twice the angle subtended at the circumference (angle BAC). Therefore:
Angle BOC=2×Angle A
Substituting the value of angle A:
Angle BOC=2×70°=140°
Step 5: Conclusion
Thus, the angle BOC is:
Angle BOC=140°
Final Answer
The angle ∠BOC is 140°.
---
We know that:
- Angle ABC = 70°
- Angle BCA = 40°
Using the property that the sum of angles in a triangle is 180°, we can find angle A:
Angle A=180°−Angle ABC−Angle BCA
Substituting the known values:
Angle A=180°−70°−40°=70°
Since O is the point of intersection of the perpendicular bisectors of the sides of triangle ABC, it is the circumcenter of the triangle.
We know that the angle subtended by an arc at the center (angle BOC) is twice the angle subtended at the circumference (angle BAC). Therefore:
Angle BOC=2×Angle A
Substituting the value of angle A:
Angle BOC=2×70°=140°
Thus, the angle BOC is:
Angle BOC=140°
The angle ∠BOC is 140°.
Similar Questions
Knowledge Check
The point of intersection of the angle bisectors of a triangle is :
AorthocentreBcentroidCincentreDcircumcentreThe point of intersection of the angle bisectors of a triangle is :
AorthocentreBcentroidCincentreDcircumcenterIn the adjoning figure of ΔABC,AD is the perpendicular bisector of side BC. The triangle ABC is :
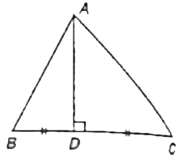 Aright angledBisoscelesCscaleneDequilateral
Aright angledBisoscelesCscaleneDequilateral
In a △ABC,∠C=120∘ If he be the harmonic mean of the length of the sides BC and CA, then the length of the bisector of ∠BCA is
View SolutionThe point at which the perpendicular bisectors of the sides of a triangle intersect is known as ________.
View SolutionIn ΔABC,∠B=70∘and∠C=30∘, AD and AE are respectively the perpendicular on side BC and bisector of ∠A. The measure of ∠DAE is:
View SolutionThe point at which the perpendicular bisectors of the sides of a triangle intersect is known as ________.
View SolutionIn ΔABC,∠C=54∘, the perpendicular bisector of AB at D meets BC at E. If ∠EAC=42∘, then what is the value (in degrees) of ∠ABC?
View Solution