In the arrangement shown in figure y=1.0 nm, d=0.24 mm and D=1.2m. The work function of the material of the emitter is 2.2 eV. If stopping potential is 0.3 x, then value of x (in V) is

If λ is the wavelength emitted by the source S, then fringe width
β=1y=Dλd
∴λ=2ydD
=2×(1×10−3)×(0.24×10−3)1.2
⇒4×10−6m
By Einstein equation
hf=W0+eV
or hcλ=W0+eV
∴V=hceλ−W0e
=(6.63×10)−34×(3×108)(1.6×10−19)×(0.4×10−6)−2.2×1.6×10−191.6×10−19
=0.9V
Topper's Solved these Questions
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 26 : (ATOMS)
15 Videos
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 27 : (NUCLEI)
15 Videos
- CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSBOOK - DISHA PUBLICATIONCHAPTER - CHAPTERWISE NUMERIC /INTEGER ANSWER QUESTIONSEXERCISE - CHAPTER 24 : (WAVE OPTICS)
15 Videos
- ATOMSBOOK - DISHA PUBLICATIONCHAPTER - ATOMSEXERCISE - EXERCISE-2: CONCEPT APPLICATOR
30 Videos
- COMMUNICATION SYSTEM BOOK - DISHA PUBLICATIONCHAPTER - COMMUNICATION SYSTEM EXERCISE - EXERCISE-2 : Concept Applicator
30 Videos
Similar Questions
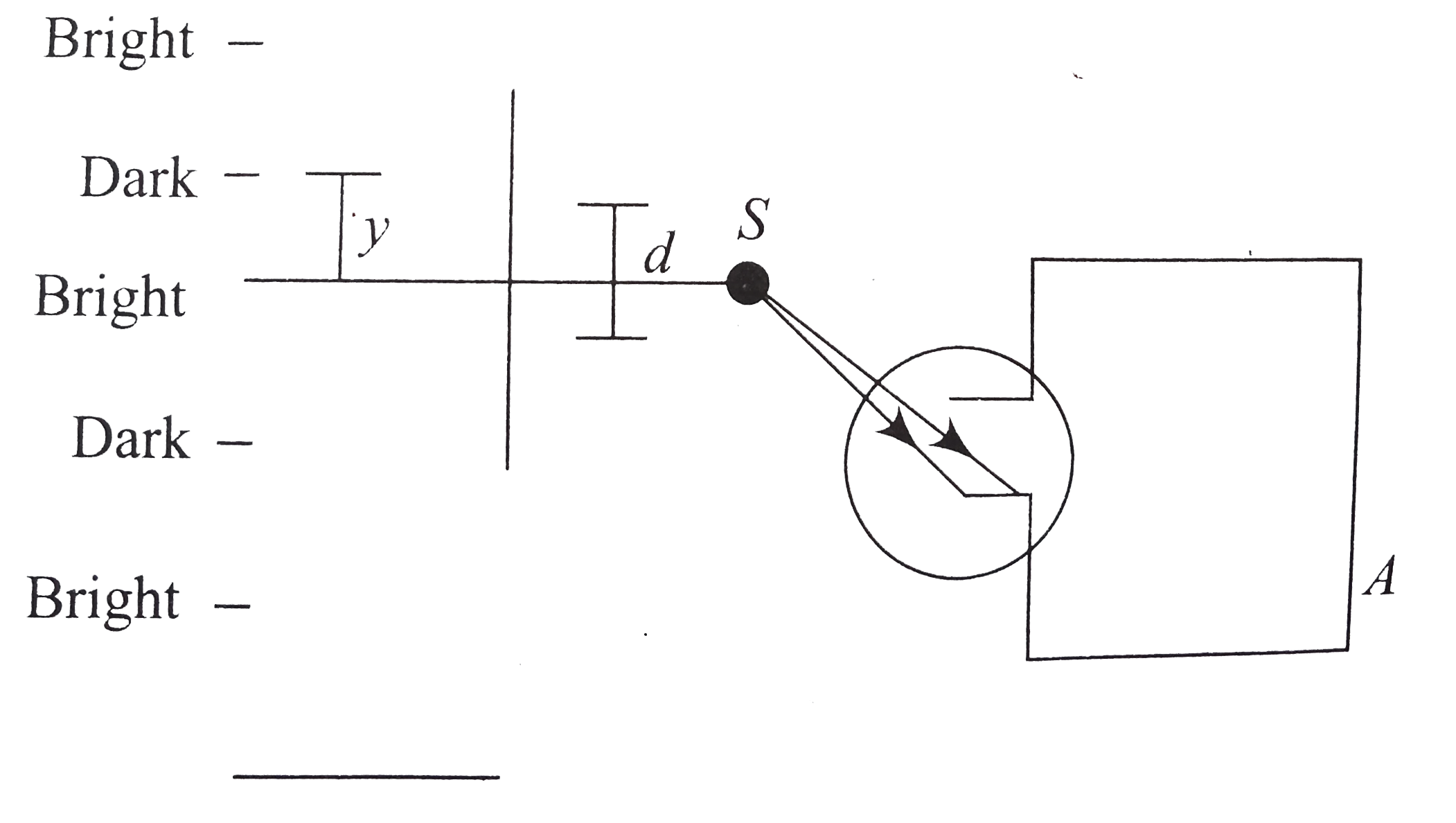
In the arrangement shown if Fig. y=1.0mm,d=0.24mm and D=1.2m. The work function of the material of the emitter if 2.2 eV. Find the stopping potential V needed to stop photocurrent (in×10−1V).
View SolutionIn the following arrangement y=1.0mm,d=0.24mm and D=1.2m. The work function of the material of the emitter is 2.2eV. The stopping potential V needed to stop the photo current will be

View Solution
Knowledge Check
In the following arrangement y=1.0mm,d=0.24mm and D=1.2m. The work function of the material of the emitter is 2.2eV. The stopping potential V needed to stop the photo current will be
 A0.9VB0.5VC0.4VD0.1V
A0.9VB0.5VC0.4VD0.1VThe work function of Cs is 2.14 ev find the wavelength of the incident light if the stopping potential is 0.6 V.
A326 nmB454 nmC524 nmD232 nmThe radiation of wavelength 332 nm is incident on a metal of work function 1.70 eV. The value of the stopping potential will be
A0.70 eVB1.14 eVC1.68 eVD2.06 eV
Light of energy 2.0 eV falls on a metal of work function 1.4 eV. The stopping potential is :
View SolutionLight of energy 2.0 eV falls on a metal of work function 1.4 eV. The stopping potential is :
View SolutionLight of energy 2.0 eV falls on a metal of work function 1.4 eV . The stopping potential is
View SolutionLight of energy 2.0 eV falls on a metal of work function 1.4 eV . The stopping potential is
View SolutionA graph of the x-component of the electric field as a function of x in a region of space is shown in figure. The y−andz−compo≠nts of the electric field are zero in this region. If the electric potential is 10 V at the origin, then the potential at x = 2.0 m is

View Solution
