Find the area of △ABC, the midpoints of whose sides AB, BC and CA are D(3,-1), E(5, 3) and F(1,-3) respectively.
The correct Answer is:8 sq units
To find the area of triangle ABC given the midpoints D, E, and F of its sides, we can follow these steps:
Step 1: Identify the coordinates of the midpoints
We are given the midpoints of the sides of triangle ABC:
- D(3, -1) is the midpoint of side AB
- E(5, 3) is the midpoint of side BC
- F(1, -3) is the midpoint of side CA
Step 2: Use the formula for the area of a triangle
The area of a triangle formed by three points (x1, y1), (x2, y2), and (x3, y3) can be calculated using the formula:
Area=12|x1(y2−y3)+x2(y3−y1)+x3(y1−y2)|
Step 3: Substitute the coordinates of points D, E, and F into the formula
Let:
- D(3,−1)⇒(x1,y1)=(3,−1)
- E(5,3)⇒(x2,y2)=(5,3)
- F(1,−3)⇒(x3,y3)=(1,−3)
Substituting these values into the area formula:
Area=12|3(3−(−3))+5((−3)−(−1))+1((−1)−3)|
Step 4: Simplify the expression
Calculating each term:
- For the first term: 3(3+3)=3×6=18
- For the second term: 5(−3+1)=5×(−2)=−10
- For the third term: 1(−1−3)=1×(−4)=−4
Now, combine these results:
Area=12|18−10−4|=12|18−14|=12|4|=42=2
Step 5: Calculate the area of triangle ABC
The area of triangle ABC is four times the area of triangle DEF (since D, E, and F are midpoints):
Area of △ABC=4×Area of △DEF=4×2=8 square units
Final Answer
The area of triangle ABC is 8 square units.
---
We are given the midpoints of the sides of triangle ABC:
- D(3, -1) is the midpoint of side AB
- E(5, 3) is the midpoint of side BC
- F(1, -3) is the midpoint of side CA
The area of a triangle formed by three points (x1, y1), (x2, y2), and (x3, y3) can be calculated using the formula:
Area=12|x1(y2−y3)+x2(y3−y1)+x3(y1−y2)|
Let:
- D(3,−1)⇒(x1,y1)=(3,−1)
- E(5,3)⇒(x2,y2)=(5,3)
- F(1,−3)⇒(x3,y3)=(1,−3)
Area=12|3(3−(−3))+5((−3)−(−1))+1((−1)−3)|
Calculating each term:
- For the first term: 3(3+3)=3×6=18
- For the second term: 5(−3+1)=5×(−2)=−10
- For the third term: 1(−1−3)=1×(−4)=−4
Area=12|18−10−4|=12|18−14|=12|4|=42=2
The area of triangle ABC is four times the area of triangle DEF (since D, E, and F are midpoints):
Area of △ABC=4×Area of △DEF=4×2=8 square units
The area of triangle ABC is 8 square units.
Topper's Solved these Questions
- STRAIGHT LINES BOOK - RS AGGARWALCHAPTER - STRAIGHT LINES EXERCISE - EXERCISE-20B
25 Videos
- STRAIGHT LINES BOOK - RS AGGARWALCHAPTER - STRAIGHT LINES EXERCISE - EXERCISE 20C
24 Videos
- STRAIGHT LINES BOOK - RS AGGARWALCHAPTER - STRAIGHT LINES EXERCISE - MISCELLANEOUS PROBLEMS
9 Videos
- STATISTICSBOOK - RS AGGARWALCHAPTER - STATISTICSEXERCISE - EXERCISE 30D
5 Videos
- THREE-DIMENSIONAL GEOMETRY BOOK - RS AGGARWALCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - EXERCISE 26 C
13 Videos
Similar Questions
The coordinates of the mid points of sides AB, BC and CA of ABC are D(1,2,−3), E(3,0,1)and F(−1,1,−4) respectively. Write the coordinates of its centroid.
View SolutionThe midpoints of the sides BC, CA and AB of a △ABC are D(2,1), E(-5,7)and F-5, -5) respectively. Find the equations of the sides of △ABC.
View Solution
Knowledge Check
IF the co ordinates of the mid points D,E,F of the sides BC,CA,AB of a triangle ABC are (-1,2),(3,5) and (2,3) respectively, the equation of BC is
A2x−y+4=0B2x−y−4=0C2x−y=0D2x+y−4=0if an equilateral triangle ABC with vertices at z1,z2andz3 be inscribed in the circle |z|=2 and again a circle is inscribed in the triangle ABC touching the sides AB, BC and CA at D(z4),E(z5)andF(z6) respectively
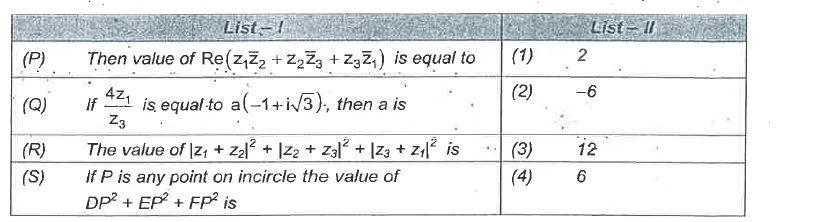 APQRS4123BPQRS3412CPQRS1324DPQRS2134
APQRS4123BPQRS3412CPQRS1324DPQRS2134In a triangle ABC, D, E and F are the mid-points of the sides BC, CA and AB, respectively. BE and DF intersect at X. DE and CF intersect at Y. Find XY=?
A)12BC
B)14BC
C)13BC
D)23BCA12BCB14BCC13BCD23BC
The midpoints of the sides BC, CA and AB of a ΔABC are D(3, 4), E(8, 9) and F(6, 7) respectively. Find the coordinates of the vertices of the triangle.
View SolutionThe incircle of an isoceles triangle ABC, with AB=AC, touches the sides AB,BC and CA at D,E and F respecrively. Prove that E bisects BC.
View SolutionThe incircle of an isosceles triangle ABC, in which AB = AC, touches the sides BC, CA and AB at D, E and F respectively . Prove that BD = DC.

View SolutionThe incircle of an isosceles triangle ABC, with AB=AC, touches the sides AB, BC, CA at D,E and F respectively. Prove that E bisects BC.
View SolutionIn Fig. ABC is a triangle co-ordinates of whose vertex A are (0,−1). D and E respectively are the mid-points of sides AB and AC their co-ordinates are (1,0) and (0,1) respectively. If F is the mid-point of BC find the areas of △ABC and △DEF
View Solution
