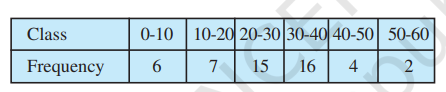
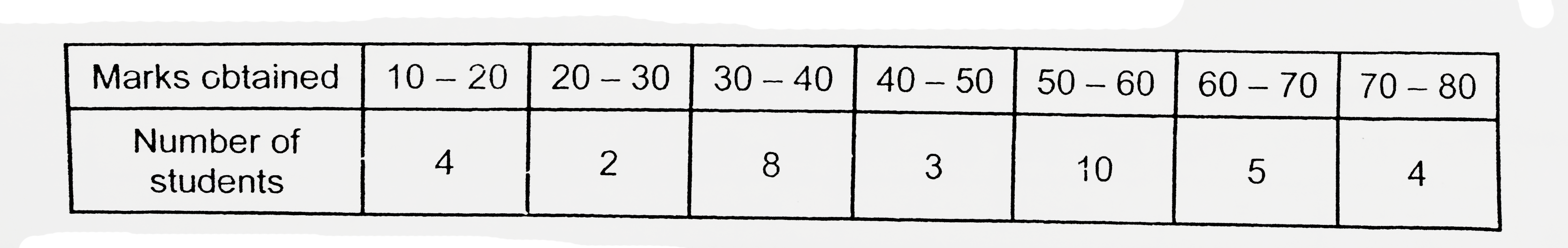
Calculate the mean deviation about the median for the following data :

Video Solution
Text Solution
Converting the given series into an exclusive series, we prepare the table, given below :
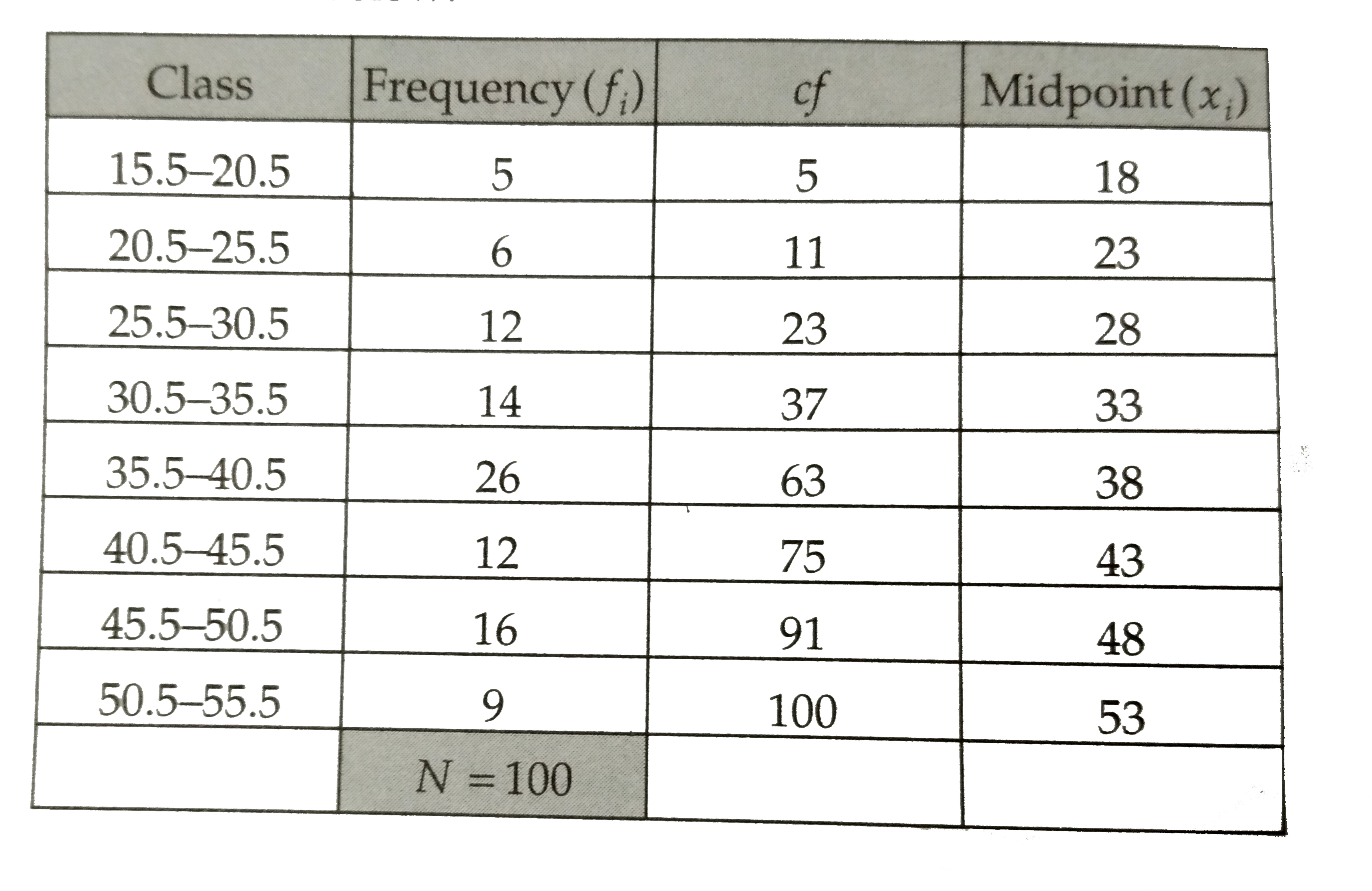
Thus N=100 and therefore, N2=50
⇒medians35.5−40.5
⇒ L=35.5,f=26,h=5andc=37.
∴ median=L+(N2−c)f×h
={35.5+(50−37)26×5}=(35.5+2.5)=38.
Thus, M=38.
Now, we prepare the table given below :
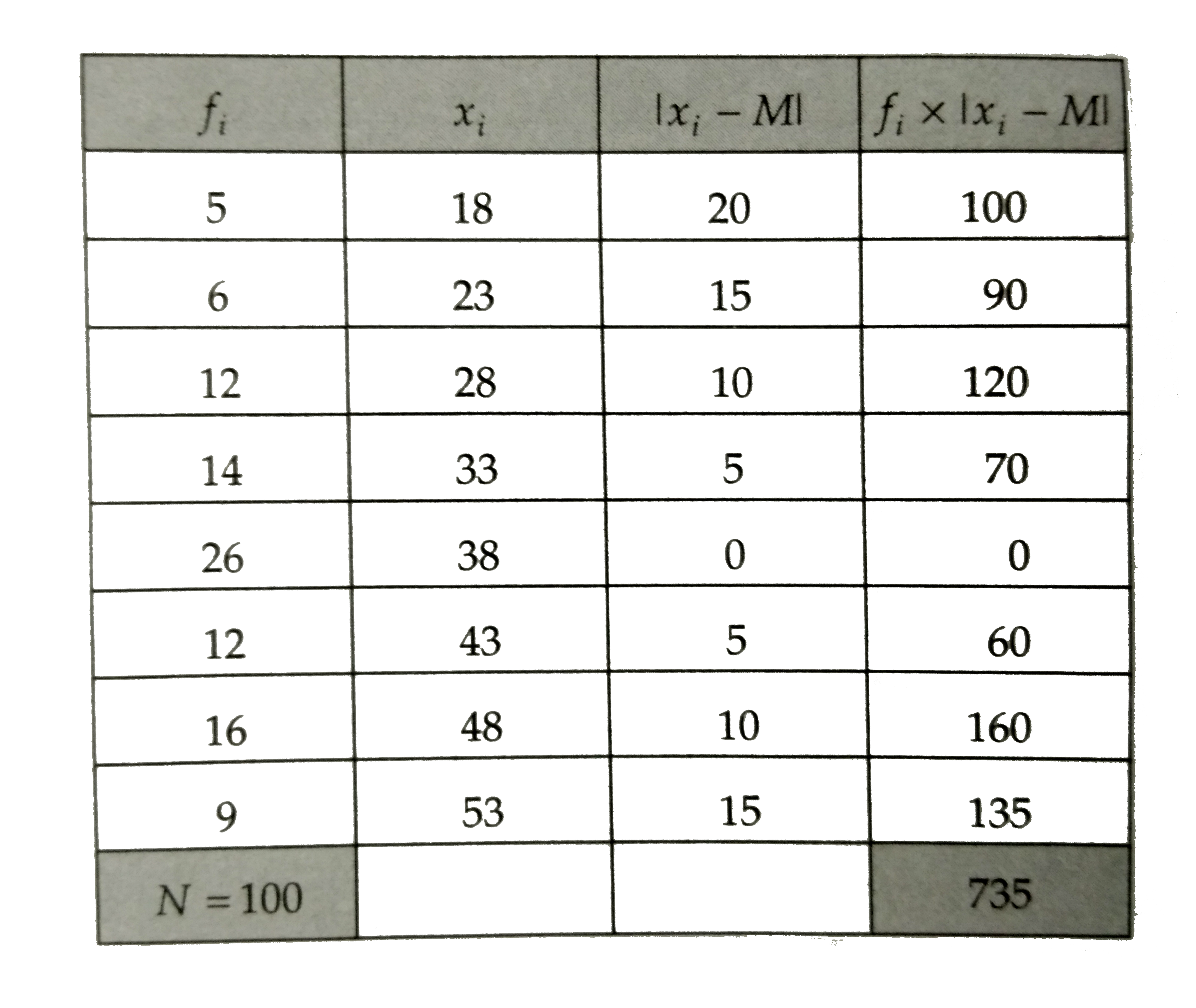
Thus, Σfi×|xi−M|=735andN=100.
∴ MD(M)=Σfi×|xi−M|N=735100=7.35.
Hence, the mean deviation about the median is 7.35.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - STATISTICSEXERCISE - Miscellaneous word problems (EXAMPLE)6videos
STATISTICS
- BOOK - RS AGGARWALCHAPTER - STATISTICSEXERCISE - EXERCISE 30A18videos
STATISTICS
- BOOK - RS AGGARWALCHAPTER - SOME SPECIAL SERIESEXERCISE - EXERCISE 13B (Very short Answer Questions)9videos
SOME SPECIAL SERIES
- BOOK - RS AGGARWALCHAPTER - STRAIGHT LINES EXERCISE - EXERCISE 20K10videos
STRAIGHT LINES
Similar Questions
Knowledge Check
- Question 1 - Select One
Find the mean deviation about median for the following data.
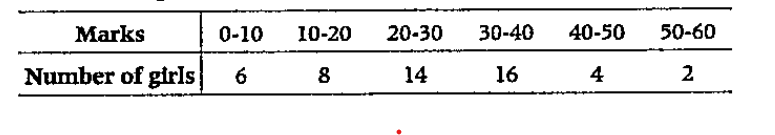 A10.34B10.50C10.40D10.37
A10.34B10.50C10.40D10.37
Calculate the mean deviation about median for the following data:
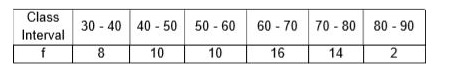 View Solution
View SolutionCalculate the mean deviation about median for the following data :
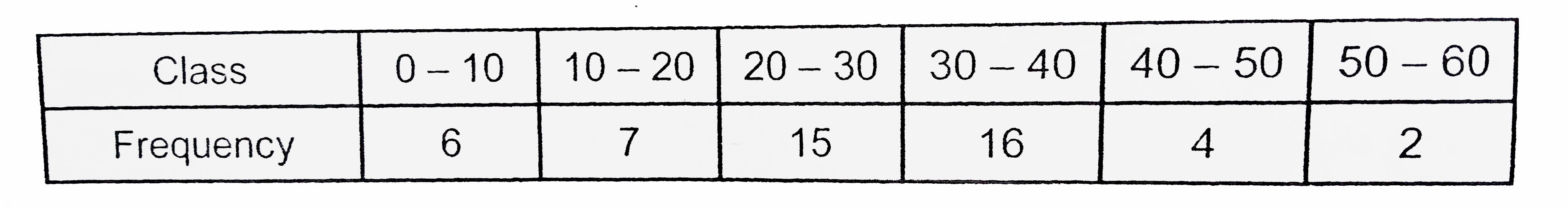 View Solution
View SolutionFind the mean deviation about the median for the following data:
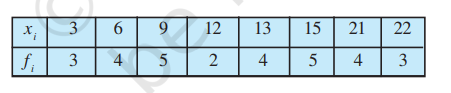 View Solution
View SolutionFind the mean deviation about the mean for the following data :
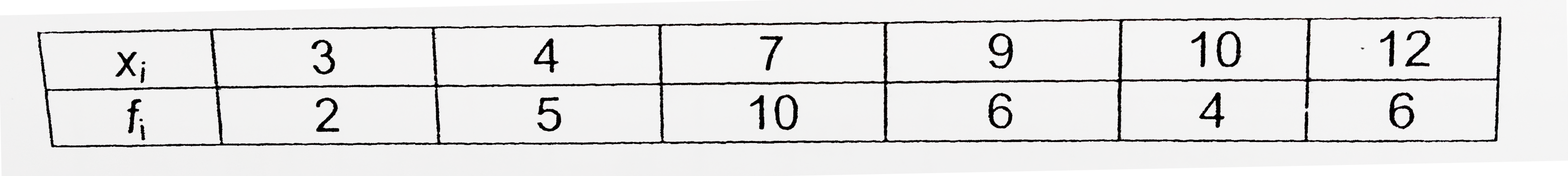 View Solution
View SolutionFind the mean deviation about the mean for the following data :
 View Solution
View SolutionCalculate the mean deviation about median from the following data: 340, 150, 210, 240, 300, 310, 320.
View SolutionCalculate the mean deviation about median from the following data: 340, 150, 210, 240, 300, 310, 320.
View Solution