Question

Construct a $\Delta ABC$ in which $BC=6$ cm, $\angle B={60}^{\circ }$ and $(AB+AC)=9$ cm. Measure $AB$ and $AC$. Justify your answer.

Explanation

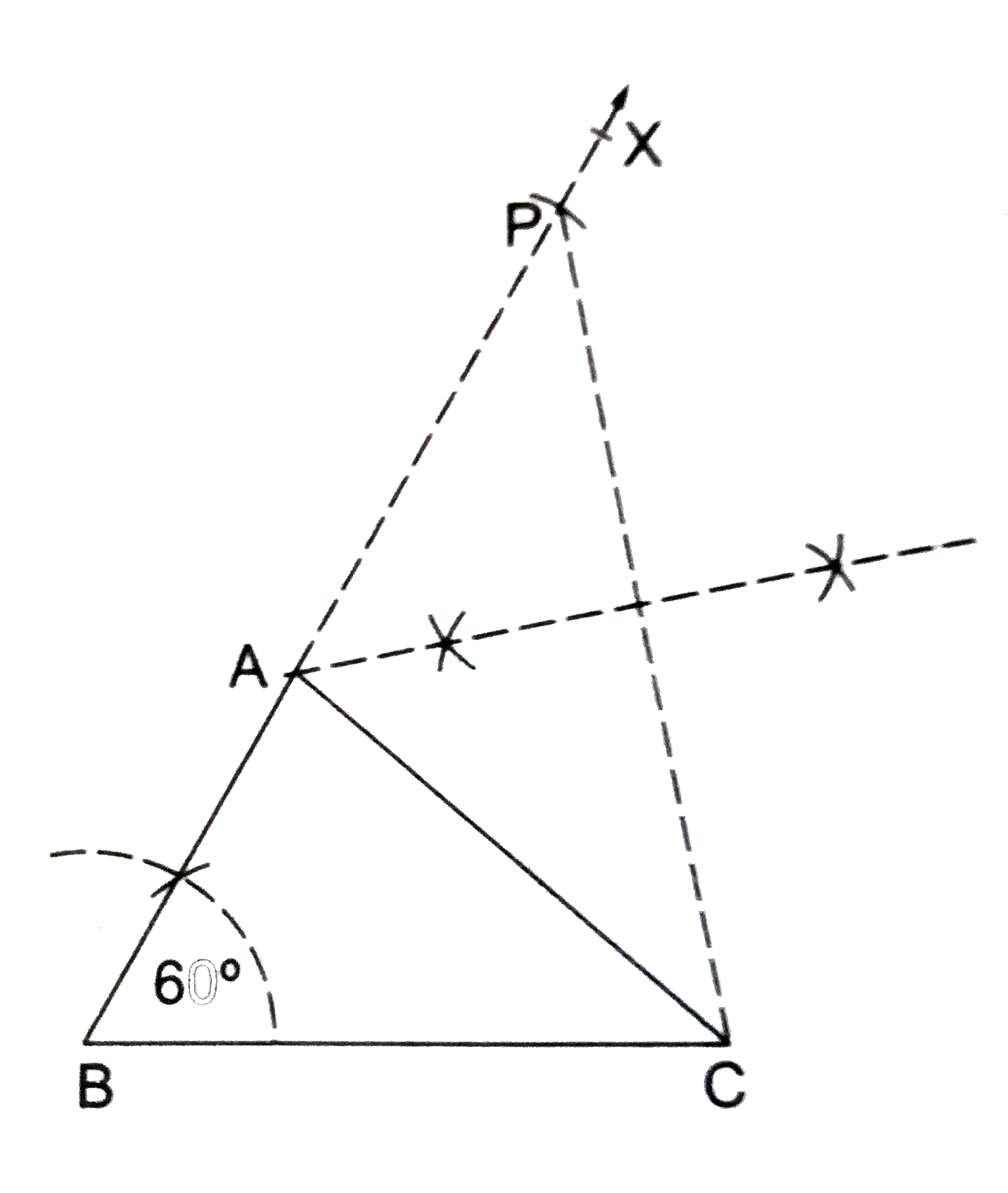
STEPS OF CONSTRUCTION
(i) Draw `BC=6` cm.
(ii) Construct `angleCBX=60^(@)`.
(iii) Along `BX`, set off `BP=9` cm.
(iv) Join CP.
(v) Draw the perpendicular bisector of CP to intersect BP at A.
(vi) Join AC.
Then, `Delta ABC` is the required triangle.
Verification :
On measuring, we find that
`AB+AC=(4+5)` cm `=9` cm.
Justification :
Clearly, A lies on the perpendicular bisector of CP.
`:. AP=AC`.
Now, `BP=9` cm [see Step (iii)]
`implies AB+AP=9` cm
`implies AB+AC=9 cm" "[ :' AP=AC]`.
Hence, `DeltaABC` is the required triangle.