A manufacturer makes two types, A and B of teapots. Three machines are needed for the manufacute and the time required for each teapot on the machines is given below.
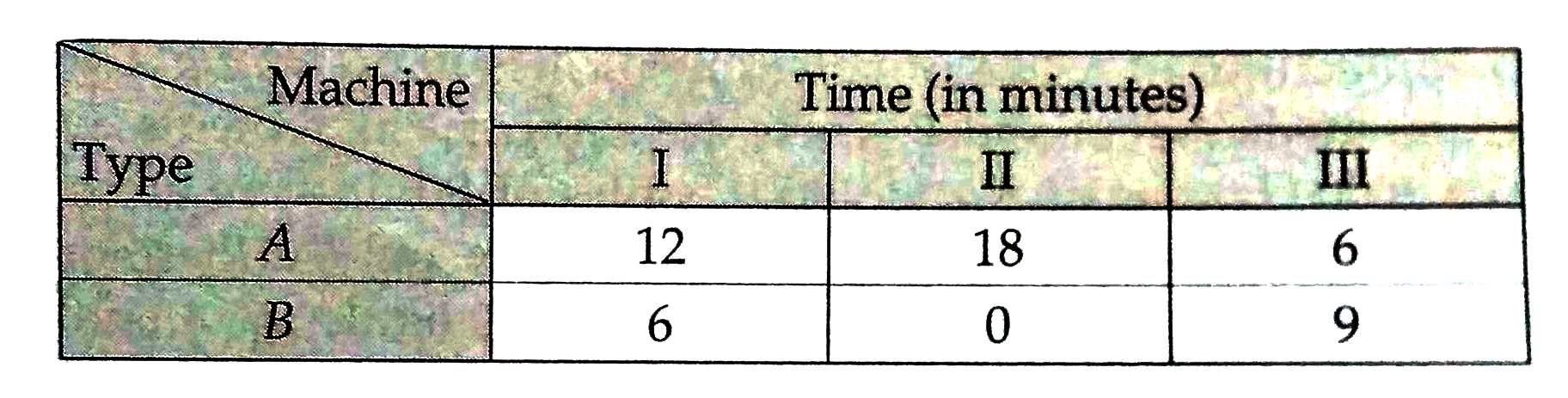
Each mahine is available for amaximum of 6 hours per day. If the profit on each teapot of type A is 75 paise and that on each teapot of type B is 50 paise, show that 15 teaport of type A and 30 of type B should be manufacured in a day to get the maximum profit.
Let x teaports of type A and y teapots of type B be manufactured. Then,
x≥0,y≥0,12x+6y≤6×60,18x+0y≤6×60,6x+9y≤6×60
⇒x≥0,y≥0,2x+y≤60,x≤20,2x+3y≤120.
Profit function is Z=75100x+50100y⇒Z=34x+12y.
Maximize Z, subject to the constraints
x≥0,y≥0,x≤20,2x+y≤60,2x+3y≤120.
Then x=15andy=30 will give the maximum vallue of Z.
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - LINEAR PROGRAMMINGEXERCISE - SECTION A4videos
LINEAR PROGRAMMING
- BOOK - RS AGGARWALCHAPTER - LINEAR PROGRAMMINGEXERCISE - SECTION B8videos
LINEAR PROGRAMMING
- BOOK - RS AGGARWALCHAPTER - LINEAR PROGRAMMINGEXERCISE - Exprcise 33B38videos
LINEAR PROGRAMMING
- BOOK - RS AGGARWALCHAPTER - LINEAR DIFFERENTIAL EQUATIONS EXERCISE - Objective Questions27videos
LINEAR DIFFERENTIAL EQUATIONS
- BOOK - RS AGGARWALCHAPTER - MATRICESEXERCISE - Exercise 5F21videos
MATRICES
Similar Questions
If A is a square matrix of order 3and|3A|=k|A| then find the value of k,
05:39View SolutionProve that each diagonal element of a skew-stmmetric matrix is zero.
View SolutionFind the maximum vlue of Z=7x+7y, subject ot the constrainst x≥0,y≥0,x+y≥2and2x+2y≤6.
02:10View SolutionThe solution of the DEdydx=ex+y is
02:17View SolutionConstruct a 2×3 matrix whose elements are given by
aij=12(i−2j)2.10:54View SolutionA manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the tune (in minutes) required for each toy on the machines is given below:Each machine is available for a maximum of 6 hours per day. If the profit on each toy of type A is Rs 7.50 and that on each toy of type B is Rs 5. show that 15 toys of type A and 30 of the B should be manufactured m a day to get maximum profit.
12:12View SolutionA manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the time (in minutes) required for each purpose and the time (in minutes) required for each toy on the machines is given below:
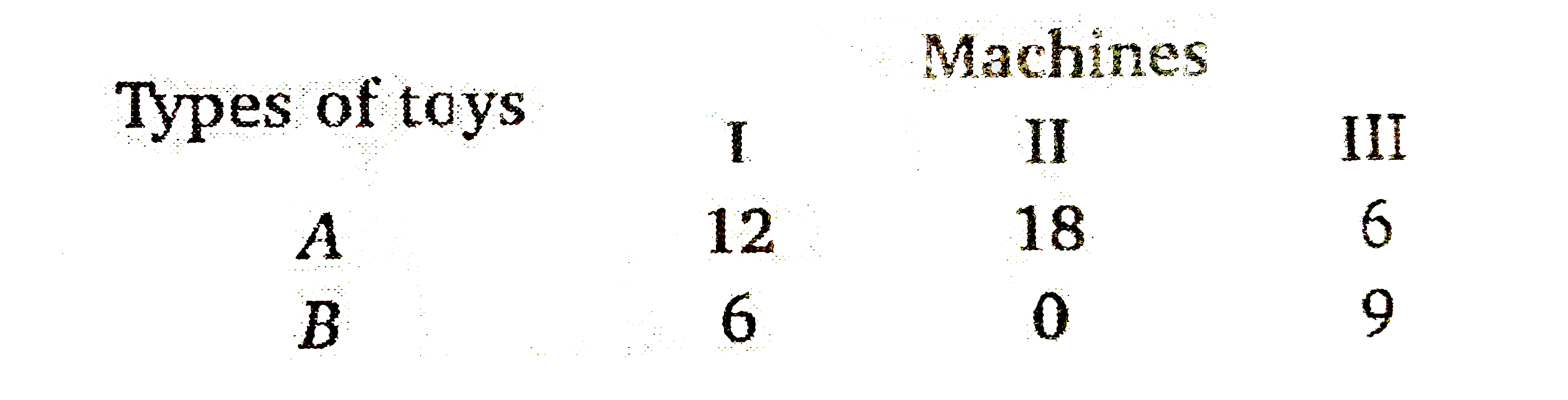
Each machine is available for a maximum of 6 hours per day. If the profit on each toy of type A is Rs. 7.50 and that on each toy of type B is Rs. 5, show that 15 toys of type A and 30 of type B should be manufactured in a day to get maximum profit.10:54View SolutionThe inequation represented by the graph given below is :
09:37View SolutionThe inequation that best describes the graph given below is _________
04:54View SolutionA manufacture makes two types of cups A and B. Three machines are required to manufacture the cups and the time in minutes required by each in as given below:

Each machine is available for a maximum period of 6 hours per day. If the profit on each cup A is 75 paisa and on B is 50 paisa, find how many cups of each type should be manufactures to maximise the profit per day.06:32View SolutionA manufacturer makes two types of toys A and B. Three machines are needed for this purpose and the time in minutes, required for each toy on the machines is given below:

Each machine is available for a maximum of 6 hours per day. If the profit on each toy of type A is Rs 7.50 and that on each toy of type B is Rs 5, show that 15 toys of type A and 30 of type B should be manufactured in a day to get maximum profit.14:04View SolutionA manufacturer makes two type of toys A and B. Three machines are needed for this purpose and the time (in minutes) required for each toy on the machines is given below :
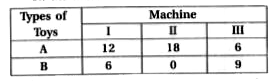
Each machine is available for a maximum of 6 hours per day. If the profit on each toy of type A is Rs. 7.50 and that on each toy of type B is Rs. 5, whow that 15 toys of type A and 30 of type B should be manufactured in a day to get maximum profit.13:50View SolutionA manufacturer maks two types A and B of tea cups. Three machines are needed for the manufacture and the time in minutes required for each cup on the machines is given below.

Each machine is available for a maximum of 6 hours per day. If the profit on each cup A is 75 paise and that on each cup B is 50 paise. How many both type of tea cups should be manufactured in a day to get the maximum profit ?06:29View SolutionA manufacturer makes two types of tea cups, say A and B. Three machines are needed for the manufacturing and the time in minutes required for each cup on the machine is given below:
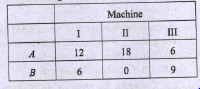
Each machine is available for a maximum of 6 hrs per day. If the profit on each cup A is 75 paise and that on each cup B is 50 paise. If x and y denote the number of cups of ttpe A and B produced in a day respectively. What is the profit function ?09:02View SolutionA manufacturer makes two types of tea cups, say A and B. Three machines are needed for the manufacturing and the time in minutes required for each cup on the machine is given below:
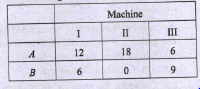
Each machine is available for a maximum of 6 hrs per day. If the profit on each cup A is 75 paise and that on each cup B is 50 paise. If x and y denote the number of cups of ttpe A and B produced in a day respectively. What are the linear in equations satisfied by x and y ?View SolutionA manufacturer makes two types of tea cups, say A and B. Three machines are needed for the manufacturing and the time in minutes required for each cup on the machine is given below:
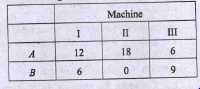
Each machine is available for a maximum of 6 hrs per day. If the profit on each cup A is 75 paise and that on each cup B is 50 paise. Show that 15 tea cups of type A and 30 tea cups of type B should be manufactured in a day to get the maximum profit.13:21View Solution

