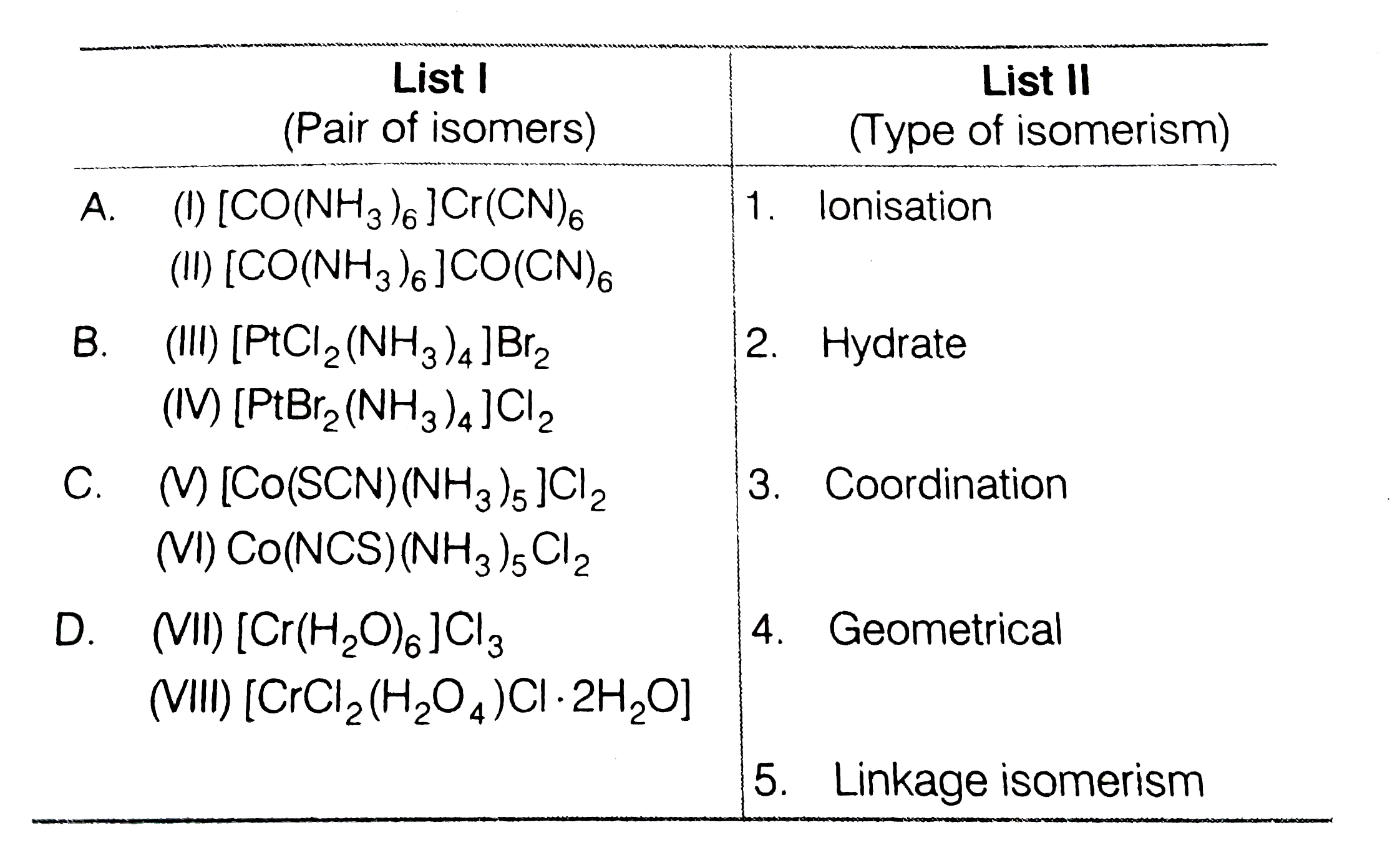
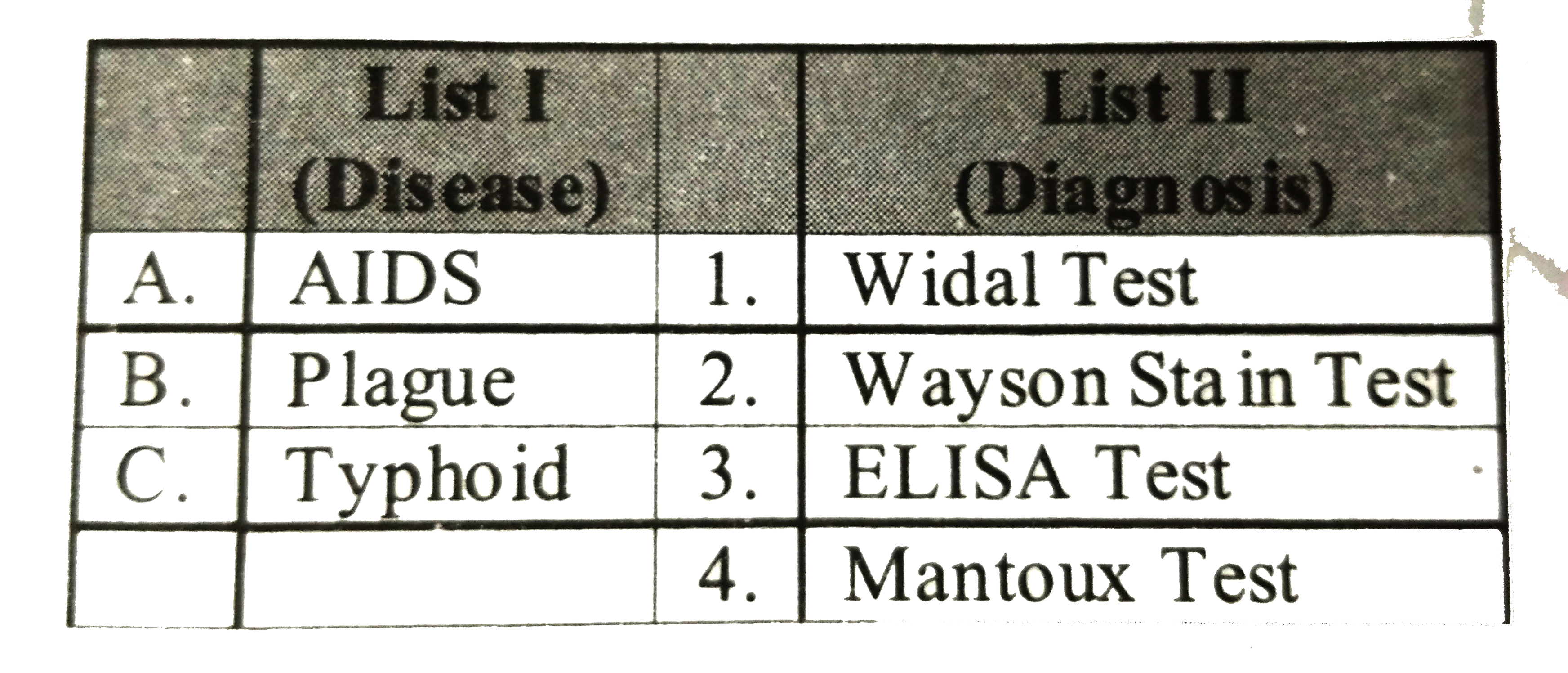Match List I with List II and select the correct answer using the code given below the lists.

A
PQRS4312
B
PQRS4321
C
PQRS3421
D
PQRS3412
Text Solution
The correct Answer is:A, B, C, D
P. Here, innermost function is inverse.
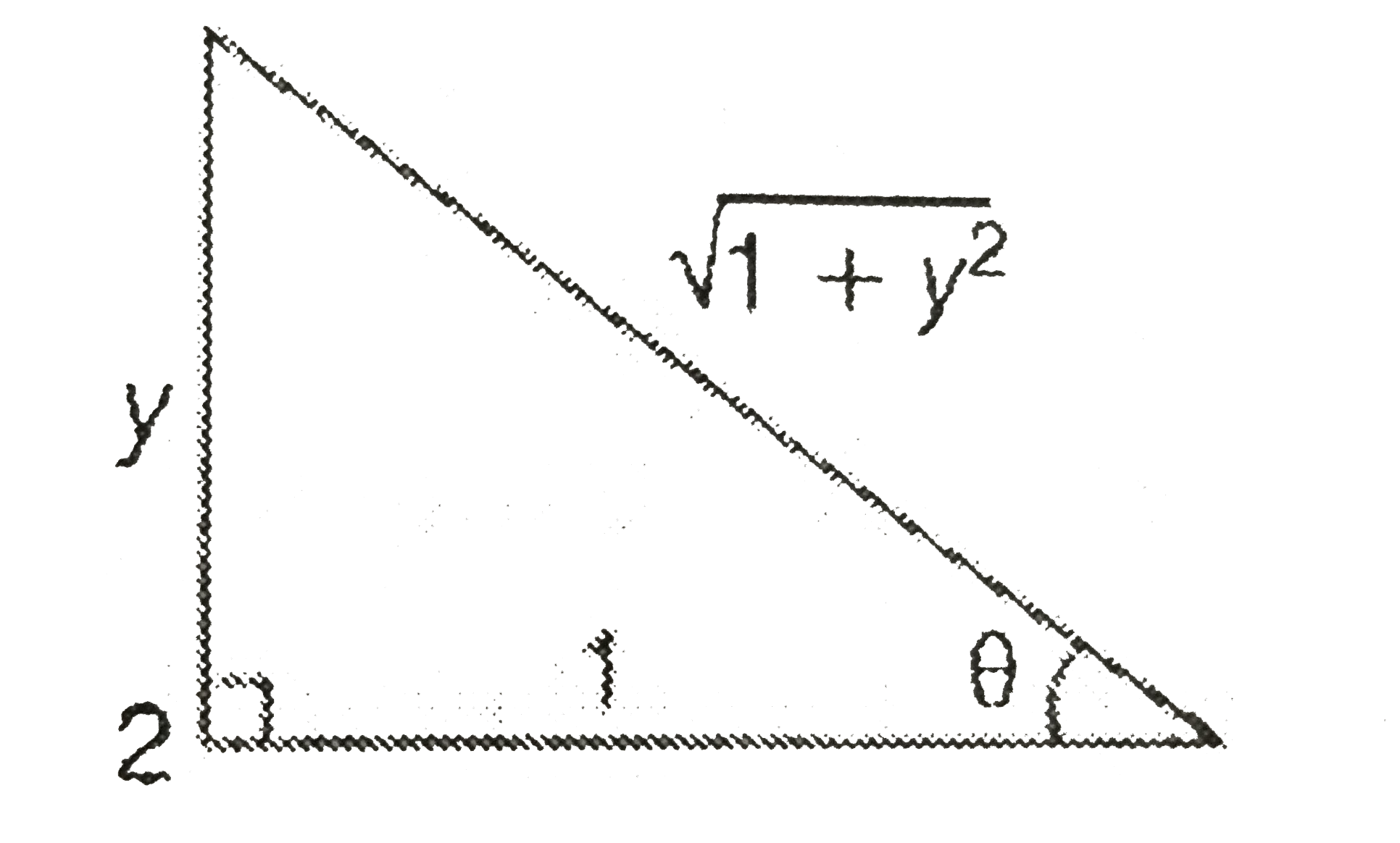
∴ Put tan−1y=θ⇒tanθ=y
⎡⎣1y2⋅{cos(tan−1y)+ysin(tan−1y)cos(sin−1y)+tan(sin−1y)}2+y4⎤⎦1/2
=⎡⎢
⎢
⎢
⎢
⎢⎣1y2⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩⎛⎜
⎜
⎜⎝1√1+y2+y2√1+y2/(√1−y2y+y√1−y2)⎫⎪
⎪
⎪⎬⎪
⎪
⎪⎭2+y4⎤⎥
⎥
⎥
⎥⎦12
=[1y2⋅y2(1−y4)+y4]1/2=1
Q. Given , cosx+cosy=−cosz
and sinx+siny=−sinz
On squaring and adding, we get
cos2x+sin2x+cos2y+sin2y+2cosxcosy+2sinxsiny=1
⇒2+2[cos(x−y)]=1⇒cos(x−y)=12
⇒2cos2(x−y2)−1=−12
⇒2cos2(x−y2)=12
R. cos2x⋅(cos(π4−x)−cos(π4+x))+2sin2x=2sinx⋅cosx
⇒cos2x⋅(√2sinx)+2sin2x=2sinx⋅cosx
⇒√2sinx[cos2x+√2sinx−√2cosx]=0
⇒sinx=0,(cosx−sinx)(cosx+sinx−√2)=0
⇒secx=1ortanx=1
⇒secx=1or√2
S. cot(sin−1√1−x2)=sin(tan−1(x√6))
⇒ x√1−x2=x√6√1+6x2
⇒1+6x2=6−6x2
⇒12x2=5⇒x=√512=√52√3
(P)→4,(Q)→3,(R)→2or4,(S)→1
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - INVERSE CIRCULAR FUNCTIONS EXERCISE - PROPERTIES OF INVERSE FUNCTIONS (NUMERICAL VALUE BASED)1videos
INVERSE CIRCULAR FUNCTIONS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - INVERSE CIRCULAR FUNCTIONS EXERCISE - PROPERTIES OF INVERSE FUNCTIONS ( ANALYTICAL & DESCRIPTIVE QUESTION)2videos
INVERSE CIRCULAR FUNCTIONS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - INVERSE CIRCULAR FUNCTIONS EXERCISE - PROPERTIES OF INVERSE FUNCTIONS (OBJECTIVE QUESTIONS II)8videos
INVERSE CIRCULAR FUNCTIONS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - INDEFINITE INTEGRATIONEXERCISE - IRRATIONAL FUNCTION & PARTIAL FRACTION4videos
INDEFINITE INTEGRATION
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - JEE MAINSEXERCISE - All Questions1videos
JEE MAINS
Similar Questions
Knowledge Check
- Question 1 - Select One
Match List I with List II and select the correct answer using the codes given below the lists:
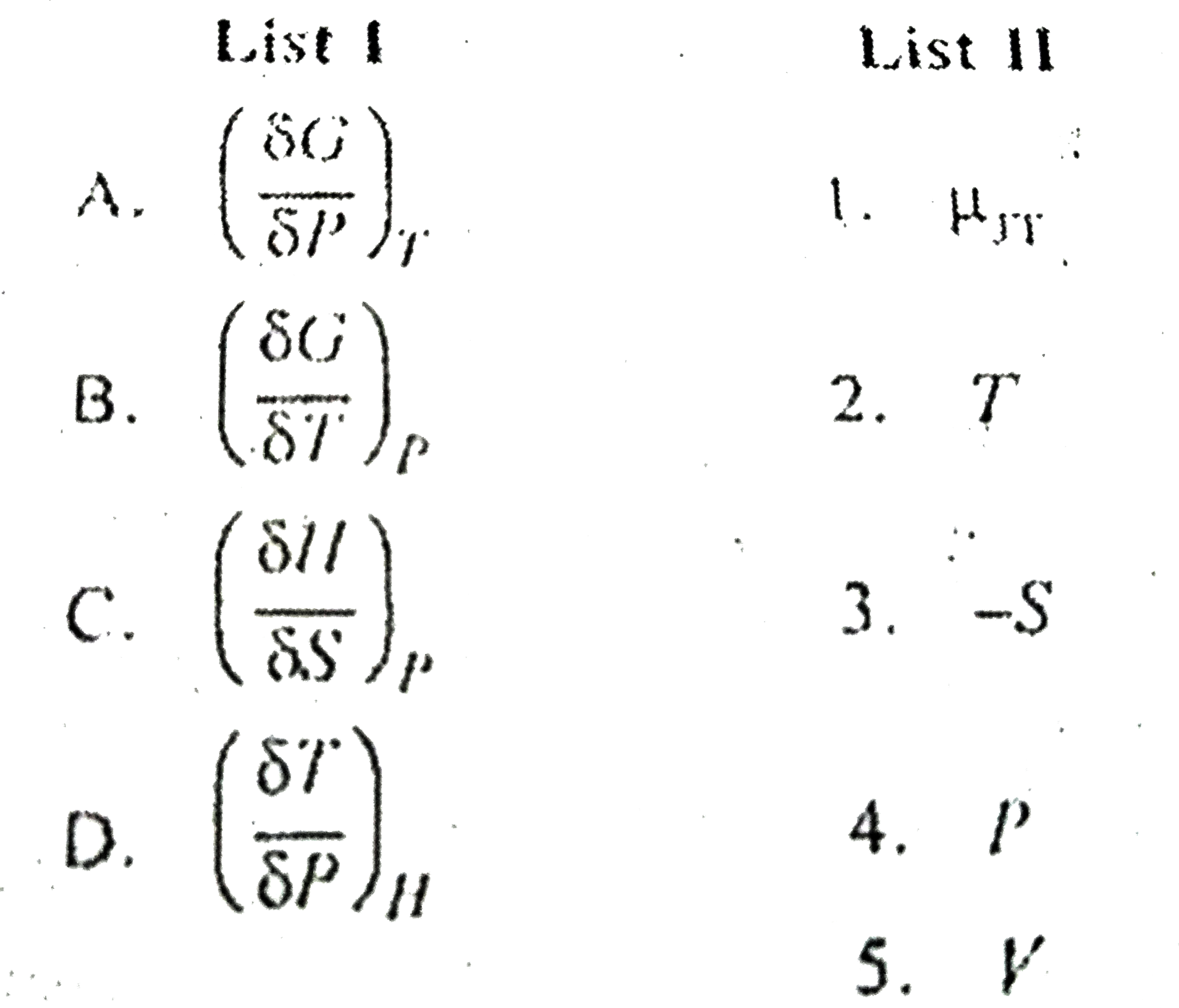 AabcdsrpqBabcdsrqpCabcdrsqpDabcdrspq
AabcdsrpqBabcdsrqpCabcdrsqpDabcdrspq - Question 2 - Select One
Match List I with List II and select the correct answer using the codes given below the lists:
 AabcdsrpqBabcdsrqpCabcdrsqpDabcdrspq
AabcdsrpqBabcdsrqpCabcdrsqpDabcdrspq - Question 3 - Select One
Match List I with List II and select the correct answer using the codes given below the lists:
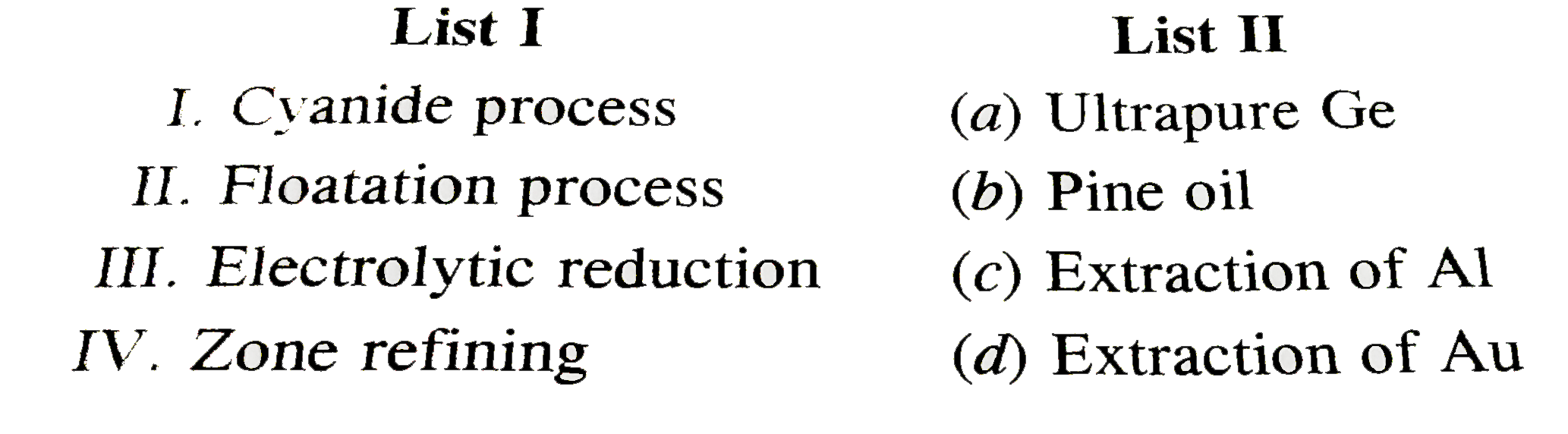 AabcdsrpqBabcdsrqpCabcdrsqpDabcdrspq
AabcdsrpqBabcdsrqpCabcdrsqpDabcdrspq
Match List I with List II and select the correct answer using the codes given below the lists :
 View Solution
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists:
 View Solution
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists:
 View Solution
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists :
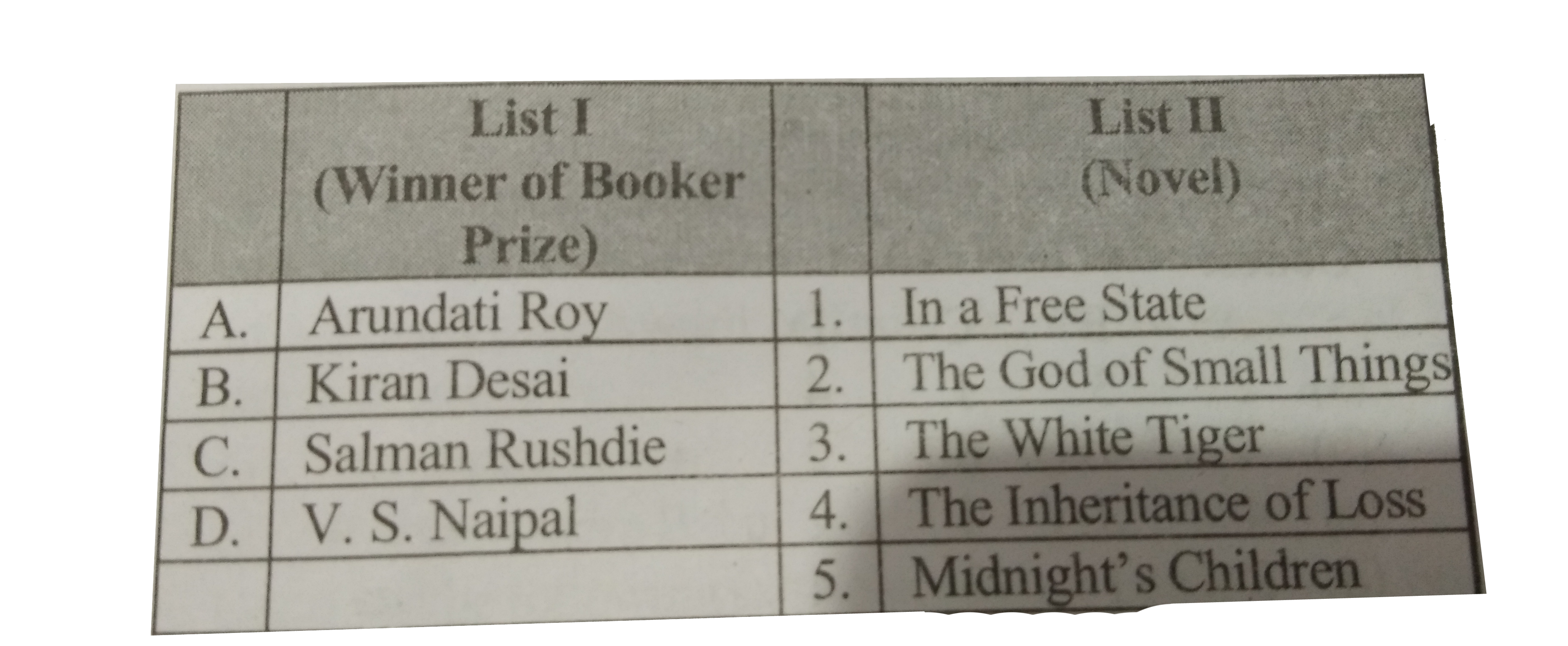 View Solution
View SolutionMatch List I with List II and select the correct answer using the codes given below the list :
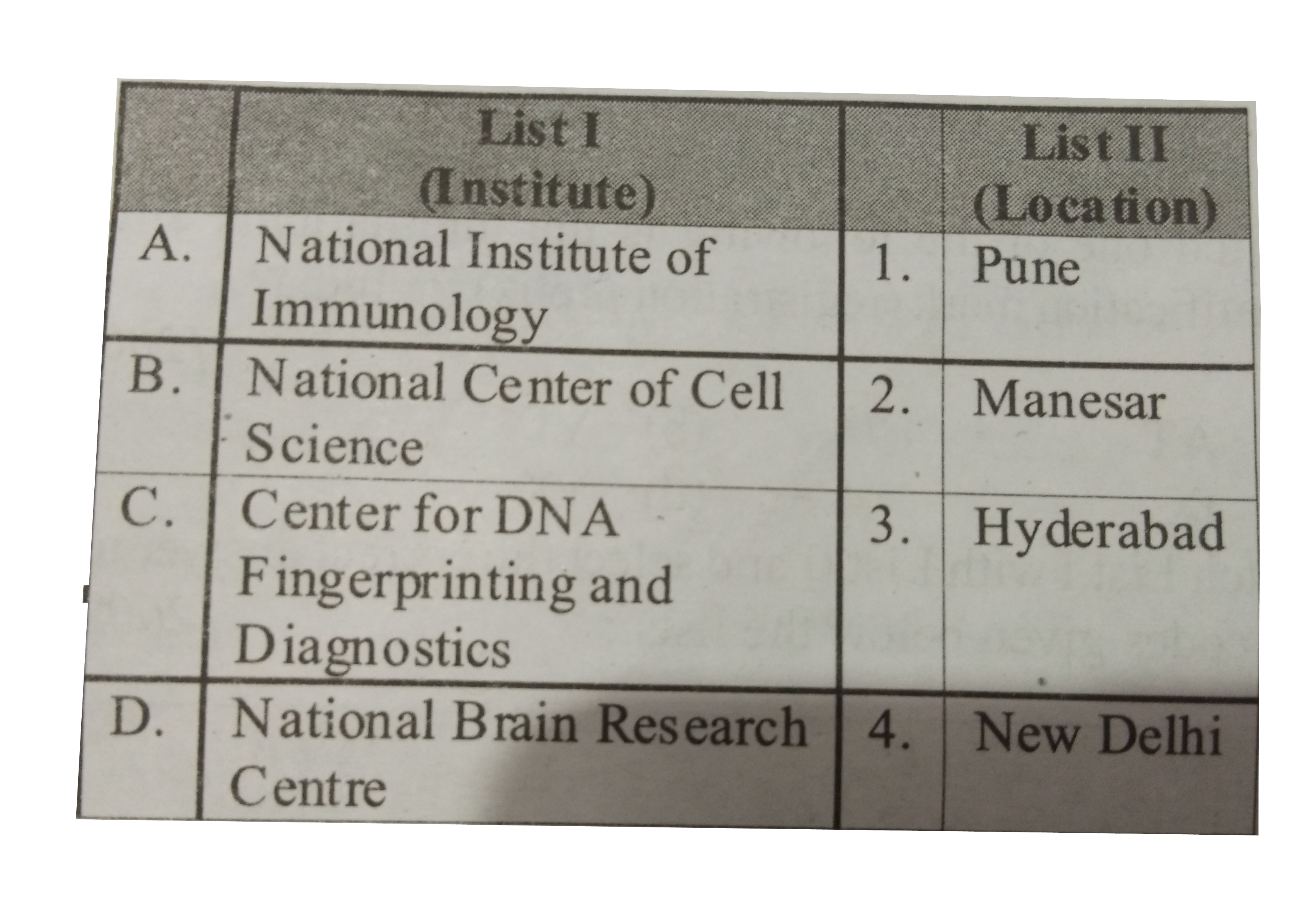 View Solution
View Solution