Prove, by vector method or otherwise, that the point of intersection of the diagonals of a trapezium lies on the line passing through the midpoint of the parallel sides (you may assume that the trapezium is not a parallelogram).

Video Solution

Text Solution

Verified by Experts

Let O be the origin of reference. Let the position vectors of A and B be $\to a and \to b$ respectively.

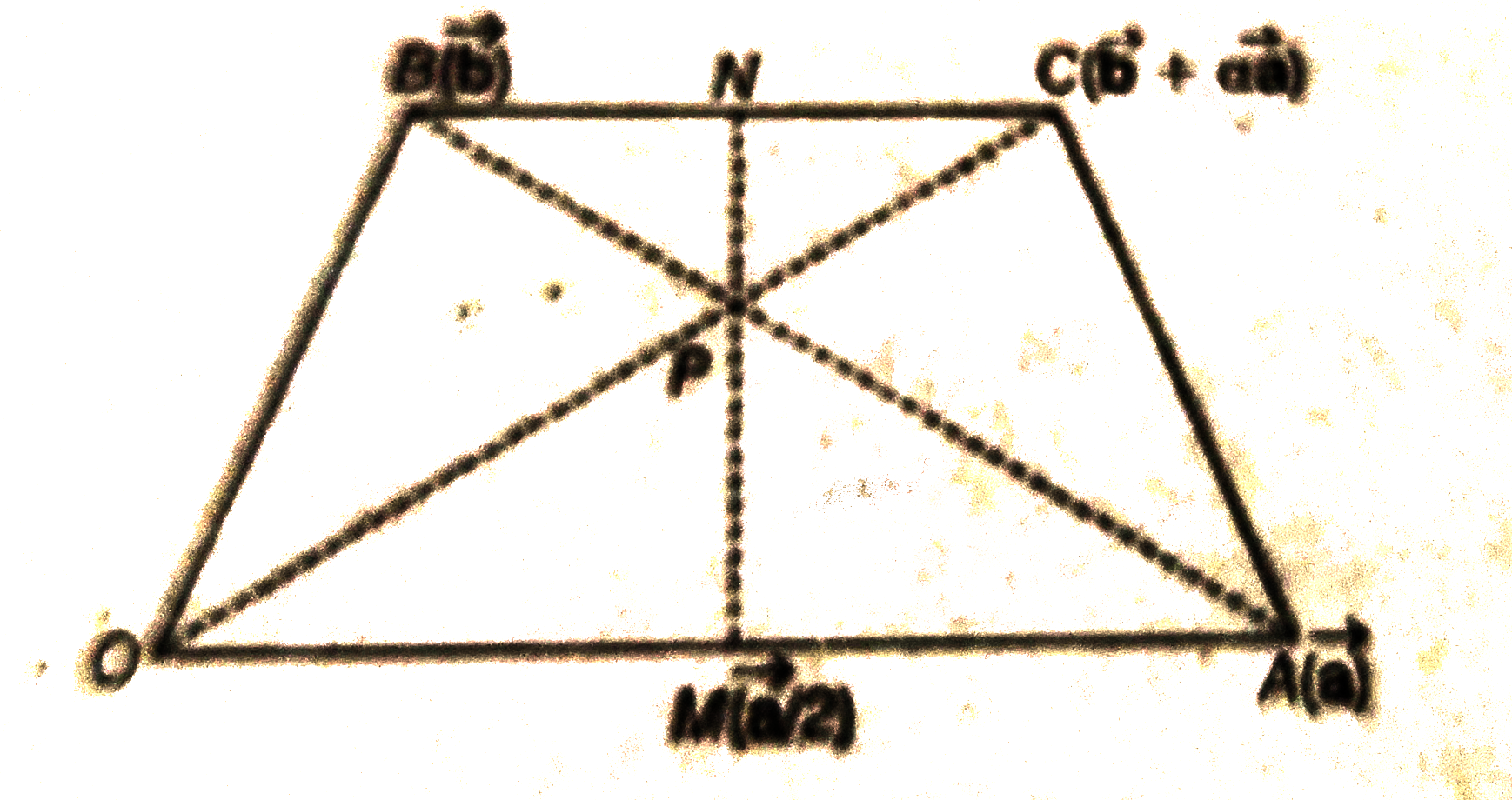
Since $B C ∣ ∣ O A, - - \to B A = α - - \to O A = a \to a$ for some constant $α$ .
Equation of $O C is \to r = t (\to b + α \to a)$ and
equation of $A B is \to r = \to a + λ (\to b - \to a)$
Let P be the point of intersection of $O C and A B$ . Then at point $P, t (\to b + α \to a) = \to a + λ (\to b - \to a)$ for some values of t and $λ$
$\Rightarrow (t α - 1 + λ) \to a = (λ - t) \to b$
Since $\to a and \to b$ are non-parallel vectors we must have
$t α = 1 + λ = 0 and λ = t \Rightarrow t = 1 / (α + 1)$
Thus position vectors of is ${\to r}_{1} = \frac{1,}{α + 1} (\to b + α \to a)$
Equation of MN is $\to r = \frac{1}{2} \to a + k [\to b + \frac{1}{2} (a - 1) \to a]$
For $k = 1 / (α + 1)$ {which is the coefficient of $\to b in {\to r}_{1}$ } we get
$\to r = \frac{1}{2} \to a + \frac{1}{α + 1} [\to b + \frac{1}{2} (α - 1) \to a]$
$= \frac{1}{α + 1} \to b + \frac{1}{α + 1} (α - 1 + α + 1) \to a$
$= \frac{1}{α + 1} (\to b + α \to a) = {\to r}_{1} \Rightarrow P$ lies on MN

Updated on:21/07/2023

The parallel sides of a trapezium are $a$ and $b$ respectively. The line joining the midpoints of its non-parallel sides will be
A $\frac{1}{2} (a - b)$
B $\frac{1}{2} (a + b)$
C $\frac{2 a b}{(a + b)}$
D $\sqrt{a b}$
The equation of a line passing through the point (4,-2,5) and parallel to the vector $3 ˆ i - ˆ j + 2 ˆ k$ is
A $\frac{x - 4}{3} = \frac{y + 2}{- 1} = \frac{z - 5}{2}$
B $\frac{x + 4}{3} = \frac{y - 2}{- 1} = \frac{z + 5}{2}$
C $\frac{x - 3}{4} = \frac{y + 1}{- 2} = \frac{z - 2}{5}$
D $\frac{x + 3}{4} = \frac{y - 1}{- 2} = \frac{z + 2}{5}$