If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:

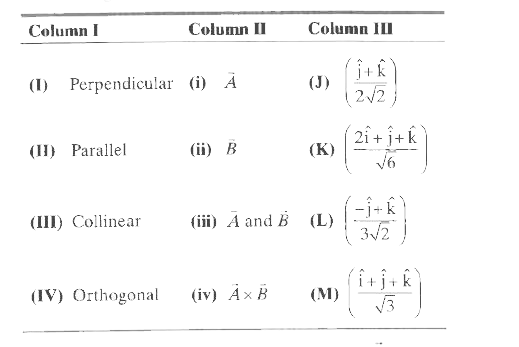
The correct alternative for a collinear vector to $\to A$ is

$(I I I) (i v) (L)$

$(I I) (i i) (K)$

$(I V) (i) (J)$

$(I) (i i i) (M)$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Answer

Step by step video, text & image solution for If vecA = 2 hati + hatj + hatk and vecB = hati + hatj + hatk are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table: The correct alternative for a collinear vector to vecA is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 PHYSICS BASIC MATHEMATICS

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:

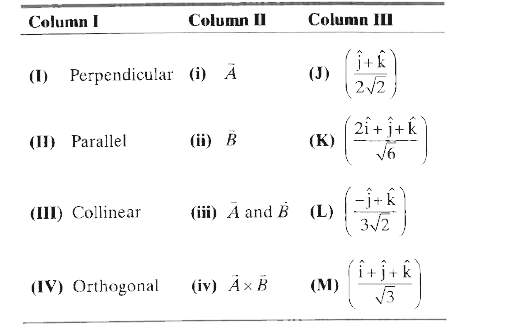
Which vector represents the direction of $\to A \times \to B ?$
A $(I I) (i v) (K)$
B $(I) (i i) (J)$
C $(I V) (i) (M)$
D $(I) (i v) (L)$
Question 2 - Select One
If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:

Which option represents a vector orthogonal to $\to A \times \to B$ ?
A $(I V) (i i) (M)$
B $(I I) (i i) (J)$
C $(I) (i) (L)$
D $(I I I) (i v) (K)$
Question 3 - Select One or More
If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are two vectores, then the unit vector is
Aperpendicular to $\to A i s (\frac{- ˆ j + ˆ k}{\sqrt{2}}) .$
Bparallel to $\to A i s (\frac{2 ˆ i + ˆ j + ˆ k}{\sqrt{6}}) .$
Cperpendicular to $\to A i s (\frac{- ˆ j + ˆ k}{\sqrt{2}}) .$
Dparallel to $\to A i s (\frac{ˆ i + ˆ j + ˆ k}{\sqrt{3}}) .$

RESNICK AND HALLIDAY-VECTORS -PRACTICE QUESTIONS

Home
Ask Doubt
Profile

If →A=2ˆi+ˆj+ˆkand→B=ˆi+ˆj+ˆk are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table: The correct alternative for a collinear vector to →A is

The correct Answer is:B

Topper's Solved these Questions

Similar Questions

Knowledge Check

If →A=2ˆi+ˆj+ˆkand→B=ˆi+ˆj+ˆk are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table: Which vector represents the direction of →A×→B?

If →A=2ˆi+ˆj+ˆkand→B=ˆi+ˆj+ˆk are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table: Which option represents a vector orthogonal to →A×→B ?

If →A=2ˆi+ˆj+ˆkand→B=ˆi+ˆj+ˆk are two vectores, then the unit vector is

RESNICK AND HALLIDAY-VECTORS -PRACTICE QUESTIONS

If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:

The correct alternative for a collinear vector to $\to A$ is

If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:

Which vector represents the direction of $\to A \times \to B ?$

If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are the two vectors, match the following answer the questions by appropriately matching the information given in the three columns of the following table:

Which option represents a vector orthogonal to $\to A \times \to B$ ?

If $\to A = 2 ˆ i + ˆ j + ˆ k and \to B = ˆ i + ˆ j + ˆ k$ are two vectores, then the unit vector is