Draw the graph of the greatest integer function: f:R→R:f(x)=[x]for all x∈R.
Video Solution
Text Solution
Using the definition of [x] we have
[x]=−2 for −2≥x<−1
[x]=−1for −1≤x<0
[x]=0 for 0≤x<1
[x]=1fro 1≤x<2
[x]=2 for 2≤x<3
[x]=3 for 3≤x<4.
Taking the scale: 10 small divisions = 1 unit, the graph of the function f(x)=[x] may be drawn as shown below.
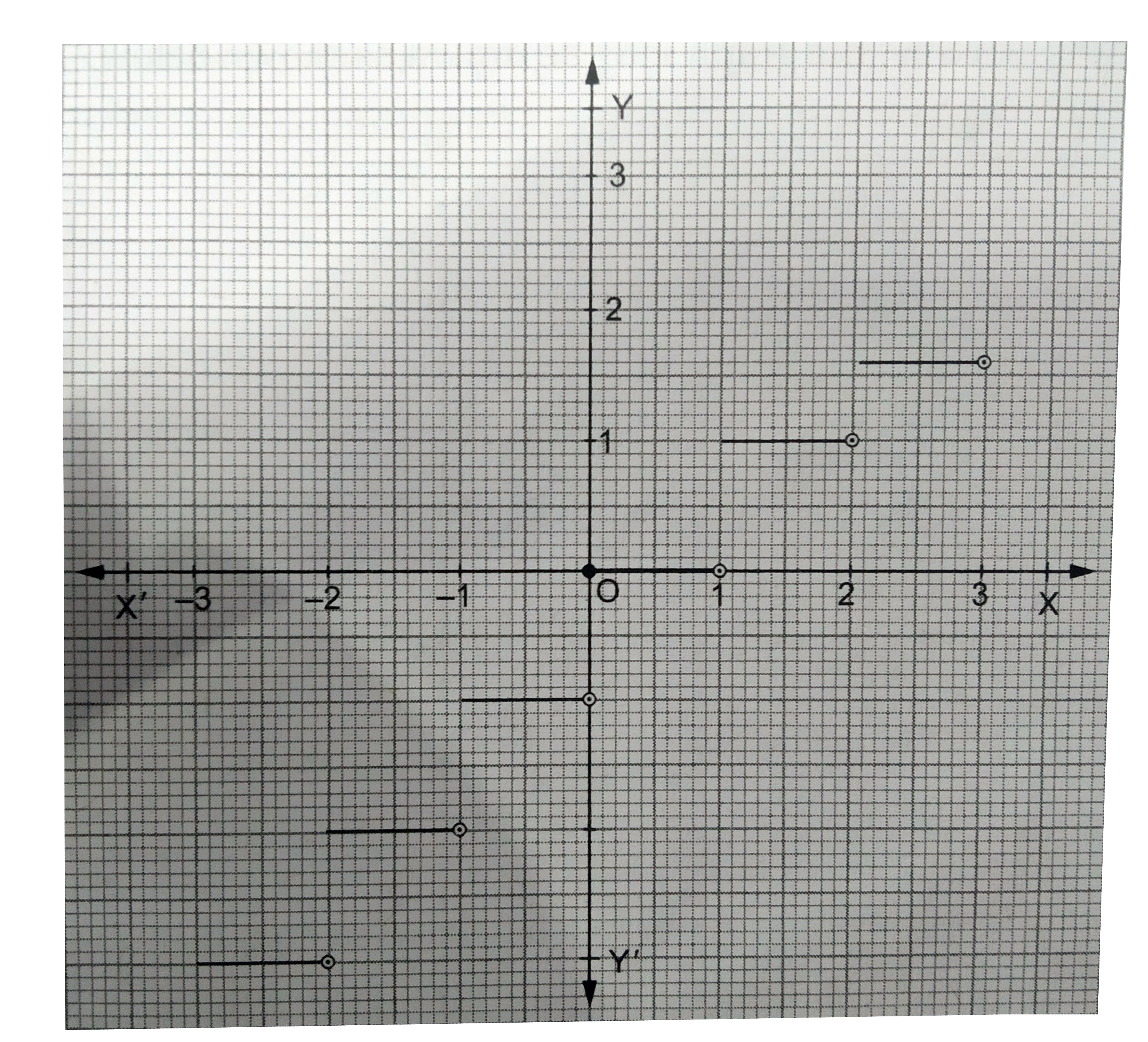
|
Topper's Solved these Questions
- FUNCTIONSBOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - PROBLEMS BASED ON DOMANIS AND RANGES OF REAL FUNCTIONS SOLVED EXAMPLES
23 Videos
- FUNCTIONSBOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - SOLVED EXAMPLES
20 Videos
- ELLIPSEBOOK - RS AGGARWALCHAPTER - ELLIPSEEXERCISE - EXERCISE
26 Videos
- GEOMETRICAL PROGRESSIONBOOK - RS AGGARWALCHAPTER - GEOMETRICAL PROGRESSIONEXERCISE - EXERCISE 12H (Very Short Answer Type Questions)
13 Videos
Similar Questions
Draw the graph of the smallest integer function f:R→R:f(x)=[x] for all x∈R.
View SolutionLet f:R→R:f(x)=x2 for all x∈R.
View SolutionDraw the graph of the fractional part function:
f:R→R:f(x)=x−[x]=[x]
View SolutionDraw the graph of the identity function f:R→R:f(x)=x for all x∈R.
View SolutionDraw the graph of the following function.
2f(x)
View SolutionDraw the graph of the following function.
f(2x)
View SolutionDraw the graph of the following function.
f(−x)
View Solutiondraw the graph of f(x)=x+[x], [.] denotes greatest integer function.
View SolutionDraw the graph of each of the following constant functions: (i) f(x)=2 for all x∈R (ii) f(x)=0 for all x∈R (iii)f(x)=-2 for all x∈R
View SolutionFind the domain and the range of the cube root function,
f:R+∪{0}→R:f(x)=x1/3 for all x∈R. Also, draw its graph.
View Solution
