Let f:R→R:f(x)=x2 for all x∈R.
Here, f:R→R:f(x)=x2 for all x∈R
Dom (f)=R and range (f)={x∈R:≥0}=[0,∞).
Now, we have:
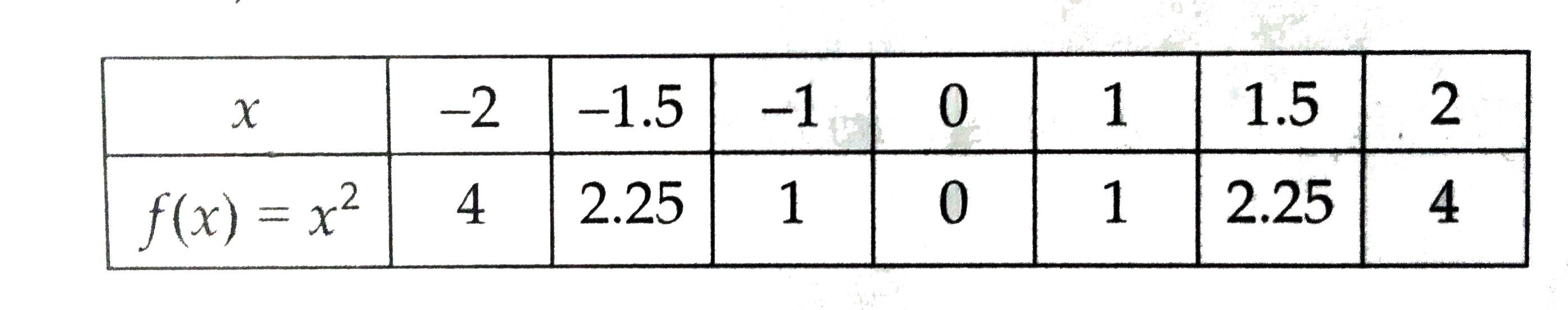
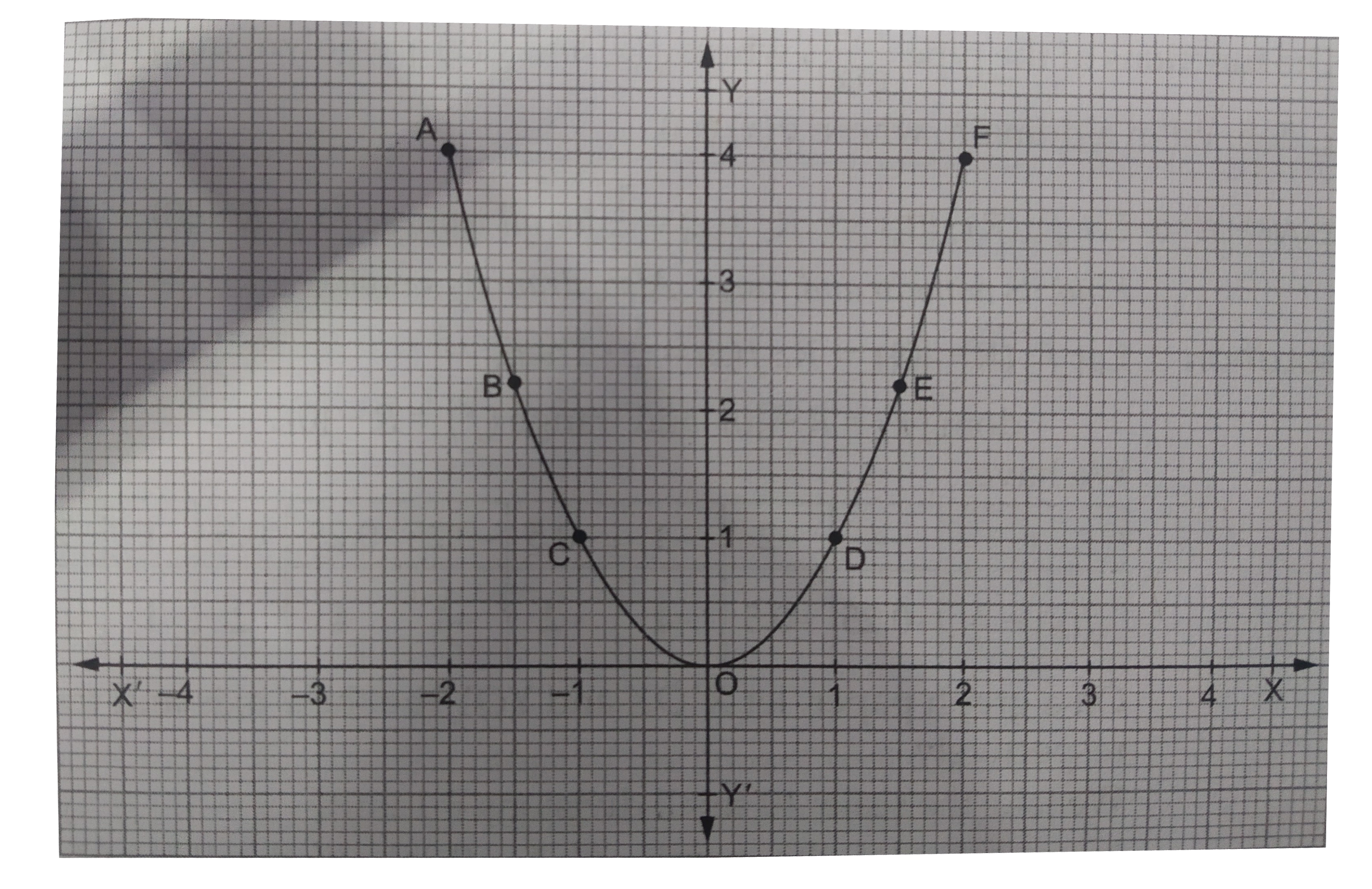
On a graph paper, we draw X' OX and YOY' as the x-axis and the y-axis respectively.
Now, we plot the points A(-2,4), B(-1.5), C(-1.1), O(0,0), D(1,1), E(1.5,2.25) and F(2,4).
Join these points freehand successively to obtain the required curve.
Scale: 10 small divisions = 1 unit
Topper's Solved these Questions
- FUNCTIONSBOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - PROBLEMS BASED ON DOMANIS AND RANGES OF REAL FUNCTIONS SOLVED EXAMPLES
23 Videos
- FUNCTIONSBOOK - RS AGGARWALCHAPTER - FUNCTIONSEXERCISE - SOLVED EXAMPLES
20 Videos
- ELLIPSEBOOK - RS AGGARWALCHAPTER - ELLIPSEEXERCISE - EXERCISE
26 Videos
- GEOMETRICAL PROGRESSIONBOOK - RS AGGARWALCHAPTER - GEOMETRICAL PROGRESSIONEXERCISE - EXERCISE 12H (Very Short Answer Type Questions)
13 Videos
Similar Questions
Knowledge Check
The function f.R→R defined by f(x)=(x2+1)35 for all x∈R is
Aone-one but not ontoBonto but not one-oneCneither one-one nor ontoDboth one-one and onto
Draw the graph of the smallest integer function f:R→R:f(x)=[x] for all x∈R.
View SolutionConsider the real function f:R→R:f(x)=x+5 for all x∈R. Find its domain and range. Draw the graph of this function.
View SolutionDraw the graph of the greatest integer function: f:R→R:f(x)=[x]for all x∈R.
View SolutionDraw the graph of each of the following constant functions: (i) f(x)=2 for all x∈R (ii) f(x)=0 for all x∈R (iii)f(x)=-2 for all x∈R
View SolutionFind whether the following are into functions (surjections) or not.
f:R→R defined by f(x)=x2+3 for all x∈R
View SolutionLet F:R→R be a thrice differntiable function. Suppose that F(1)=0,F(3)=−4 and F'(x)<0 for all xε(1/2,3). Let f(x)=xF(x) for all x∈R. Then the correct statement(s) is (are)
View SolutionLet f:R→R:f(x)=x2. Determine (i) range (f) (ii) {x:f(x)=4}
View Solution
