Consider the real function $f : R \to R$ , defined by
$f (x) = ⎧ ⎪ ⎨ ⎪ ⎩ \begin{matrix} 1 - x, when x < 0 1, when x = 0 x + 1, when x > 0 \end{matrix}$
Write its domain and range. Also, draw the graph of f(x).

Video Solution

Text Solution

Verified by Experts

$x < 0 \Rightarrow y = 1 - x$ .
$x = 1 \Rightarrow y = 1$ .
$x > 1 \Rightarrow y = x + 1$ .

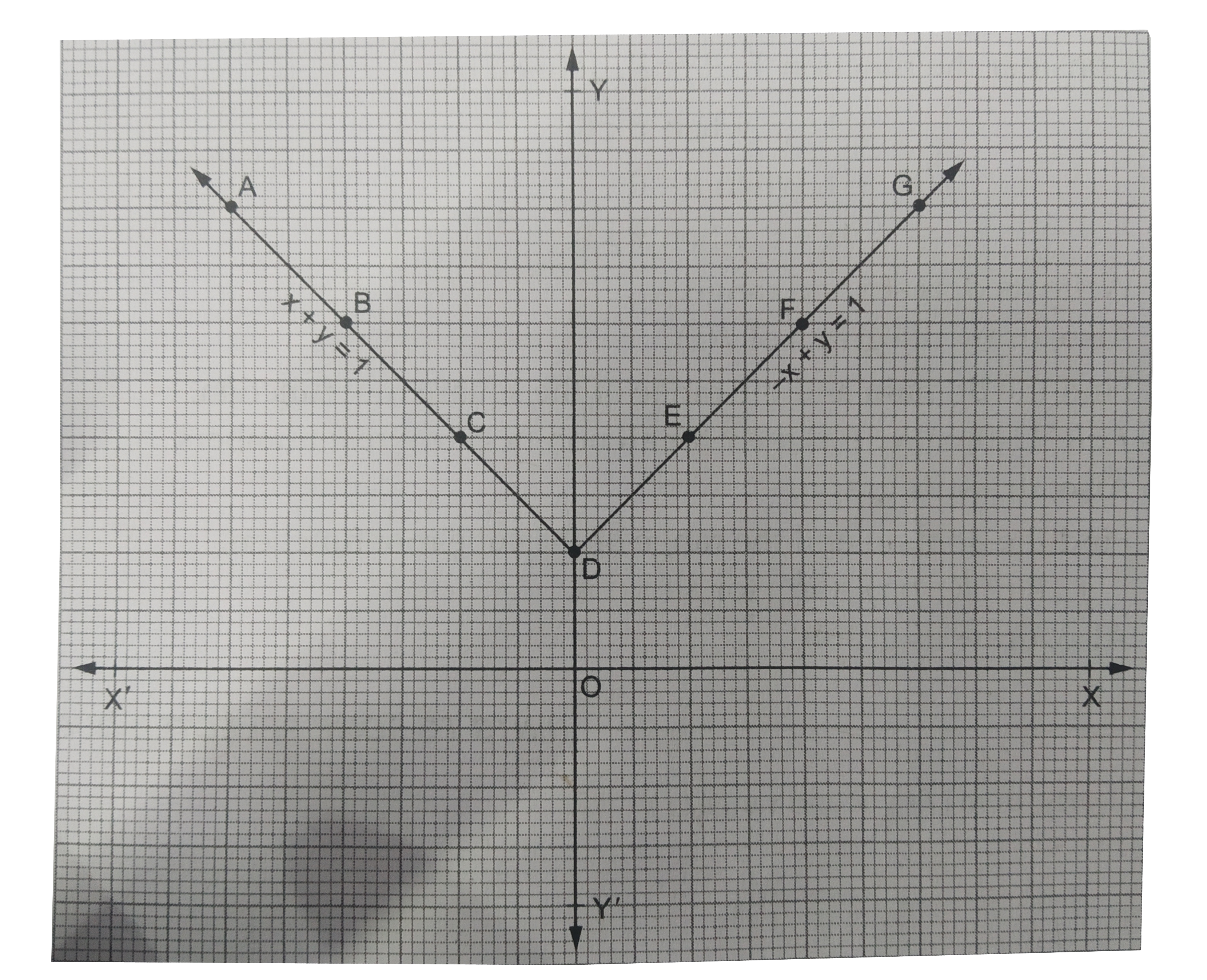
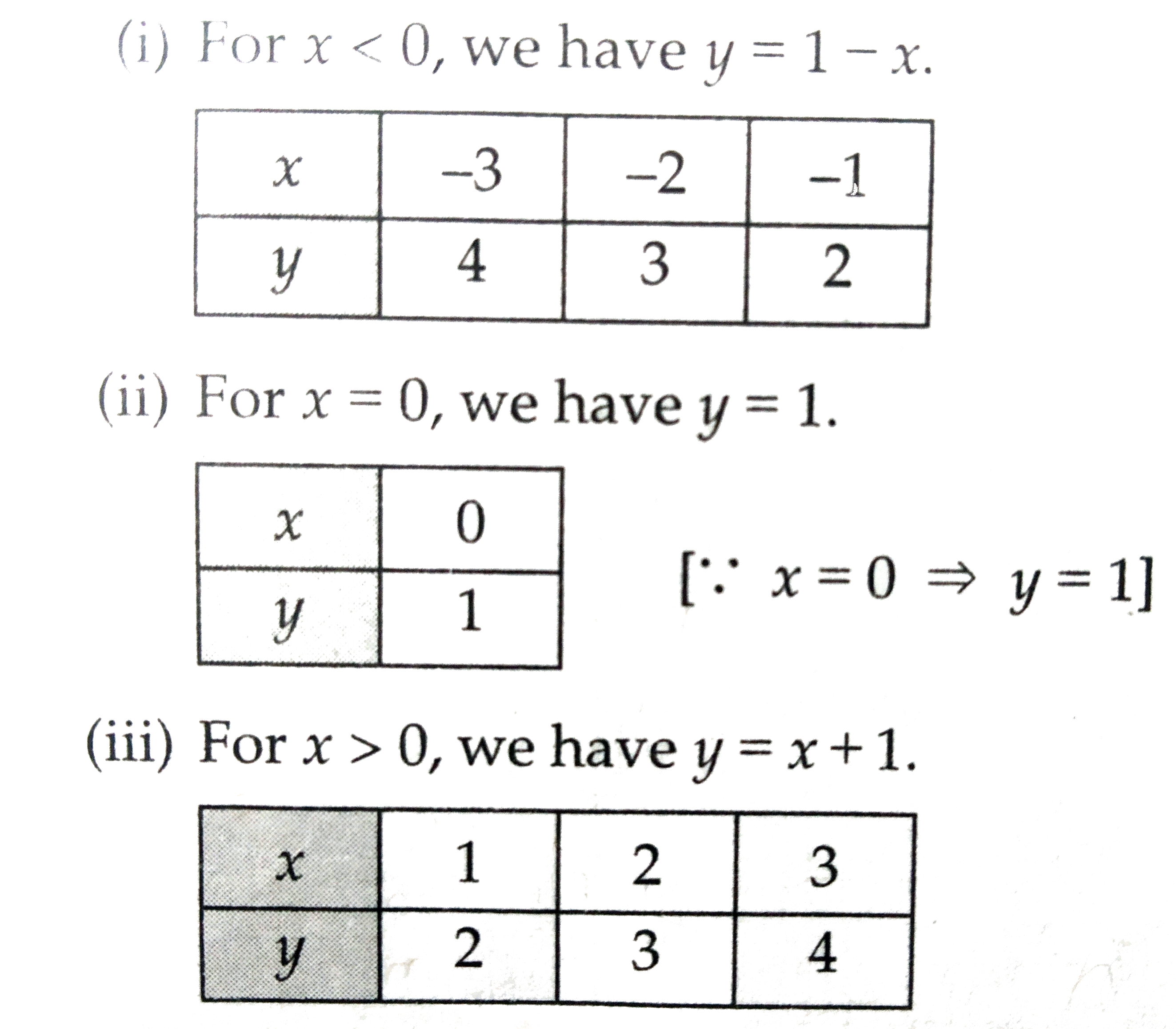
On a garph paper, draw horizontal line X' OX and the vertical line YOY'. Now, plot the points A(-3,4), B(-2,3), C(-1,2), D(0,1), E(1,2), F(2,3) and G(3,4).
Join ABCD and DEFG to get the required graph.

|

Updated on:21/07/2023

Class 11 MATHS RELATIONS AND FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The function f is defined as :
$f (x) = ⎧ ⎪ ⎨ ⎪ ⎩ \begin{matrix} 1, x > 0 0, x = 0 - 1, x < 0 \end{matrix}$
The range of f is :
A{1,0}
B{0,-1}
C{1,-1}
D{1,0,-1}
Question 2 - Select One
If $f (x) = ⎧ ⎪ ⎪ ⎨ ⎪ ⎪ ⎩ \begin{matrix} x - 1, & x < 0 \frac{1}{4}, & x = 0 x^{2}, & x > 0 \end{matrix}$ , then
A $lim x \to 0 + f (x) = 1$
B $lim x \to 0 - f (x) = 1$
Cf (X) is discontinuous at x = 0
DNone of these
Question 3 - Select One
If $f (x) = ⎧ ⎨ ⎩ \begin{matrix} \frac{x}{e^{\frac{1}{x}} + 1} & , & when x \neq 0 0 & , & when x = 0 \end{matrix}$ , then
A $lim x \to 0^{+} f (x) = 1$
B $lim x \to 0^{-} f (x) = 1$
Cf(x) is continuous at $x = 0$
Df(x) is discontinuous at $x = 0$

RS AGGARWAL-FUNCTIONS-EXERCISE 3D

Home
Ask Doubt
Profile