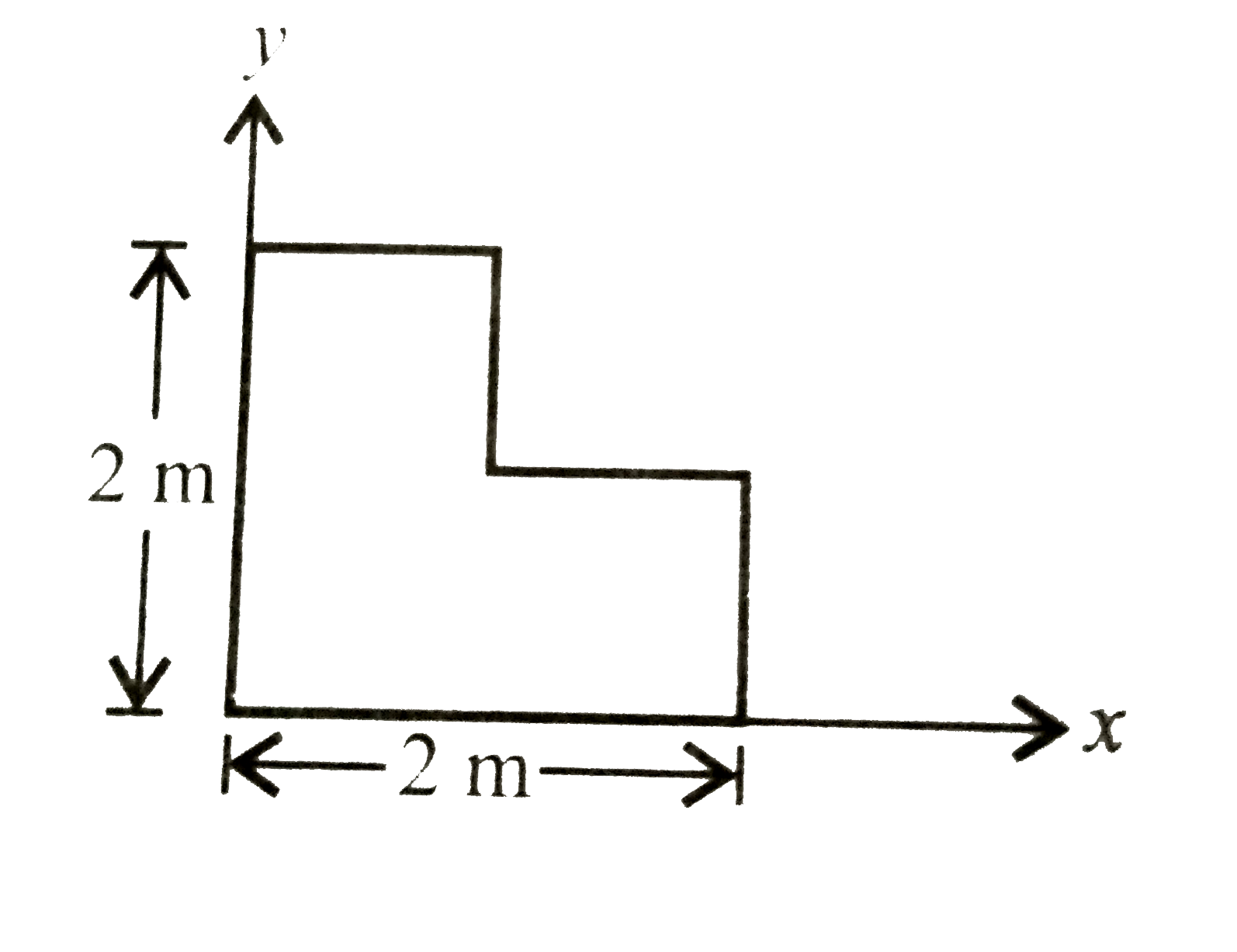
Find the centre of mass of a uniform L-shaped lamina (a thin flat plate) with dimensions as shown. The mass of the lamina is 3 kg.Solution in Kannada
Choosing the X and Y axes as shown in Fig. 7.11 we have the coordinates of the vertices of the L-shaped lamina as given in the figure. We can think of the L-shape to consist of 3 squares each of length 1m. The mass of each square is 1kg, since the lamina is uniform. The centres of mass C1,C2andC3 of the squares are, by symmetry, their geometric centres and have coordinates (1/2,1/2), (3/2,1/2), (1/2,3/2) respectively. We take the masses of the squares to be concentrated at these points. The centre of mass of the whole L shape (X, Y) is the centre of mass of these mass points.

Hence
X=[1(1/2)+1(3/2)+1(1/2)]kgm(1+1+1)kg=56m
Y=[1(1/2)+1(1/2)+1(3/2)]kgm(1+1+1)kg=56m
The centre of mass of the L-shape lies on the line OD. We could have guessed this without calculations. Can you tell why? Suppose, the three squares that make up the L shaped lamina of Fig. 7.11 had different masses. How will you then determine the centre of mass of the lamina?
Similar Questions
Find the centre of mass of a uniform L-shaped lamina (a thin flat plate) with dimensions as shown. The mass of the lamina is 3 kg.
View SolutionFind the centre of mass of a uniform L-shaped lamina (a thin flat plate) with dimensions as shown. The mass of the lamina is 3 kg.
View SolutionFind the centre of mass of a uniform L-shaped lamina (a thin flat plate) with dimensions as shown. The mass of the lamina is 3 kg.
View SolutionFind the centre of mass of a uniform L shaped lamina (a thin flat plate) with dimension as shown in Fig. The mass of the lamina is 3kg.

View SolutionThe x, y coordinates of the centre of mass of a uniform L-shaped lamina of mass 3 kg is
View SolutionFind the position of centre of mass of the uniform lamina shown in figure.

View SolutionThe coordinates of the centre of mass of a uniform flag -shaped lamina (thin flat plate) of mass 4kg .( The coordinates of the same are shown in the figure) are :
View Solution
