Find the equation of the circle with center at (3,−1) and which cuts off an intercept of length 6 from the line 2x−5y+18=0
Video Solution
Text Solution
The correct Answer is:(x−3)2+(y+1)2=38
Let the circle be as shown below.
CM=|2(3)−(5(−1)+18)|√22+(−5)2=√29
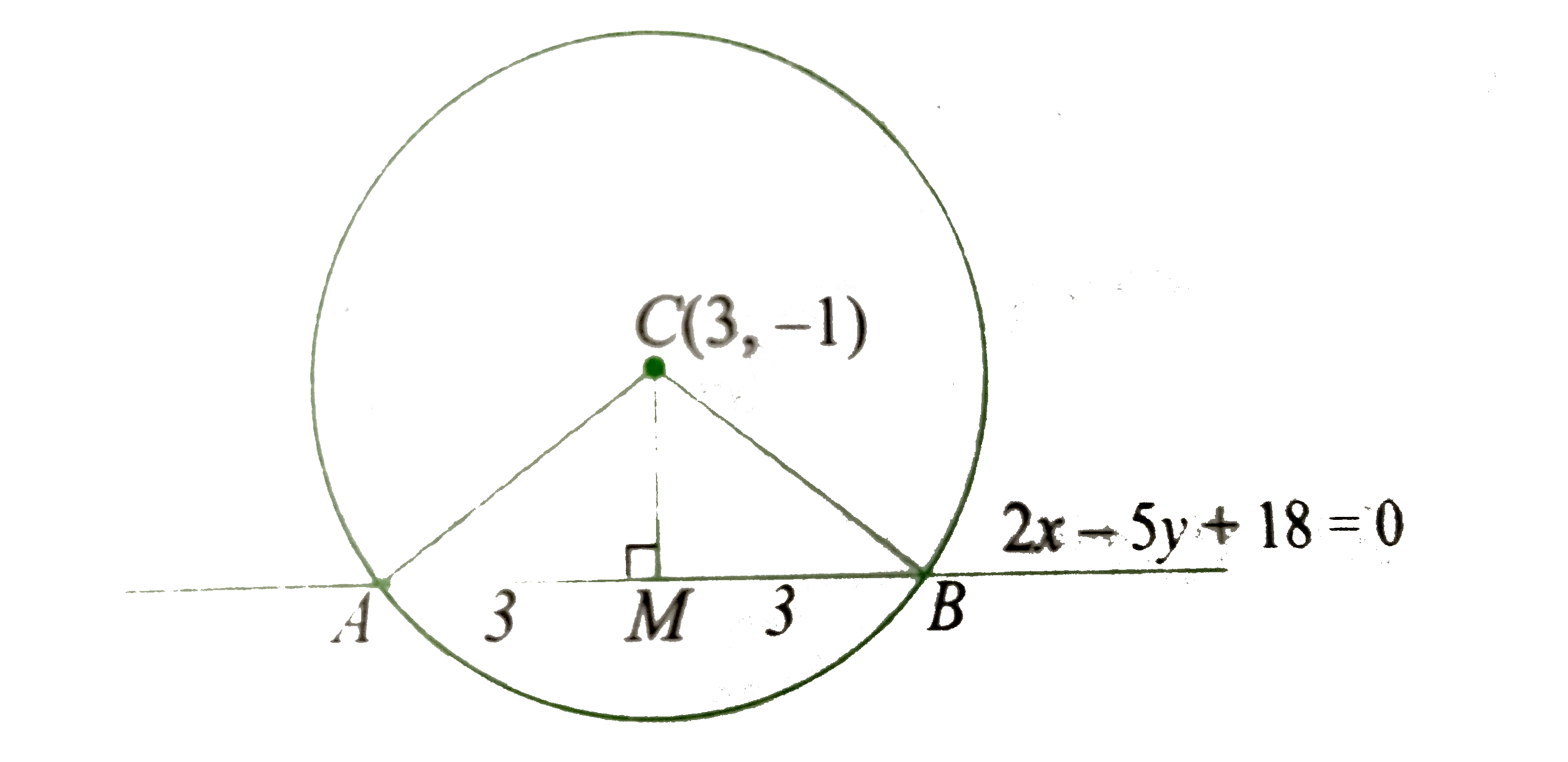
∴AC2=AM2+CM2=9+29=38
∴ Radius =√38
|
Topper's Solved these Questions
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.2
5 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - Exercise 4.3
18 Videos
- CIRCLE BOOK - CENGAGECHAPTER - CIRCLE EXERCISE - JEE Advanced (Single Correct Answer Type)
14 Videos
- BINOMIAL THEORMBOOK - CENGAGECHAPTER - BINOMIAL THEORMEXERCISE - Question Bank
31 Videos
- CIRCLESBOOK - CENGAGECHAPTER - CIRCLESEXERCISE - Question Bank
32 Videos
Similar Questions
Knowledge Check
Equation of circle centred at (3,-2) , which cuts off a chord
of length 6 from the line 4x−3y+2=0 , isA(x−3)2+(y+2)2=5B(x+3)2+(y−2)2=5Cx2+y2−6x+4y−12=0Dx2+y2−4x+6y+12=0Radius of the circle with centre (3,1) and cutting a chord of length 6 on the line 2x+5y+18=0 is
A6B7C√38D2√5If a circle has centre (3,-1) and cut-off an intercept of length 6 from the line 2x-5y+18=0. Then, the equation of the circle is
Ax2+y2−6x+2y+28=0Bx2+y2+6x+2y−28=0Cx2+y2−6x−2y+28=0Dx2+y2−6x+2y−28=0
Find the equation of the circle , centred at (1,4) , which
cuts off of a chord of length 4 units on the line 3x+4y+1=0
View SolutionLines 5x+12y−10=0 and 5x−12y−40=0touch a circle C1 of diameter 6.If the centre of C1 lies in the first quadrant, find the equation of the circle C2 which is concentric with C1 and cuts, intercepts of length 8 on these lines.
View SolutionFind the equation of the circle , which passes through the origin and cuts off intercepts a and b from the axes.
View SolutionFind the equation of circle which passes through origin and cuts off intercepts of unit length on lines y2−x2=0.
View SolutionFind the equation of the straight line which passes though (1,−3) and cuts off equal intercepts on the axes.
View Solution
