An ideal gas in a thermally insulated vessel at internal pressure = P1, volume = V1 and absolute temperature = T1 expands irreversibly against zero external pressure, as shown in the diagram. The final internal pressure, volume and absolute temperature of the gas are P2,V2 and T2, respectively. For this expansion.

q=0
T2=T1
P2V2=P1V1
P2Vγ2=P1Vγ1
The correct Answer is:A, B, C
Since the vessel is thermally insulated so
q=0
Pext=0, so w=0
so ΔU=0 (ideal gas)
Hence ΔT=0
⇒ΔT=0⇒T2=T1⇒P2V2=P1V1
The process is however adiabatic irriversible.
So we cannot apply P2Vγ2=P1Vγ1
Topper's Solved these Questions
- THERMODYNAMICS AND THERMOCHEMISTRY BOOK - ERRORLESS CHAPTER - THERMODYNAMICS AND THERMOCHEMISTRY EXERCISE - JEE section (Reasoning type questions)
6 Videos
- THERMODYNAMICS AND THERMOCHEMISTRY BOOK - ERRORLESS CHAPTER - THERMODYNAMICS AND THERMOCHEMISTRY EXERCISE - JEE section (Comprehension type questions)
9 Videos
- THERMODYNAMICS AND THERMOCHEMISTRY BOOK - ERRORLESS CHAPTER - THERMODYNAMICS AND THERMOCHEMISTRY EXERCISE - JEE section (Only one correct answer)
42 Videos
- SOME BASIC CONCEPTS OF CHEMISTRYBOOK - ERRORLESS CHAPTER - SOME BASIC CONCEPTS OF CHEMISTRYEXERCISE - JEE Section (Matrix Match type questions)
3 Videos
Similar Questions
Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
View SolutionTwo containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
View Solution
Knowledge Check
An ideal gas in thermally insulated vessel at internal (pressure)=P1,(volume)=V1 and absolute temperature=T1 expands irreversiby against zero external, pressure , as shown in the diagram,
The final internal pressure, volume and absolute temperature of the gas are p2,V2andT2, respectively . For this expansion
 Aq=0BT2=T1Cp2V2=p1V1DP2Vγ2=P1Vγ2
Aq=0BT2=T1Cp2V2=p1V1DP2Vγ2=P1Vγ2Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
AP1T1+P2T2BP1T1+P2T2(T1+T2)2CP1T2+P2T2(T1+T1)2DP12T1+P22T2Two containers of equal volume contain the same gas at pressure P1 and P2 and absolute temperature T1 and T2, respectively. On joining the vessels, the gas reaches a common pressure P and common temperature T. The ratio P/T is equal to
Ap1T2+p2T1T1×T2Bp1T2+p2T1T1+T2C12[p1T2+p2T1T1T2]Dp1T2−p2T1T1×T2]
A gas enclosed in a vessel has pressure P, volume V and absolute temperature T, write the formula for number of molecule N of the gas.
View SolutionA gas enclosed in a vessel has pressure P, volume V and absolute temperature T, write the formula for number of molecule N of the gas.
View SolutionTwo gases which are at pressure p1 volume V1 and temperature T1 and pressure p2 volume V2 and temperature T2, respectively are forced into a vessel of volume V at temperature T. Calculate pressure of the mixture.
View SolutionIf P, V and T stand for pressure, volume and absolute temperature of a gas, which of the following will be true for Boyle's Law
View SolutionTwo identical vessels contain the same gas at pressure P1 and P2 at absolute temperature T1andT2, respectively. On joining the vessels with a small tube as shown in the figure. The gas reaches a common temperature T and a common pressure P. Determine the ratio P/T
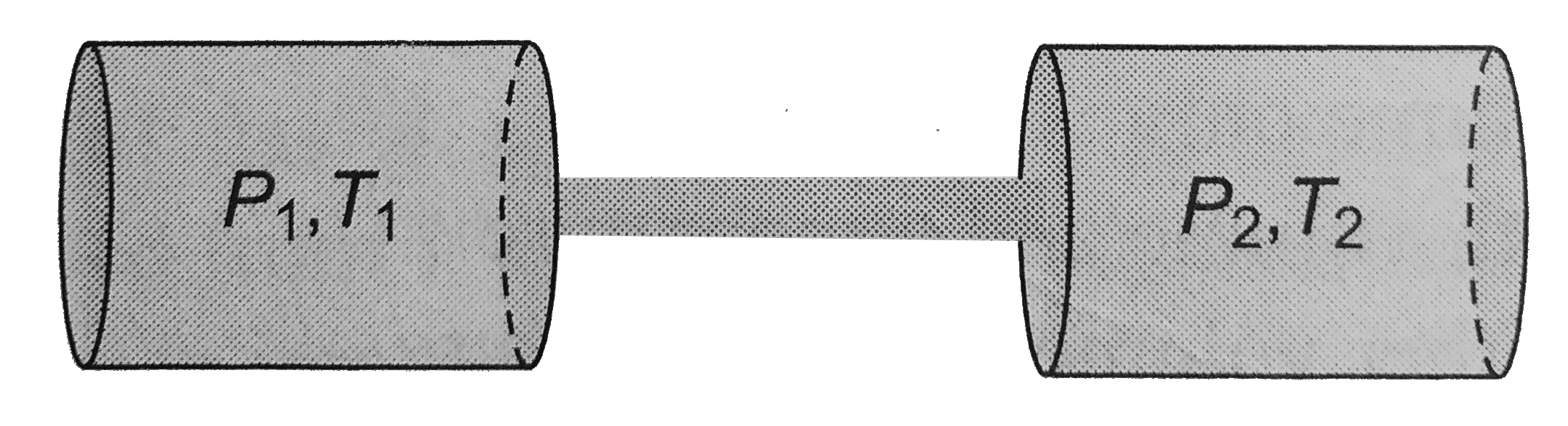
View Solution
