If sin(A+B)=1 and cos(A−B)=1, 0∘≤(A+B)≤90∘ and A>B then find A and B.
To solve the problem step-by-step, we start with the given equations and conditions:
1. Given Equations:
- sin(A+B)=1
- cos(A−B)=1
2. Understanding the Sine Function:
- The sine function equals 1 at 90∘. Therefore, we can conclude:
A+B=90∘
3. Understanding the Cosine Function:
- The cosine function equals 1 at 0∘ and 360∘. However, since A−B must be within the range of angles we are considering, and given that A+B=90∘, we will focus on:
A−B=0∘
- This implies:
A=B
4. Substituting A = B into A + B = 90:
- If A=B, then we can substitute B for A in the equation A+B=90∘:
A+A=90∘⟹2A=90∘⟹A=45∘
5. Finding B:
- Since A=B, we have:
B=45∘
6. Final Values:
- Therefore, the values of A and B are:
A=45∘,B=45∘
Summary of the Solution:
- A=45∘
- B=45∘
- sin(A+B)=1
- cos(A−B)=1
- The sine function equals 1 at 90∘. Therefore, we can conclude:
A+B=90∘
- The cosine function equals 1 at 0∘ and 360∘. However, since A−B must be within the range of angles we are considering, and given that A+B=90∘, we will focus on:
A−B=0∘
- This implies:
A=B
- If A=B, then we can substitute B for A in the equation A+B=90∘:
A+A=90∘⟹2A=90∘⟹A=45∘
- Since A=B, we have:
B=45∘
- Therefore, the values of A and B are:
A=45∘,B=45∘
- A=45∘
- B=45∘
Topper's Solved these Questions
Similar Questions
If sin(A−B)=12 and cos(A+B)=12, 0∘<(A+B)<90∘ and A>B then find A and B.
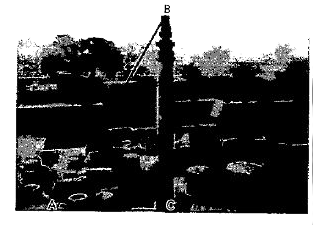
View SolutionPillars of Ashoka are a series of columns in Indian subcontinent. One of the Pillars of Ashoka is located in Vaishali having a single lion capital and another inside the Allahabad Fort, known as Allahabad pillar or Ashoka Stambha, shown as BC in the figure below. The point A is taken on the ground such that ΔABC forms a right-angled triangle. If sin(A + B) = 1 and sin(A−B)=12, 0∘≤A−B≤90∘, A>B, the values of A and B are:
View SolutionIf sin (A - B) = 0 , cos (A + B) = 0, 0∘<A+B≤90∘, find A and B.
View SolutionIf tan(A+B)=√3 and tan(A−B)=1, 0∘<A+B<90∘ and A>B , find the values of A and B.
View SolutionIf sin(A−B)=12 and cos(A+B)=12, 0∘<(A+B)<90∘ and A>B then find A and B.
View SolutionIf sin(A+B)=√32andsin(A−B)=12,0∘<A+B≤90∘,A>B, then find the value of A and B.
View SolutionIf sin(A−B)=12 and cos(A+B)=12, find A and B.
View SolutionIf tan(A+B)=√3 and tan(A−B)=1√3,0∘<A+B≤90∘,A>B , find A and B .
View SolutionIf tan(A+B)=√3 and tan(A−B)=1√3,0∘<A+B≤90∘,A>B, then find the value of A and B.
View SolutionIf sin(A-B) = 1/2 and cos(A+B) = 1/2,0^@ < A+B < 90^@ and A>B, then find A and B.
View Solution
