Question

In a non-right -angled triangle $\Delta PQR$, let p,q,r denote the lengths of the sides opposite to the angles at P,Q,R respectively. The median from R meets the side PQ at S, the perpendicular from P meets the side QR at E, and RS and PE intersect at O. if $p=\sqrt{3},q=1$,and the radius of the circumcircle of the $\Delta PQR$ equals 1, then which of the following options is/are correct ?

Options

A. Length of `OE=(1)/(6)`
B. Length of `RS =(sqrt(7))/(2)`
C. Area of `Delta SOE=(sqrt(3))/(2)`
D. Radius of incircle of `Delta PRQ =sqrt(3)/(2)(2-sqrt(3))`

Answer

A::B::D

Explanation

Let a non- right angled `Delta PQR`,Now, by sine rule.

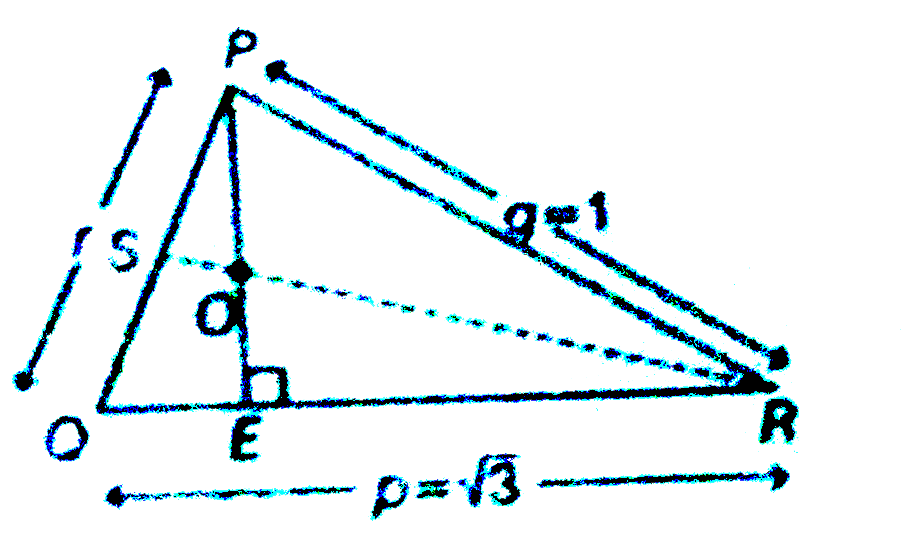
`(P)/(sin P)=(q)/(sin Q)=(r)/(sin R)=2xx` circumradius
`rArr (sqrt(3))/(sinP) =(1)/(sin Q)=(r)/(sin R)=2xx1 " " ["cirumradius =1 unit"]`
`rArr sin P =sqrt(3)/(2) and sin Q=(1)/(2)`
`rArr P=120^@ and Q=30^@` (`because Delta PQR` is non -right angled triangle)
So, `R=30^@`
`rArr =1`, so `Delta PQR` is an isoceles triangle. And, sinces RS and PE are the median of `Delta PQR`, so 'O' is centroid of the `Delta PQR`.
Now,
Option (a), From Apollonitus therorem,
`2(PE^2+QE^2)=PQ^2+PR^2+PR^2rArr 2(PE^2+(3)/(4))=1+1`
`rArr PE^2=1-(3)/(4)rArr PE^2=(1)/(4)rArr PE =(1)/(2) "units"`
and `OE=(1)/(3)PE=(1)/(6)"units" [because O "divides" PE is 2:1]`
Option (b) ,
Again from Apollonius theorem,
`2(PS^2+RS^2)=PR^2+QR^2rArr 2((1)/(4)+RS^2)=1+3`
`rArr RS^2=2-(1)/(4)rArr RS^2=(7)/(4)rArr RS=(sqrt(7))/(2)"units"`
Option (c),
Area of `Delta SOE =(1)/(2)(OE)(ST)`
`=(1)/(2)xx(1)/(6)[(PS)sin 60^@]`
`=(1)/(12)xx(1)/(2)xx(sqrt(3))/(2)`
`=(sqrt(3))/(48)` square units.
Option (d),
`because "Inradius of " Delta PQR =(Delta)/(s)=((1)/(2)pqsinR)/((1)/(2)(p+q+r))=((1)/(2)(sqrt3)(1)(1)/(2))/((1)/(2)(sqrt(3)+1+1))`
`=(sqrt(3))/(2)(2-sqrt(3))"units"`
Hence, options (a), (b) and (d) are correct.