Let the point B be the reflection of the point $A (2, 3)$ with respect to the line $8 x - 6 y - 23 = 0$ Let $⌈_{A} and ⌈_{B}$ respectively. Be circles of radil 2 and I which centres A and B respectively. Let T be a common tangent to the circles $⌈ A and ⌈ B$ such that both the circles are on the same side of T. If C is the point of intersection of T and the line passing thorugh A and B, then the length of the line segment AC is .............

Text Solution

Verified by Experts

The correct Answer is:10

Accroding to given information the figure is as following

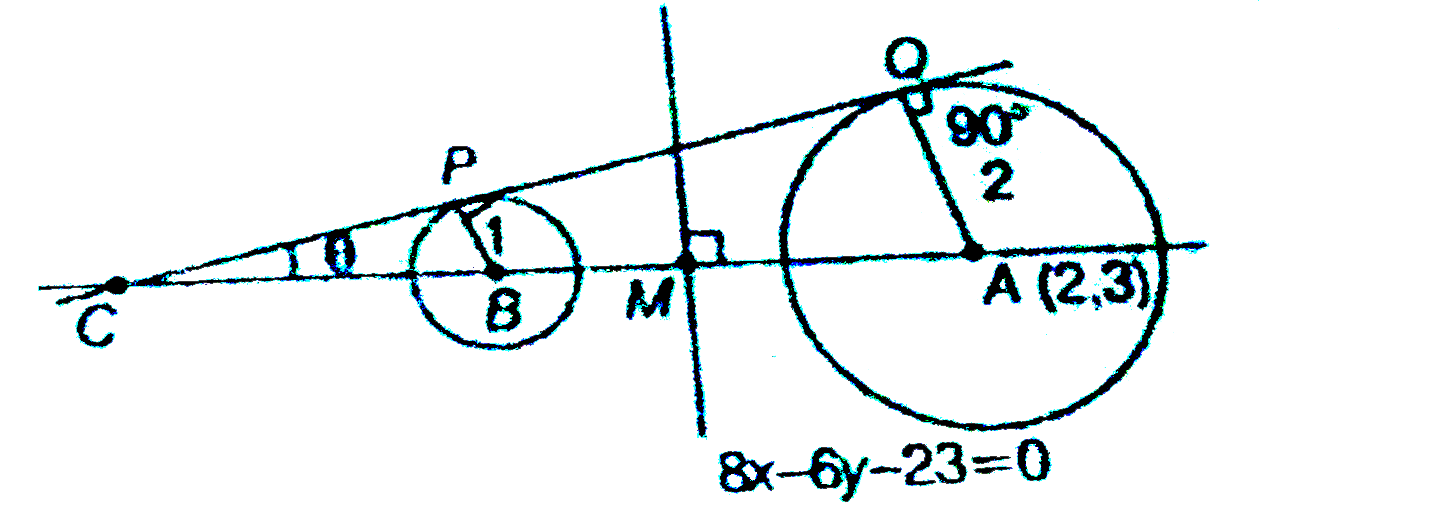
From the figure, $A C = \frac{2}{sin θ} ... . . (i)$
$∵ sin θ = \frac{1}{C B}$
$(from Δ C P B) ... ... (i i)$
$and sin θ = \frac{2}{A C} = \frac{2}{C B + A B} (from Δ C Q A) ... ... (i i i)$
$∵ A B = A M + M B = 2 A M$
$= 2 \frac{| (8 \times 2) - (6 \times 3) - 23 |}{\sqrt{64 + 36}} = \frac{2 \times 25}{10} = 500$
From Eqs. (ii) and (iii), we get
$sin θ = \frac{1}{C B} = \frac{2}{C B + A B}$
$\Rightarrow \frac{1}{C B} = \frac{2}{C B + 5}$
$\Rightarrow C B + 5 = 2 G B \Rightarrow C B = 5 = \frac{1}{sin θ}$
From the Eq.(i),
$A C = \frac{2}{sin θ} = 2 \times 5 = 10, 00$ .

|

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

T is a point on the common tangents at P of two circles and if TA and TB are respectively the other tangents at A and B to the two circles drawn from the point T then
A $T A = 2 T B$
B $T A = T B$
C $T A = \frac{1}{2} T B$
D $3 T A = T B$

IIT JEE PREVIOUS YEAR-SOLVED PAPER 2019-Paper -1 section-3

Home
Ask Doubt
Profile