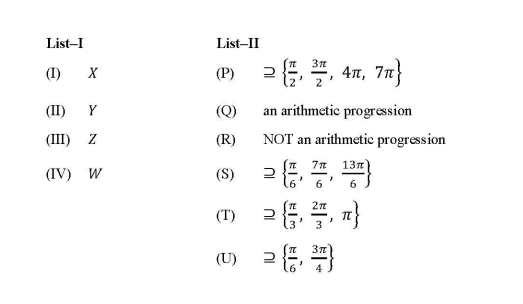
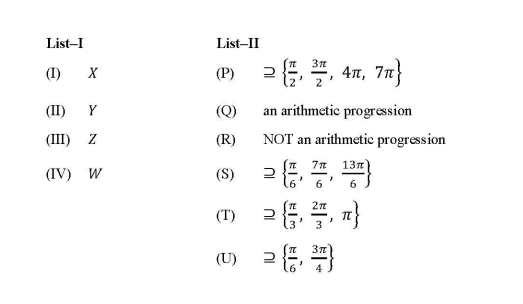
Let $f (x) = sin (π cos x) and g (x) = cos (2 π sin x)$ be two finctions defined for $x > 0$ . Define the following sets whose elements are written in the increasing order :
$X = {x : f (x) = 0}, Y = {f' (x) = 0}$
$Z = {x : g (x) = 0}, W = {g' (x) = 0}$
List -I contains the sets X,Y,Z and W. List -II contains some information regarding these sets.

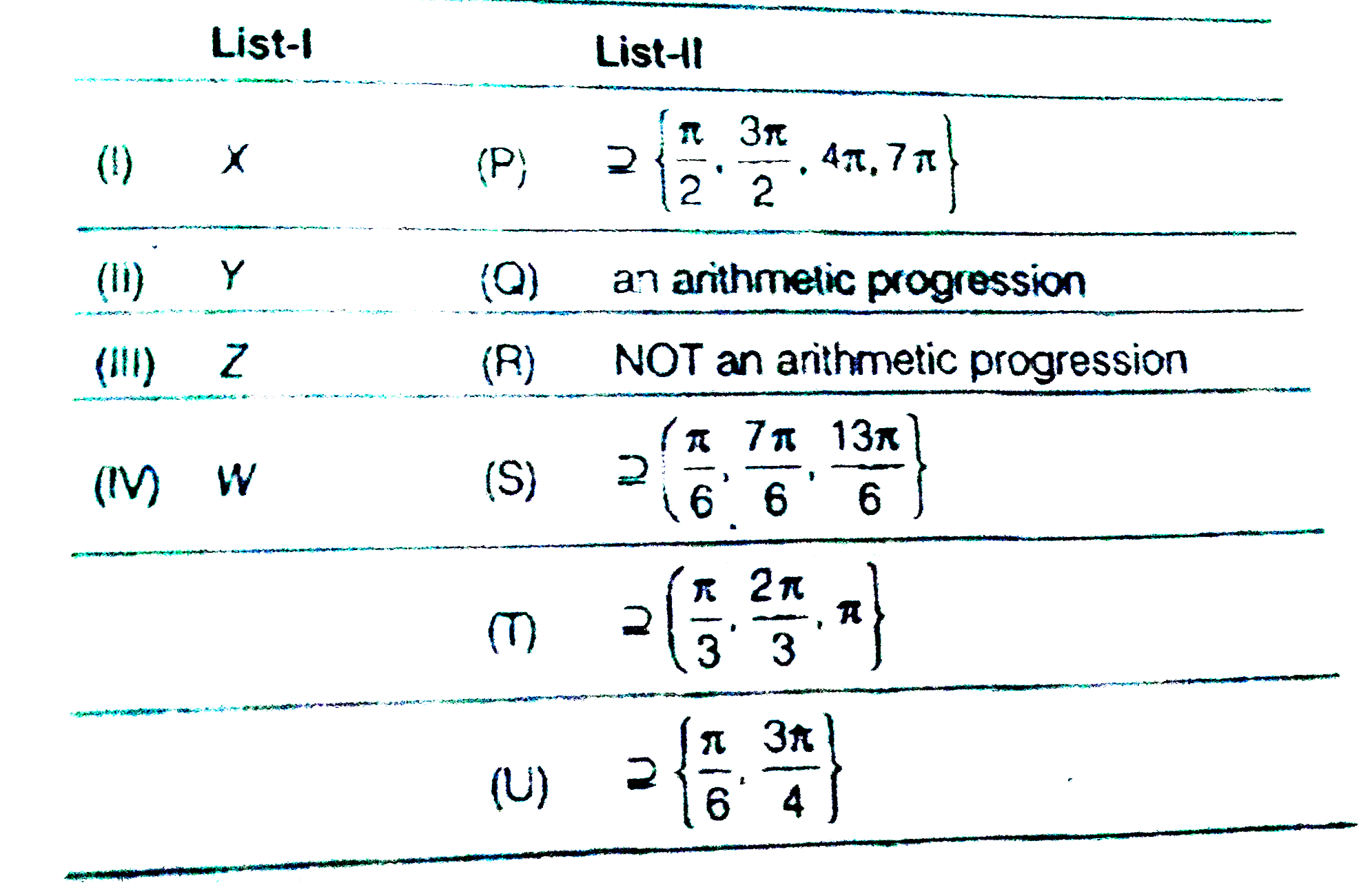
Which of the following is the only CORRET combination ?

Text Solution

Verified by Experts

The correct Answer is:A

For $X = {x : f (x) = 0}, x > 0$
Now, $f (x) = 0$
$\Rightarrow sin (π cos x) = 0, x > 0$
$\Rightarrow cos x = n π, n \in$ Integer.
$\Rightarrow cos x n$
$\Rightarrow cos x = - 1, 0, 1 {∵ cos x \in [- 1, 1]}$
$\Rightarrow x = n π or (2 n + 1) \frac{π}{2}, n$ is a integer so, (i) $\to (P), (Q)$
For, $Y = {x : f' (x) > 0$
Now, $f' (x) = 0$
$\Rightarrow - π sin x cos (π cos x) = 0$
$\Rightarrow$ either $sin x = 0 n π, n$ is an integer , or $cos (π cos x) = 0$
$\Rightarrow π cos x = (2 n + 1) \frac{π}{2}, n$ is an integer.
$\Rightarrow cos x = \frac{2 n + 1}{2}$
$\Rightarrow cos x = \pm \frac{1}{2} {∵ cos x \in [- 1, 1]}$
$\Rightarrow x = 2 n π \pm \frac{2 π}{3}, n$ is an integer.
So, (ii) $\to (Q), (T)$
Hence, option (a) is correct.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Knowledge Check

Answer the following by appropriately matching the lists based on the information given in the paragraph.
Let $f (x) = sin (π cos x)$ and $g (x) = cos (2 π sin x)$ be two functions defined for $x > 0$ . Define the following sets whose elements are written in the increasing order:
$X = {x : f (x) = 0}, Y = {x : f^{'} (x) = 0}, Z = {x : g (x) = 0}, W = {x : g^{'} (x) = 0}$
List-I contains the sets $X, Y, Z$ and W. List-II contains such some information regarding these sets:

Which of the following is the only CORRECT combinations?
A(II),(R ), (S)
B(I), (P), (R )
C(II), (Q), (T)
D(I), (Q), (U)
Answer the following by appropriately matching the lists based on the information given in the paragraph.
Let $f (x) = sin (π cos x)$ and $g (x) = cos (2 π sin x)$ be two functins defined for $x > 0$ . Define the following sets whose elements are written in the increasing order:
$X = {x : f (x) = 0}, Y = {x : f^{'} (x) = 0}, Z = {x : g (x) = 0}, W = {x : g^{'} (x) = 0}$
List-I contains the sets $X, Y, Z$ and W. List-II contains such some information regarding these sets:

Which of the following is the only CORRECT combinations?
A(IV),(Q),(T)
B(IV),(P),(R ),(S)
C(III),( R),(U)
D(III),(P), (Q),(U)
Let f(x)= $sin (π cos x)$ and g(x)= $cos (1 π sin x)$ e two function defined for $x > 0$ define the following sets whose elements are written in increasing order.
$X = {x : f (x) = 0}, Y = {x : f' (x) = 0}$
$Z = {x : g (x) = 0}, W = {x : g' (x) = 0}$
$\begin{matrix} List I & List II (I) X & (P) \supseteq {\frac{π}{2}, \frac{3 π}{2}, 4 π, 7 π} (I I) Y & (Q) an arithmetic progression (I I I) Z & (R) not an arithmetic progression (I V) W & (S) \supseteq {\frac{π}{6}, \frac{7 π}{6}, \frac{13 π}{6}} (T) \supseteq {\frac{π}{3}, \frac{2 π}{3}, π} (U) \supseteq {\frac{π}{6}, \frac{3 π}{4}} \end{matrix}$
Q. Which of th following is the only correct combination
AIV-(P),(R),(S)
BIII-(R),(U)
CIII-(P),(Q),(U)
DIV-(Q),(T)

IIT JEE PREVIOUS YEAR-SOLVED PAPER 2019-Paper -2 section-3

Home
Ask Doubt
Profile

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

IIT JEE PREVIOUS YEAR-SOLVED PAPER 2019-Paper -2 section-3