Let the circles G1:x2+y2=9 and C1:(x−3)2+(y−4)2=16, intersect at the point X and Y . Suppose that another circle.
C3:(x−h)2+(y−k)2=r2 satisfies the following conditions :
(i) Centre of C3 is collinear with the centres of C1 and C2.
(ii) C1andC2 both lie inside C3 and
(iii) C3 touches C1 and M and C2 and N.
Let the line through X and Y intersect C3 at Z and W, and let a common tangent of C1 and C3 be a tangent to the parabola x2=8ay.
There are some expression given in the List -I whose values are given in List -II below.
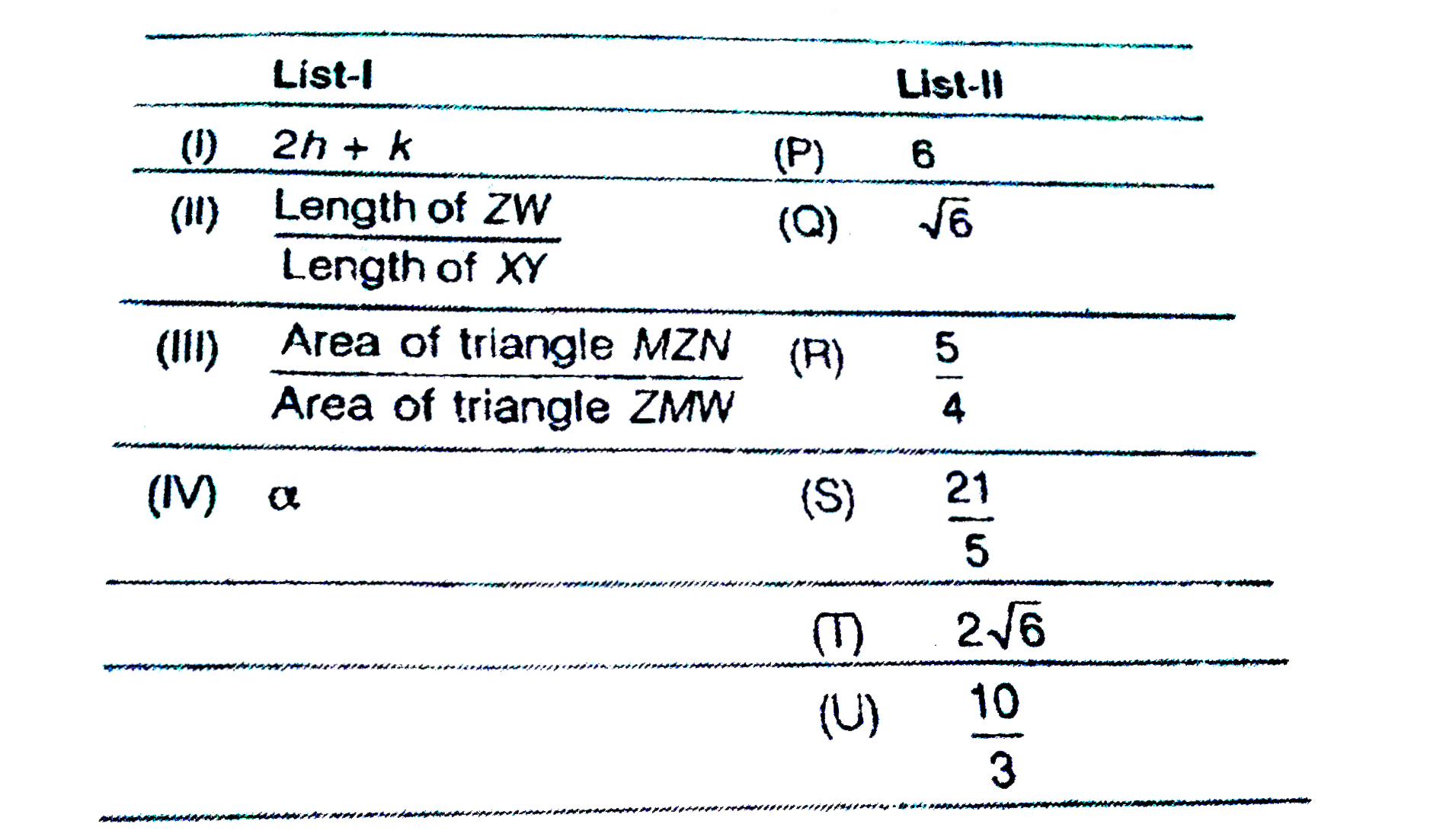
Which of the following is the only INCORRECT combination ?
(II),(T)
(I),(S)
(II),(Q)
(I),(U)
The correct Answer is:C
∵length of ZWlength of XY=√6
So, combination (ii), Q is only correct.
Hence, option (c) is correct.
Topper's Solved these Questions
- SOLVED PAPER 2019BOOK - IIT JEE PREVIOUS YEARCHAPTER - SOLVED PAPER 2019EXERCISE - Paper -2 section-2
6 Videos
- SEQUENCES AND SERIESBOOK - IIT JEE PREVIOUS YEARCHAPTER - SEQUENCES AND SERIESEXERCISE - RELATION BETWEEN AM,GM, HM AND SOME SPECIAL SERIES
34 Videos
- STRAIGHT LINE AND PAIR OF STRAIGHT LINESBOOK - IIT JEE PREVIOUS YEARCHAPTER - STRAIGHT LINE AND PAIR OF STRAIGHT LINESEXERCISE - GENERAL EQUATION OF PAIR OF STRAIGHT LINES (Objective Questions I) (Only one correct option)
1 Videos
Similar Questions
Knowledge Check
Let the circle C1:x2+y2=9 and C2:(x−3)2+(y−4)2=16 intersect at the point X and Y. Suppose that another circle C3:(x−h)2+(y−k)2=r2 satisfies the following conditions
(i). Centre of C3 is collinear with the center of C1&C2
(ii). C1&C2 both lie inside C3 and
(iii). C3 touches C1 at M and C2 at N
Let hte line through X and Y intersect C3 at Z and W and let a common tangent of C1 & C3 be a tangent to the parabola x2=8αy
There are some expressions given in the following lists
List IList II(I) 2h+k(P) 6(II) length of ZWlength of XY(Q) √6(III) Area of ΔMZNArea of ΔZMW(R) 54(IV) α(S) 215(T) 2√6(U) 103
Q. Which of the following is the only correct combination?
(A) (I)-(S)
(B) (II)-(Q) ltBrgt (C) (I)-(U)
(D) (II)-(T)A(I)-(S)B(II)-(Q)C(I)-(U)D(II)-(T)Let the circle C1:x2+y2=9 and C2:(x−3)2+(y−4)2=16 intersect at the point X and Y. Suppose that another circle C3:(x−h)2+(y−k)2=r2 satisfies the following conditions
(i). Centre of C3 is collinear with the center of C1&C2
(ii). C1&C2 both lie inside C3 and
(iii). C3 touches C1 at M and C2 at N
Let hte line through X and Y intersect C3 at Z and W and let a common tangent of C1 & C3 be a tangent to the parabola x2=8αy
There are some expressions given in the following lists
List IList II(I) 2h+k(P) 6(II) length of ZWlength of XY(Q) √6(III) Area of ΔMZNArea of ΔZMW(R) 54(IV) α(S) 215(T) 2√6(U) 103
Q. Which of the following is the only incorrect combination?A(IV)-(U)B(III)-(R)C(IV)-(S)D(I)-(P)If the circles (x−3)2+(y−4)4=16 and (x−7)2+y−7)2=9 intersect at points A and B, then the area (in sq. units) of the quadrilateral C1AC2B is equal to (where, C1andC2 are centres of the given circles)
A6B12C18D24
A circle C1 passes through the centre of the circle C2;x2+y2=4 and gets bisected at its point of intersection with C2 .The radius of c1 is equal to:
View SolutionA circle C touches the line x = 2y at the point (2, 1) and intersects the circle C1:x2+y2+2y−5= 0 at two points P and Q such that PQ is a diameter of C1. Then the diameter of C is :
View SolutionConsider two curves C1:y2=4x ; C2=x2+y2−6x+1=0. Then, a. C1 and C2 touch each other at one point b. C1 and C2 touch each other exactly at two point c. C1 and C2 intersect(but do not touch) at exactly two point d. C1 and C2 neither intersect nor touch each other
View SolutionLet C1:y=x2sin3x,C2:y=x2andC3:y=−y2, then
View SolutionIf two diameters of the circle 3x2+3y2−6x−18y−7=0 are along the lines 3x+y=c1 and x−3y=c2. Then, the value of c1c2 is
View Solution
