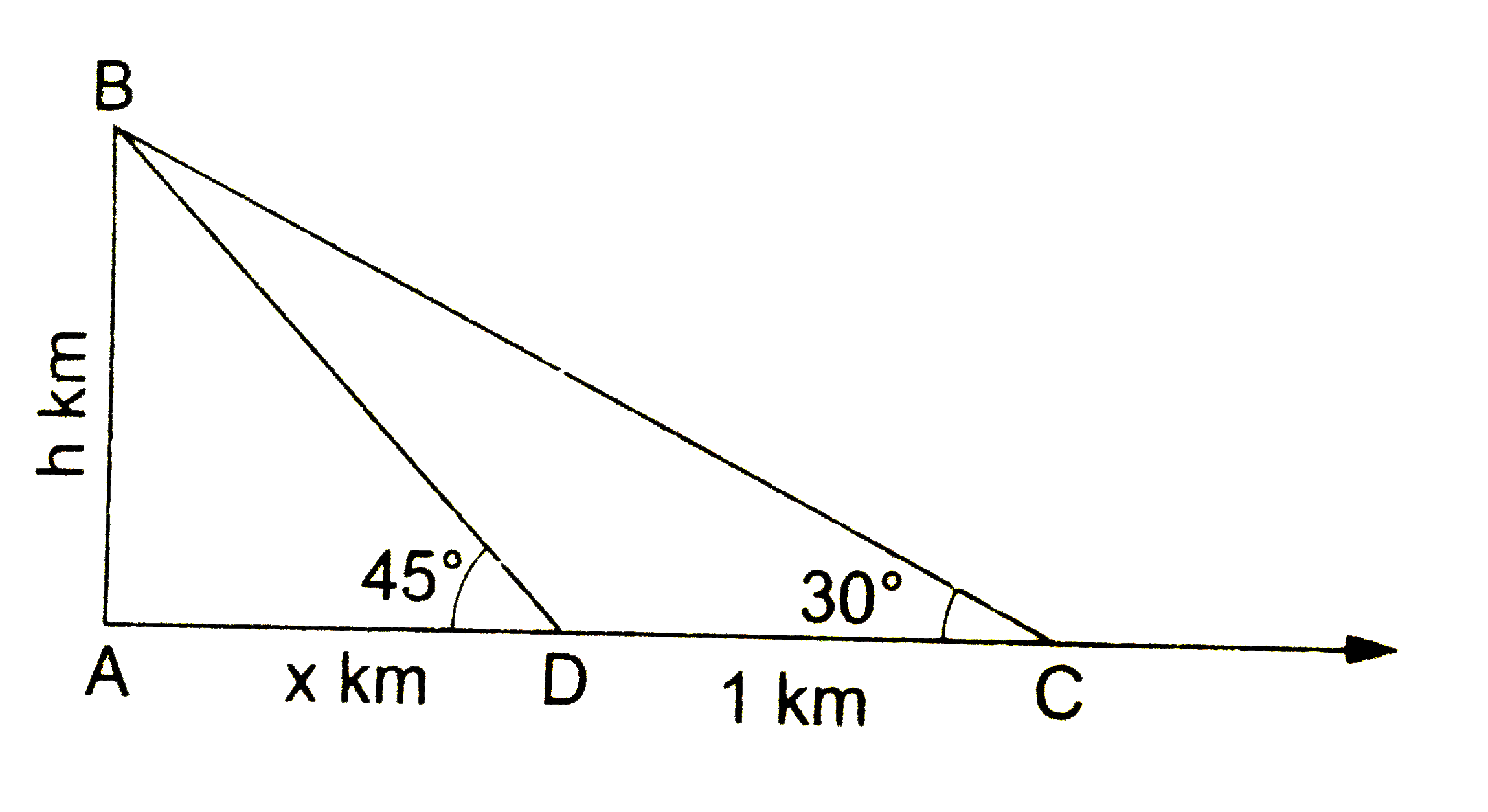
From the top of a hill, the angles of depression of two consecutive kilometre stones due east are found to be 30∘ and 45∘. Find the height of the hill.
12(√3−1)km
12(√3+1)km
(√3−1)km
(√3+1)km
The correct Answer is:B
Let AB be the hill.
From right ΔBAD, we have
ADAB=cot45∘⇒xh=1⇒x=h…….(i)
From right ΔBAC, we have
ACAD=cot30∘⇒x+1h=√3⇒x=(h√3−1)………(ii)
From (i) and (ii), we have
h=(h√3−1)⇒h(√3−1)=1
⇒h=1(√3−1)
⇒h=⎧⎪
⎪⎨⎪
⎪⎩1(√3−1)×(√3+1)(√3+1)⎫⎪
⎪⎬⎪
⎪⎭=12(√3+1).
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - HEIGHTS AND DISTANCESEXERCISE - Exercise 1438videos
HEIGHTS AND DISTANCES
- BOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)34videos
COORDINATE GEOMETRY
- BOOK - RS AGGARWALCHAPTER - LINEAR EQUATIONS IN TWO VARIABLES EXERCISE - QUESTION2videos
LINEAR EQUATIONS IN TWO VARIABLES
Similar Questions
From the top of the hill; the angle of depressions of two consecutive kilometre stones due east are found to be 30∘ and 45∘ . Find the height of the hill.
View SolutionFrom the top of a building 60m high the angles of depression of the top and the bottom of a tower are observed to be 300and600 . Find the height of the tower.
View Solution
Knowledge Check
- Question 1 - Select One
From the top of a cliff 50 m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘. The height of tower is
A50mB50√3mC50(√3−1)mD50(1−√33)m - Question 2 - Select One
From the top of a cliff 50m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘ The height of tower is
A50 mB50√3C50(√3−1)D50(1−√33)m - Question 3 - Select One
From the top of a cliff 50m high, the angles of depression of the top and bottom of a tower are observed to be 30∘ and 45∘ The height of tower is
A50 mB50√3C50(√3−1)D50(1−√33)m
From the top of a building 60m high the angles of depression of the top and the bottom of a tower are observed to be 30o and 60o . Find the height of the tower.
View SolutionFrom the top of a cliff, 200m high, the angle of depression of the top and bottom of a tower are observed to be 30∘ and 60∘, find the height of the tower.
View SolutionFrom an aeroplane vertically over a straight horizontal road, the angles of depression of two consecutive kilometre-stones on the opposite sides of the aeroplane are observed to be αandβ. The height of the aeroplane above the road is
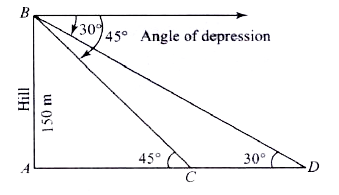
View SolutionThe height of a hill is 150 m. From the top of the hill the angles of depression of two objects lying towards east to the hill are 45∘ and 30∘. Find the distance btween the objects.
 View Solution
View SolutionFrom an aeroplane just over a straight road, the angles of depression of two consecutive kilometre is stones situated at opposite sides of the aeroplane were found to be 60∘ and 30∘ respectively. The height (in km) of the aerophlane from the road at that instant was
(Given √3=1.732)View Solution

