Sides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Prove that ΔABC~DetlaPQR
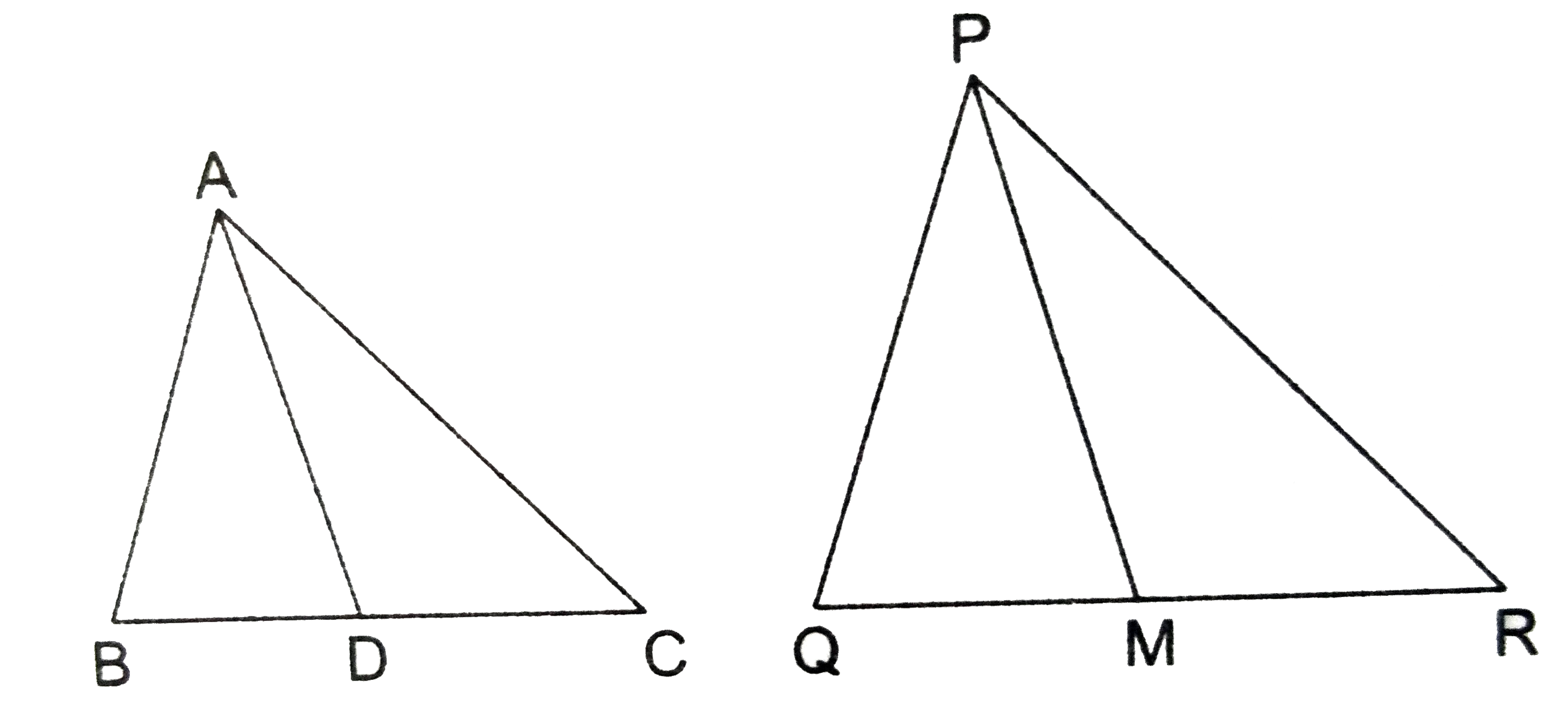
GIVEN AD and PM ar medians of ΔABCandΔPQR respectively such that
ABPQ=ACPR=ADPM
TO PROVE ΔAB~ΔPQR
CONSTRUCTION Produce AD to E such that AD= DE and produce PM to N such that PM= MN.
Join EC and NR.
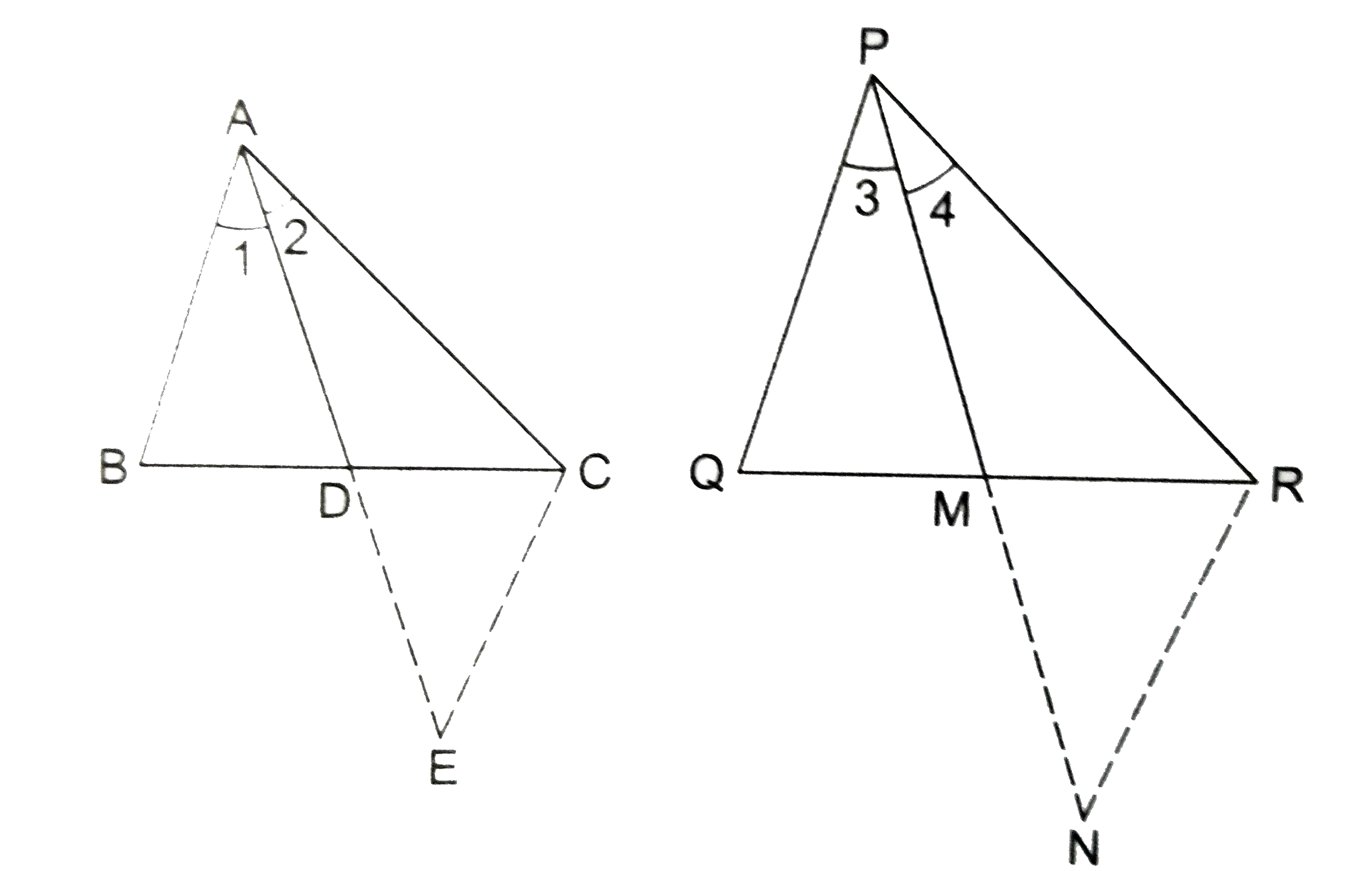
PROOF In ΔABDandΔECD, we have
BD=CD[∴D is midpoint of BC]
AD= ED [ by construction]
∠BDA=∠CDE [ vertices opposite angels]
∴ΔABD≅ΔECD [ by SAS- contruency]
And so, AB=EC ....(i) [cpct]
Similarly, ΔPQM≅ΔNRM
and So, PQ=NR ....(ii) [ cpct]
Now, ABPQ=ACPR=ADPM (given)
⇒ECNR=ACPR=ADPM [ using (i) and (ii)]
⇒ECNR=ACPR=2AD2PM=AEPN[∴2AD=AE,2PM=PN]
⇒ΔACE~ΔPNR [ SSS- similarity]
∴∠2=∠4 [ corresponding angels of similar triangles are equal]
Similarly, ∠1=∠3= [ it can be proved by joining BE and QN and showing ΔABE~ΔPQN]
∴∠1+∠2=∠3+∠4,i.e.,∠BAC=∠QPR. ....(iii)
Now, in ΔABCandΔPQR, we have
ABPQ=ACPR [ given]
∠BACE=∠QPR [ form (ii)]
∴ΔABCD~ΔPQR[ by SAS-similarity]
Topper's Solved these Questions
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLE
4 Videos
- TRIANGLESBOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - EXERCISE 7 A
1 Videos
- T-RATIOS OF SOME PARTICULAR ANGLESBOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 11
27 Videos
- TRIGONOMETRIC IDENTITIESBOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself
20 Videos
Similar Questions
Sides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Show that ΔABC~ΔPQR.
View SolutionSides AB and BC and median AD of a triangle ABC are respectively proportional to sides PQ and QR and median PM of ΔPQR. Show that ΔABC~ΔPQR.
View Solution
Knowledge Check
If PS is median of the triangle PQR, then ar(ΔPQS): ar(ΔQRP) is
 A1 : 1B2 : 1C1 : 2DCan't be determined
A1 : 1B2 : 1C1 : 2DCan't be determinedPoints P and Q lie on sides AB and AC of triangle ABC respectively such that segment PQ is parallel to side BC. If the ratio of areas of triangle APQ: triangle ABC is 25 : 36, then the ratio PA : PB is
A5:6B1:5C6:5D5:1
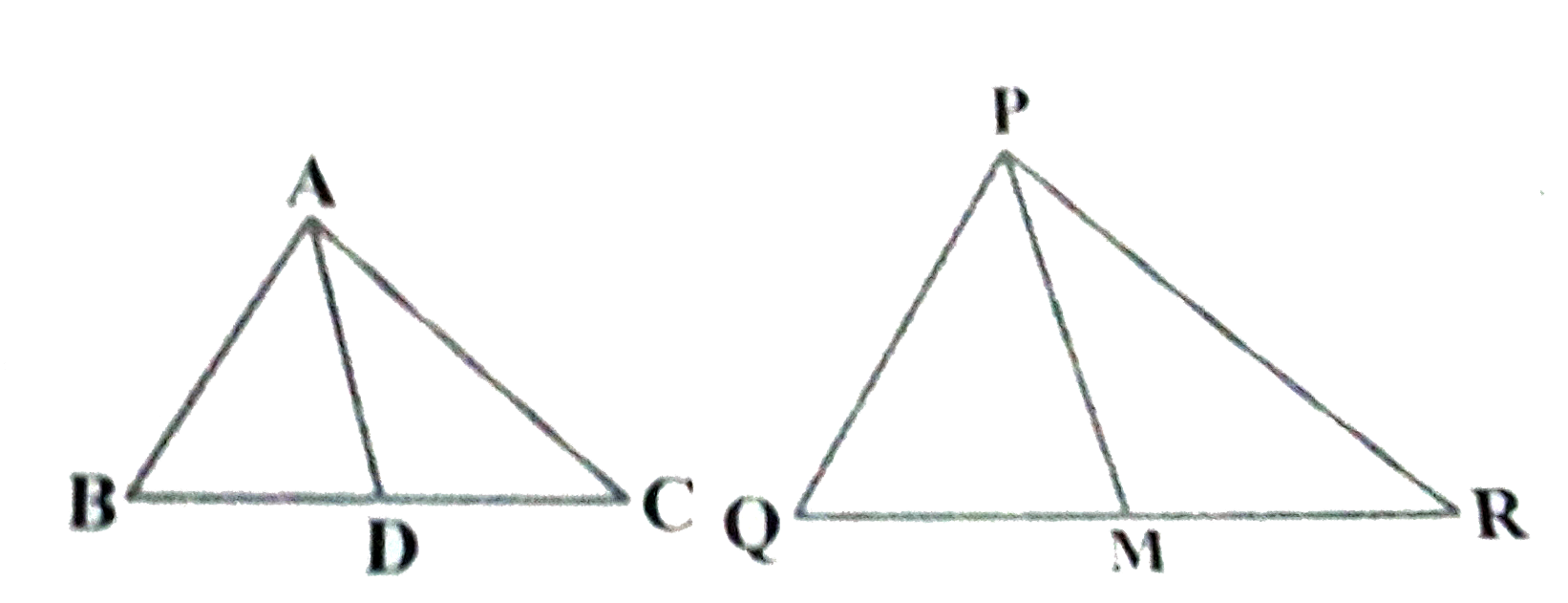
Sides AB and BC and median AD of a triangle ABC are respectively propor-/ tional to sides PQ and QR and median PM of ΔPQR (see Fig. 6.41). Show that ΔABC~ΔPOR.
View SolutionTwo sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of ΔPQR (see figure). Show that

ΔABM≅ΔPQN
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionThe sides AB and BC and the median AD of triangle ABC are equal to the sides PQ and QR and the median PM of triangle PQR respectively. Prove that the triangles ABC and PQR are congruent.
View SolutionIf two sides and a median bisecting one of these sides of a triangle are respectively proportional to the two sides and corresponding median of another triangle; then triangle are similar.
View SolutionIn Figure, ABC is an isosceles triangle with AB=AC, BD and CE are two medians of the triangle. Prove that BD=CE
View Solution
