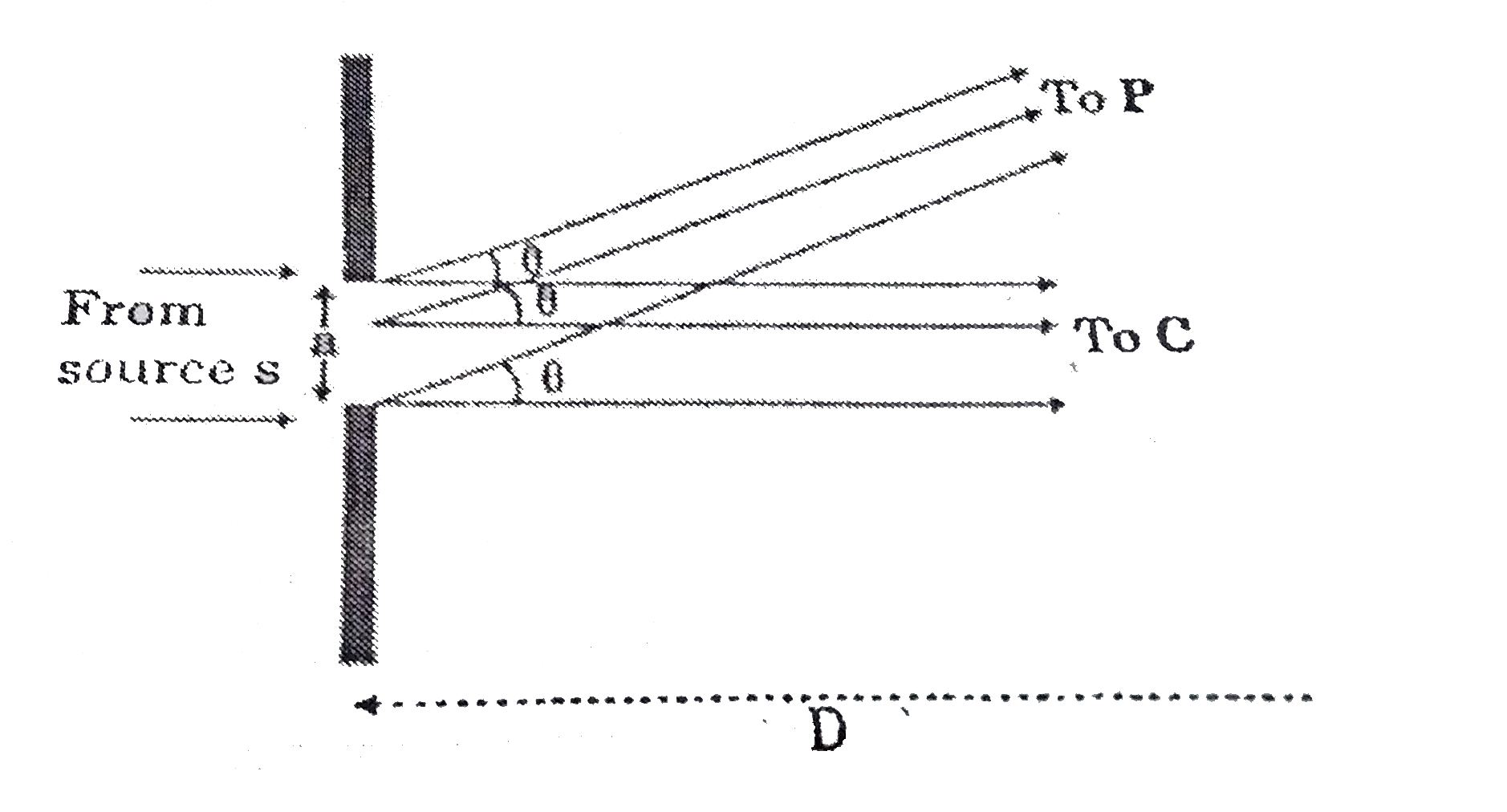
$The figure, drawn here, show sthe geometry of path difference for diffraction by a single-slit of width 'a'. Give appropriate reasoning to explain why the intensity of light is : (a) maximum at the central point C on the screen. (b) nearly zero for point P on the screen when theta=(lamda)/(a). Hence, write an expression for the total linearm width of the central maximum on a screen kept at a distance 'D' from the plane of the slit.$
The figure, drawn here, show sthe geometry of path difference for diffraction by a single-slit of width 'a'. Give appropriate reasoning to explain why the intensity of light is :
(a) maximum at the central point C on the screen.
(b) nearly zero for point P on the screen when $θ = \frac{λ}{a}$ .
Hence, write an expression for the total linearm width of the central maximum on a screen kept at a distance 'D' from the plane of the slit.

Text Solution

Verified by Experts

Let a plane wavefront is coming from a source and is incident on a slit AB of width .a. parallel to it. In accordance with Huygen.s principle, secondary wavelents pass through the slit in different directions.

(a) For wavelets travelling straight towards the central point C on the screen, there is no path difference between various wavelets and so all of them reach in same phase. as a result, the intensity o flight at central point C is maximum.
(b) For wavelets diffracting at an angle $θ$ , the path difference between the extreme wavelets starting from the ends A and B of slit is:
Path difference =BD=AD $sin θ = a sin θ$
If this path difference equals to $λ$ , then the entire wavefront may be considered as a combination of two half parts such that wavelets from corresponding points on these halves differ in path by $\frac{λ}{2}$ and are thus in mutually opposite phase and nullify each other. so resultant intensity at point P on the screen is practicaly zero at an angle $θ$ , such that
$a sin θ = λ or s i θ = \frac{λ}{a}$
if $θ$ is small then we have $sin θ = θ = \frac{λ}{a}$ for zero intensity at point P.
From the above it is clear that the central diffraction maximum on the screen extends from
$θ_{1} = - \frac{λ}{a}$ to $θ_{2} = + \frac{λ}{a}$ and has an angular width of $α = \frac{2 λ}{a}$ .
If the screen is placed at a distance .D. from the plane of the slit, then linear width of central diffraction maximum.
$β = D α = D \cdot \frac{2 λ}{a} = \frac{2 D λ}{a}$ .

Updated on:21/07/2023