What is the percentage of persons who read only two papers?
19%
31%
44%
None of the above
The correct Answer is:A
To find the percentage of persons who read only two papers, we need to analyze the given data regarding the percentages of individuals reading each paper and the overlaps between them.
Let's denote:
- A = percentage of persons reading paper A = 42%
- B = percentage of persons reading paper B = 51%
- C = percentage of persons reading paper C = 68%
We will also need to know the percentage of persons who read all three papers (let's denote this as x). However, since the video transcript does not provide the exact value of x or the overlaps, we will assume that we have this information.
Step 1: Use the principle of inclusion-exclusion
To find the percentage of persons who read only two papers, we can use the principle of inclusion-exclusion. The formula for three sets is:
|A∪B∪C|=|A|+|B|+|C|−|A∩B|−|A∩C|−|B∩C|+|A∩B∩C|
Step 2: Define the overlaps
Let:
- |A∩B| = percentage of persons reading both A and B
- |A∩C| = percentage of persons reading both A and C
- |B∩C| = percentage of persons reading both B and C
- |A∩B∩C|=x (percentage of persons reading all three papers)
Step 3: Calculate the percentage of persons reading only two papers
The percentage of persons reading only two papers can be calculated as follows:
Only A and B=|A∩B|−|A∩B∩C|
Only A and C=|A∩C|−|A∩B∩C|
Only B and C=|B∩C|−|A∩B∩C|
Thus, the total percentage of persons reading only two papers is:
Only two papers=(|A∩B|−x)+(|A∩C|−x)+(|B∩C|−x)
Step 4: Substitute known values
Now, if we had the values for |A∩B|, |A∩C|, and |B∩C|, we could substitute them into the equation to find the total percentage of persons reading only two papers.
Step 5: Calculate the percentage
Finally, we would express the result as a percentage of the total population surveyed.
- A = percentage of persons reading paper A = 42%
- B = percentage of persons reading paper B = 51%
- C = percentage of persons reading paper C = 68%
To find the percentage of persons who read only two papers, we can use the principle of inclusion-exclusion. The formula for three sets is:
Let:
- |A∩B| = percentage of persons reading both A and B
- |A∩C| = percentage of persons reading both A and C
- |B∩C| = percentage of persons reading both B and C
- |A∩B∩C|=x (percentage of persons reading all three papers)
The percentage of persons reading only two papers can be calculated as follows:
Only A and C=|A∩C|−|A∩B∩C|
Only B and C=|B∩C|−|A∩B∩C|
Now, if we had the values for |A∩B|, |A∩C|, and |B∩C|, we could substitute them into the equation to find the total percentage of persons reading only two papers.
Finally, we would express the result as a percentage of the total population surveyed.
Topper's Solved these Questions
Similar Questions
In a city, three daily newspapers A,B,C are published. 42% of the people in that city read A, 51% read B and 68% read C. 30% read A and B, 28% read B and C ,36%read A and C. 8% do not read any of the three newspapers. The percentage of persons who read all the three papers is
View SolutionIn a certain town of population size 1,00,000 three types of newspapers (f, II and Ill) are available. The percentages of' the people in the town who read these papers are as follows :
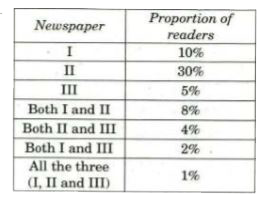
What is the number of people who read only one newspaper ?
View Solution
Knowledge Check
What is the percentage of persons who read only one paper?
A38%B48%C51%DNone of the aboveIn a city, three daily newspapers A, B, C are published, 42% read A, 51% read B, 68% read C, 30% read A and B, 28% read Band C 36% read A and C, 8% do not read any of the three newspapers.
What is the percentage of persons who read only one paper?A0.38B0.48C0.51DNone of theseWhat is the percentage of persons who read only A but neither B nor C?
A4%B3%C1%DNone of the above
For the next Four (21-24) questions that follow:
In a city, three daily newspapers A, B, C are published, 42% read A, 51% read B, 68% read C, 30% read A and B, 28% read Band C 36% read A and C, 8% do not read any of the three newspapers.
What is the percentage of persons who read only A but neither B nor C?
View SolutionWhat is the percentage of persons who read all the three papers?
View SolutionFor the next Four (21-24) questions that follow:
In a city, three daily newspapers A, B, C are published, 42% read A, 51% read B, 68% read C, 30% read A and B, 28% read Band C 36% read A and C, 8% do not read any of the three newspapers.
What is the percentage of persons who read all the three papers?
View SolutionIn a office ,the ratio of the percentages of employees who like only tea, percentage of employees who like only coffee ,perscentage of employees who like neither of the drinks is 8 : 7: 6: 4 Find the percentage of employees who like neither of the deinks.
View SolutionIn a office ,the ratio of the percentages of employees who like only tea, percentage of employees who like only coffee ,perscentage of employees who like neither of the drinks is 8 : 7: 6: 4 Find the percentage of employees who like neither of the deinks.
View Solution