What is the percentage of persons who read only one paper?
38%
48%
51%
None of the above
The correct Answer is:B
To solve the problem of finding the percentage of persons who read only one newspaper among three newspapers A, B, and C, we can use the principle of inclusion-exclusion.
1. Identify the given data:
- Percentage of people who read newspaper A (P(A)) = 42%
- Percentage of people who read newspaper B (P(B)) = 51%
- Percentage of people who read newspaper C (P(C)) = 68%
- Percentage of people who read both A and B (P(A ∩ B)) = 30%
- Percentage of people who read both B and C (P(B ∩ C)) = 28%
- Percentage of people who read both A and C (P(A ∩ C)) = 36%
- Percentage of people who do not read any newspaper = 8%
2. Calculate the percentage of people who read at least one newspaper:
P(At least one)=100%−P(None)=100%−8%=92%
3. Use the principle of inclusion-exclusion:
The formula for the union of three sets is:
P(A∪B∪C)=P(A)+P(B)+P(C)−P(A∩B)−P(B∩C)−P(A∩C)+P(A∩B∩C)
Substituting the known values:
92%=42%+51%+68%−30%−28%−36%+P(A∩B∩C)
Simplifying the equation:
92%=42+51+68−30−28−36+P(A∩B∩C)
92=42+51+68−30−28−36+P(A∩B∩C)
92=42+51+68−94+P(A∩B∩C)
92=67+P(A∩B∩C)
P(A∩B∩C)=92−67=25%
4. Calculate the percentage of people who read only one newspaper:
- Percentage of people who read only A:
P(Only A)=P(A)−P(A∩B)−P(A∩C)+P(A∩B∩C)
P(Only A)=42%−30%−36%+25%=1%
- Percentage of people who read only B:
P(Only B)=P(B)−P(A∩B)−P(B∩C)+P(A∩B∩C)
P(Only B)=51%−30%−28%+25%=−2%(not possible, so it means some overlap)
- Percentage of people who read only C:
P(Only C)=P(C)−P(A∩C)−P(B∩C)+P(A∩B∩C)
P(Only C)=68%−36%−28%+25%=29%
5. Total percentage of people who read only one newspaper:
P(Only A)+P(Only B)+P(Only C)=1%+(−2%)+29%=28%
6. Final answer:
The percentage of persons who read only one paper is 28%.
- Percentage of people who read newspaper A (P(A)) = 42%
- Percentage of people who read newspaper B (P(B)) = 51%
- Percentage of people who read newspaper C (P(C)) = 68%
- Percentage of people who read both A and B (P(A ∩ B)) = 30%
- Percentage of people who read both B and C (P(B ∩ C)) = 28%
- Percentage of people who read both A and C (P(A ∩ C)) = 36%
- Percentage of people who do not read any newspaper = 8%
P(At least one)=100%−P(None)=100%−8%=92%
The formula for the union of three sets is:
P(A∪B∪C)=P(A)+P(B)+P(C)−P(A∩B)−P(B∩C)−P(A∩C)+P(A∩B∩C)
Substituting the known values:
92%=42%+51%+68%−30%−28%−36%+P(A∩B∩C)
Simplifying the equation:
92%=42+51+68−30−28−36+P(A∩B∩C)
92=42+51+68−30−28−36+P(A∩B∩C)
92=42+51+68−94+P(A∩B∩C)
92=67+P(A∩B∩C)
P(A∩B∩C)=92−67=25%
- Percentage of people who read only A:
P(Only A)=P(A)−P(A∩B)−P(A∩C)+P(A∩B∩C)
P(Only A)=42%−30%−36%+25%=1%
P(Only B)=P(B)−P(A∩B)−P(B∩C)+P(A∩B∩C)
P(Only B)=51%−30%−28%+25%=−2%(not possible, so it means some overlap)
P(Only C)=P(C)−P(A∩C)−P(B∩C)+P(A∩B∩C)
P(Only C)=68%−36%−28%+25%=29%
P(Only A)+P(Only B)+P(Only C)=1%+(−2%)+29%=28%
The percentage of persons who read only one paper is 28%.
Topper's Solved these Questions
Similar Questions
In a certain town of population size 1,00,000 three types of newspapers (f, II and Ill) are available. The percentages of' the people in the town who read these papers are as follows :
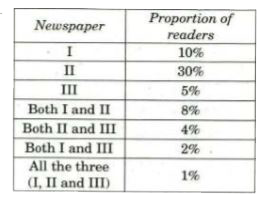
What is the number of people who read only one newspaper ?
View SolutionIn a city, three daily newspapers A,B,C are published. 42% of the people in that city read A, 51% read B and 68% read C. 30% read A and B, 28% read B and C ,36%read A and C. 8% do not read any of the three newspapers. The percentage of persons who read all the three papers is
View Solution
Knowledge Check
What is the percentage of persons who read only two papers?
A19%B31%C44%DNone of the aboveWhat is the percentage of persons who read only A but neither B nor C?
A4%B3%C1%DNone of the aboveFor the next Four (21-24) questions that follow:
In a city, three daily newspapers A, B, C are published, 42% read A, 51% read B, 68% read C, 30% read A and B, 28% read Band C 36% read A and C, 8% do not read any of the three newspapers.
What is the percentage of persons who read only A but neither B nor C?AA) 4%BB) 3%CC) 1%DD) None of these
In a certain city only two newspapers A and B are published, it is known that 25% of the city population reads A and 20% reads B, while 8% reads both A and B. It is also known that 30% of those who read A but not B look int advertisements and 40% of those who read B bu not A look into advertisements while 50% of those who read both A and B look into advertisements What is the percentage of the population reads an advertisement? [1984]
View SolutionWhat is the percentage of persons who read all the three papers?
View SolutionFor the next Four (21-24) questions that follow:
In a city, three daily newspapers A, B, C are published, 42% read A, 51% read B, 68% read C, 30% read A and B, 28% read Band C 36% read A and C, 8% do not read any of the three newspapers.
What is the percentage of persons who read all the three papers?
View SolutionIn a office ,the ratio of the percentages of employees who like only tea, percentage of employees who like only coffee ,perscentage of employees who like neither of the drinks is 8 : 7: 6: 4 Find the percentage of employees who like neither of the deinks.
View SolutionIn a office ,the ratio of the percentages of employees who like only tea, percentage of employees who like only coffee ,perscentage of employees who like neither of the drinks is 8 : 7: 6: 4 Find the percentage of employees who like neither of the deinks.
View Solution