Question

For each non-zero real number x, let $f\left(x\right)=\frac{x}{\left|x\right|}$. The range of f is

Options

A. a null set
B. a set consisting of only one element
C. a set consisting of two elements
D. a set consisting of infinitely many elements

Answer

C

Explanation

For a non-zero real number x
`f(x)=(x)/(|x|)`
domain `f(x)=(x)/(|x|)` range

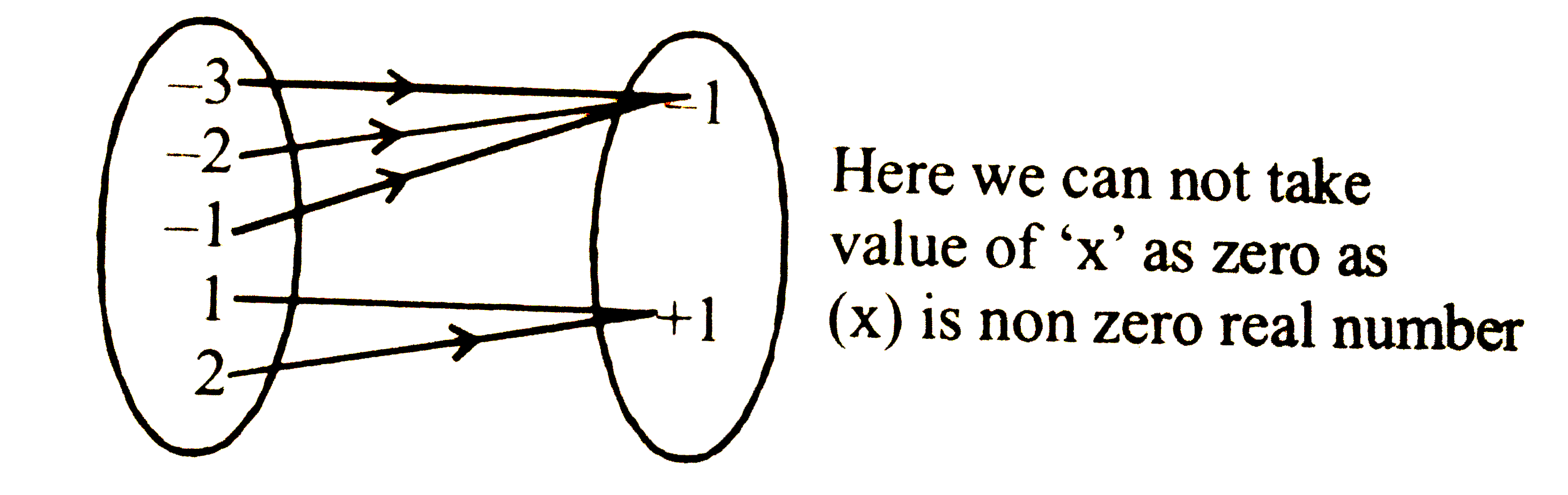
for any value of x in domain a set consisting of two elements i.e. (-1, +1)
`therefore` Option (c ) is correct.