Let Sk,k=1,2,...,100, denote the sum of the infinite geometric series whose first term is k−1k! and the common ratio 1k. Then the value of 1002100!+100∑k=1∣∣(k2−3k+1)Sk∣∣, is
3
6
8
9
The correct Answer is:A
We have,
Sk=k−1k!1−1k=1(k−1)!
∴ 100∑k=2∣∣(k2−3k+1)Sk∣∣
=100∑k=2∣∣
∣
∣∣{(k−1)2−k}(k−1)!∣∣
∣
∣∣
=100∑k=2∣∣∣k−1(k−2)!−k(k−1)!∣∣∣
=100∑k=2∣∣∣(k−2)+1(k−2)!−(k−1)+1(k−1)!∣∣∣
=100∑k=2∣∣∣1(k−3)!−1(k−2)!+1(k−2)!−1(k−2)!∣∣∣
=100∑k=2{1(k−3)!−1(k−2)!}100∑k=2{1(k−2)!−1(k−1)!}
={(1−11!)+(11!−12!)+(12!−13!)+...+(197!−198!)}
+{(1−11!)+(11!−12!)+(12!−13!)+...+(198!−199!)}
=(1−198!)+(1−199!)=2198!−199!=2−10099!
Hence,
(100)2100!+100∑k=1∣∣(k2−3k+1)Sk∣∣
10099!+S1+100∑k=2∣∣(k2−3k+1)Sk∣∣
=10099!+1+2−10099!=3
Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMACHAPTER - SEQUENCES AND SERIESEXERCISE - Section II - Assertion Reason Type
13 Videos
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMACHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise
129 Videos
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMACHAPTER - SEQUENCES AND SERIESEXERCISE - Chapter Test
60 Videos
- QUADRATIC EXPRESSIONS AND EQUATIONS BOOK - OBJECTIVE RD SHARMACHAPTER - QUADRATIC EXPRESSIONS AND EQUATIONS EXERCISE - Chapter Test
50 Videos
- SETSBOOK - OBJECTIVE RD SHARMACHAPTER - SETSEXERCISE - Chapter Test
30 Videos
Similar Questions
Let Sk, where k=1,2,....,100, denotes the sum of the infinite geometric series whose first term is k−1k! and the common ratio is 1k. Then, the value of 1002100!+100∑k=2∣∣(k2−3k+1)Sk∣∣ is....
View SolutionLet sk,k=1,2,3,…,100 denote the sum of the infinite geometric series whose first term is k−1⌊k and the common ratio is 1k. Then, the value of
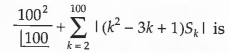 is
is
View Solution
Knowledge Check
Let Sk, where k=1,2,....,100, denotes the sum of the infinite geometric series whose first term is k.k−1k! and the common ratio is 1k. Then, the value of 1002100!+100∑k=2∣∣(k2−3k+1)Sk∣∣ is....
A3B2C1D0Let Sk, be the sum of an infinite geometric series whose first term is kand common ratio is kk+1(k>0) . Then the value of ∞∑k=1(−1)kSk is equal to
A1−4loge√2Bloge2−1C1−loge2D1−loge4Let SK be the sum of an infinte G.P. series whose first term is K and common ratio is KK+1(K>0). Then the value of ∞∑K=1(−1)KSK is equal to
Aloge4Bloge2−1Cloge2+1D1−loge4
Let Sk denote sum of infinite geometric series (K=1,2,3,.......), where first term is (k2−1) and common ratio is 1K, then the unit digit of the sum (∞∑k=1Sk2k−1), is
View SolutionFor k=2,3,…, let Sk denote the sum of the infinite G.P. whose first term is k2+k−2 and common ratio is 1k, then ∞Σk=1Sk2k=
View SolutionFind the value of 2551 S where S=50∑k=1kk4+k2+1
View SolutionFind the value of limn→∞n∑k=1k2+k−1(k+1)!.
View SolutionLet Sk be sum of an indinite G.P whose first term is 'K' and commmon ratio is 1k+1. Then Σ10k=1Sk is equal to _________.
View Solution
