If the graph of the function y = f(x) is symmetrical about the line x = 2, then
A
B
C
D
Video Solution
Text Solution
The correct Answer is:B
It the graphof f(x) is symmetrical about the line x = 2, then
for all .
|
Topper's Solved these Questions
- REAL FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - REAL FUNCTIONS EXERCISE - Section II - Assertion Reason Type
8 Videos
- REAL FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - REAL FUNCTIONS EXERCISE - Exercise
95 Videos
- REAL FUNCTIONS BOOK - OBJECTIVE RD SHARMACHAPTER - REAL FUNCTIONS EXERCISE - Chapter Test
60 Videos
- PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMBOOK - OBJECTIVE RD SHARMACHAPTER - PROPERTIES OF TRIANGLES AND CIRCLES CONNECTED WITH THEMEXERCISE - Chapter Test
55 Videos
- SCALAR AND VECTOR PRODUCTS OF THREE VECTORS BOOK - OBJECTIVE RD SHARMACHAPTER - SCALAR AND VECTOR PRODUCTS OF THREE VECTORS EXERCISE - Exercise
65 Videos
Similar Questions
Knowledge Check
If the graph of a function f(x is symmetrical about the line x = a, then
Af(a+x)=f(a−x)Bf(a+x)=f(x−a)Cf(x)=f(−x)Dnone of theseIf the garph of the function f(x)=ax3+x2+bx+c is symmetric about the line x = 2, then the value of a+b is equal to
A10B−4C16D−10If the function f(x) is symmetric about the line x=3, then the value of the integral I=∫8−2f(x)f(x)+f(6−x)dx is
A0B5C10D16
If the graph of y=f(x) is symmetrical about the lines x=1andx=2, then which of the following is true? (a)f(x+1)=f(x) (b) f(x+3)=f(x) (c)f(x+2)=f(x) (d) None of these
View SolutionIf the graph of the function
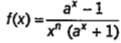 is symmetric about y-axis, then n is equal to :
is symmetric about y-axis, then n is equal to :
View SolutionThe graph of the function f(x)=−2−|x|
View SolutionIf f(x)=f(2a−x) then the graph of f(x) is symmetric about the line
View SolutionIf the graph of y=ax3+bx2+cx+d is symmetric about the line x=K then the value of a+K is (y is not a constant function)
View Solution
