Here we find a missing force by using the acceleration. In the overhead view of Fig. 5-4a, a 2.0 kg cookie tin is accelerated at 3.0 m/s2 in the direction shown by →a, over a frictionless horizontal surface. The acceleration is caused by three horizontal forces, only two of which are shown: →F1 of magnitude 10 N and →F2 of magnitude 20 N. What is the third force →F3 in unit-vector notation and in magnitude-angle notation?
The net force →Fnet on the tin is the sum of the tree forces and is related to the acceleration →a via Newton.s second law (→Fnet=m→a). Thus,
→F1+→F2+→F3=m→a, (5−6)
which gives us
→F3=m→a−→F1−→F2. (5−7)
Calculations: Because this is a two-dimensional problem, we cannot find →F3 merely by substituting the magnitudes for the vector quantities on the right side of Eq. 5-7. Instead, we must vectorially add m→a,−→F1 (the reverse of →F1), and −→F2 (the reverse of →F2), as shown in Fig. 5-4b. This addition can be done directly on a vector-capable calculator because we know both magnitude and angle for all three vectors. However, here we shall evaluate the right side of Eq. 5-7 in terms of components, first along the x axis and then along the y axis. Caution: Use only one axis at a time.
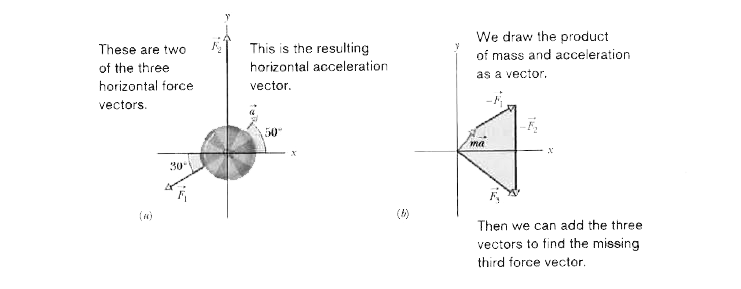
Figure 5-4 (a) An overhead view of two three horizontal forces that act on a cookie tin, resulting in acceleration →a.→F3 is not shown. (b) An arrangement of vectors m→a,−→F1, and −→F2 to find force →F3.
x components: Along the x axis we have
F3,x=max=F1,x−F2,x
=m(acos50∘)−F1cos(−150∘)−F2cos90∘.
Then, substituting known data, we find
F3,x=(2.0 kg)(3.0 m/s2)cos50∘−(10 N)cos(−150∘)
−(20 N)cos90∘
=12.5 N.
y comonents: Similarly, along the y axis we find
F3,y=may−F1,y−F2,y
=m(asin50∘)−F1sin(−150∘)−F2sin90∘
=(2.0 kg)(3.0 m/s2)sin50∘−(10 N)sin(−150∘)
−(20 N)sin90∘
=10.4 N.
Vector: In unit-vector notation, we can write
→F3=F3,xˆi+F3,yˆj=(12.5 N)ˆi−(10.4 N)ˆj
≈(13 N)ˆi−(10 N)ˆj.
We can now use a vector-capable calculator to get the magnitude and the angle of →F3. We can also use Eq. 3-6 to obtaiin the magnitude and the angle (from the positive direction of the x axis) as
F3=√F23,x+F23,y=16 N
and θ=tan−1(F3,y)F3,x=−40∘.
Topper's Solved these Questions
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - CHECKPOINT
5 Videos
- FORCE AND MOTION - I BOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION - I EXERCISE - PROBLEMS
52 Videos
- FLUIDSBOOK - RESNICK AND HALLIDAYCHAPTER - FLUIDSEXERCISE - PRACTICE QUESTIONS (Integer Type )
5 Videos
- FORCE AND MOTION-IIBOOK - RESNICK AND HALLIDAYCHAPTER - FORCE AND MOTION-IIEXERCISE - PRACTICE QUESTIONS (Integer Type)
1 Videos
Similar Questions
Knowledge Check
A body is at rest under the action of three forces, two of which are →F1=4ˆi,→F2=6ˆj, the third force is
A4ˆi+6ˆjB4ˆi−6ˆjC−4ˆi+6ˆjD−4ˆi−6ˆjTwo forces →F1=500N due east and →F2=250N due north have their common initial point. →F2−→F1 is
A250√5N,tan−1(2)WofNB250N,tan−1(2)W of NCZeroD750N,tan−1(3/4)NofWTwo forces act on a 4.5 kg block resting on a frictionless surface as shown in the given figure. What is the magnitude of the horizontal acceleration of the block ?
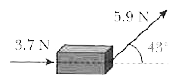 A1.8 m/s2B1.2 m/s2C0.82 m/s2D3.2 m/s2
A1.8 m/s2B1.2 m/s2C0.82 m/s2D3.2 m/s2
Two forces →F1=3ˆi+4ˆj and →F2=3ˆj+4ˆk are acting simultaneously at a point. What is the magnitude of the resultant force ?
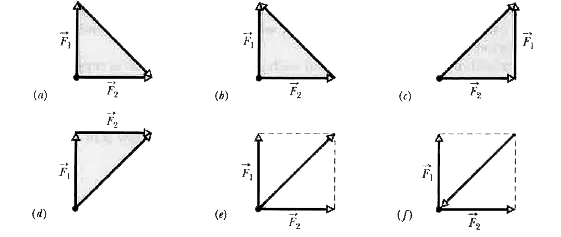
View SolutionWhich of the figure's six arrangements correctly show the vector addition of forces →F1 and →F2 to yield the third vector, which is meant to represent their net force →Fnet ?
View SolutionAn object rests on a horizontal frictionless surface. A horizontal force of magnitude F is applied. This force produces an acceleration
View SolutionThree forces are acting on a particle of mass m initially in equilibrium if the first two forces (→R1and→R2) are perpendicular to each other and suddenly the third force (→R3) is removed, then the magnitude of acceleration of the particle is:
View SolutionTwo forces −→F1and−→F2 are acting at right angles to each other , find their resultant ?
View Solution
