Two forces →F1=500N due east and →F2=250N due north have their common initial point. →F2−→F1 is
250√5N,tan−1(2)WofN
250N,tan−1(2)W of N
Zero
750N,tan−1(3/4)NofW
The correct Answer is:A
To solve the problem of finding →F2−→F1, we will follow these steps:
Step 1: Identify the Forces
We have two forces:
- →F1=500N due east
- →F2=250N due north
Step 2: Represent the Forces as Vectors
In a Cartesian coordinate system:
- →F1 can be represented as (500,0) (since it acts along the x-axis).
- →F2 can be represented as (0,250) (since it acts along the y-axis).
Step 3: Calculate →F2−→F1
Now we will calculate →F2−→F1:
→F2−→F1=(0,250)−(500,0)=(0−500,250−0)=(−500,250)
Step 4: Calculate the Magnitude of the Resultant Vector
To find the magnitude of the resultant vector →F2−→F1:
|→F2−→F1|=√(−500)2+(250)2
Calculating this:
|→F2−→F1|=√250000+62500=√312500=250√5N
Step 5: Calculate the Direction of the Resultant Vector
To find the direction (angle θ) of the resultant vector, we can use the tangent function:
tanθ=OppositeAdjacent=250500=12
Thus,
θ=tan−1(12)
This angle is measured from the negative x-axis (due west) towards the positive y-axis (due north).
Final Answer
The resultant vector →F2−→F1 has a magnitude of 250√5N and an angle of tan−1(0.5) north of west.
---
We have two forces:
- →F1=500N due east
- →F2=250N due north
In a Cartesian coordinate system:
- →F1 can be represented as (500,0) (since it acts along the x-axis).
- →F2 can be represented as (0,250) (since it acts along the y-axis).
Now we will calculate →F2−→F1:
→F2−→F1=(0,250)−(500,0)=(0−500,250−0)=(−500,250)
To find the magnitude of the resultant vector →F2−→F1:
|→F2−→F1|=√(−500)2+(250)2
Calculating this:
|→F2−→F1|=√250000+62500=√312500=250√5N
To find the direction (angle θ) of the resultant vector, we can use the tangent function:
tanθ=OppositeAdjacent=250500=12
Thus,
θ=tan−1(12)
This angle is measured from the negative x-axis (due west) towards the positive y-axis (due north).
The resultant vector →F2−→F1 has a magnitude of 250√5N and an angle of tan−1(0.5) north of west.
Topper's Solved these Questions
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Subjective
28 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
Similar Questions
A particle of m=5kg is momentarily at rest at x=att=. It is acted upon by two forces →F1 and →F2. Vec(F)1=70ˆj N. The direction and manitude of →F2 are unknown. The particle experiences a constant acceleration, →a,in the direction as shown in (figure) Neglect gravity.
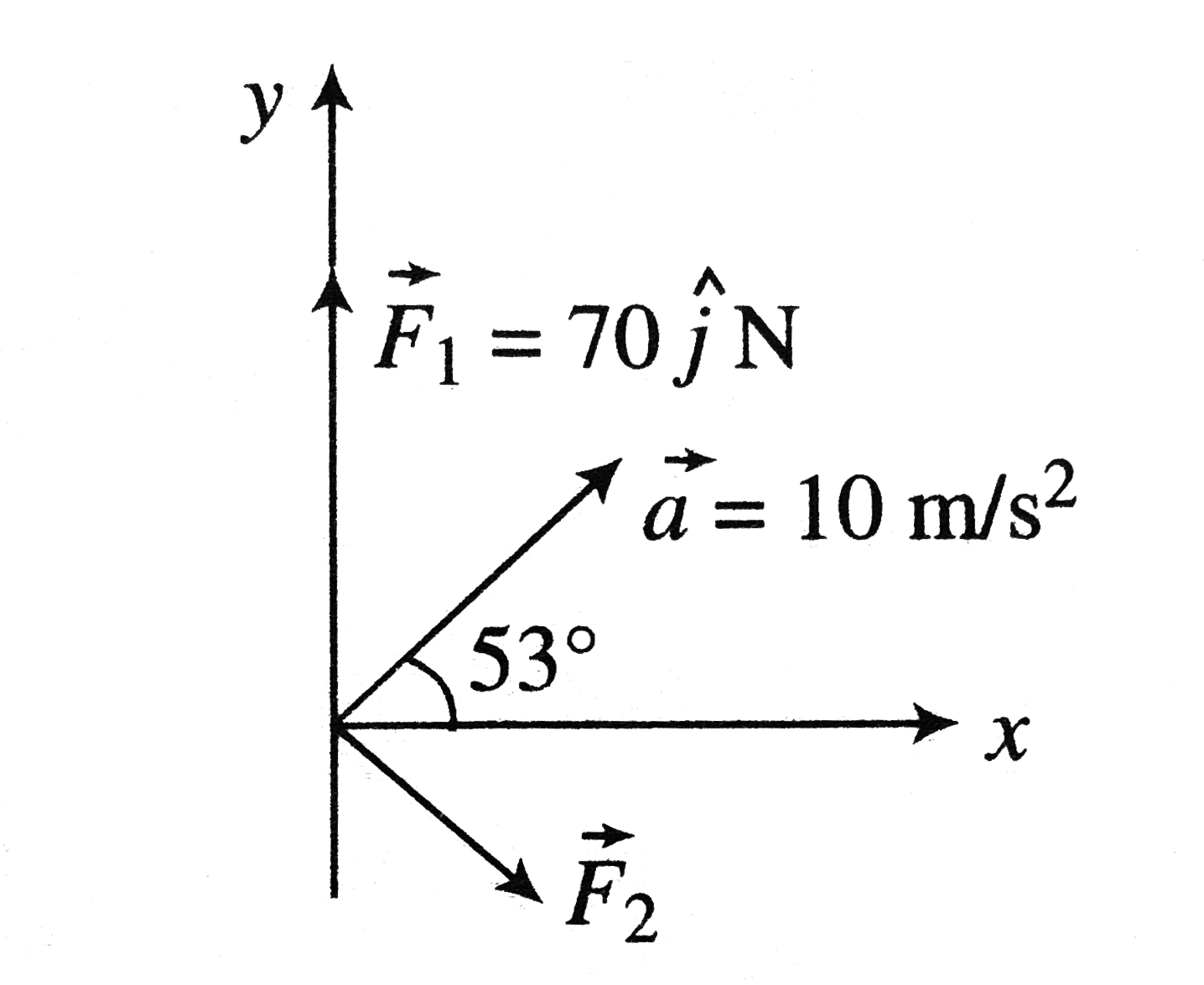
a.Find the missing force →F2.
b. What is the velocity vector of the particle at t=10s?
c. What third force, →F3 is required to make the acceleration of the particle zero? Either give magnitude and direction of →F3 or its components.
View SolutionA slab is subjected to two forces →F1and→F2 of same magnitude F as shown in the figure . Force →F2 is in XY-plane while force F1 acts along z-axis at the point (2→I+3→j) .The moment of these forces about point O will be :

View Solution
Knowledge Check
A body is moving under the action of two force −−−−−−−−−−→F1=2ˆi−5ˆj , −−−−−−−−−−→F2=3ˆi−4ˆj . Its velocity will become uniform under a third force −→F3 given by.
A5ˆi−9ˆjB−5ˆi−9ˆjC5ˆi+9ˆjD−5ˆi+9ˆjA body is moving under the action of two force −−−−−−−−−−→F1=2ˆi−5ˆj , −−−−−−−−−−→F2=3ˆi−4ˆj . Its velocity will become uniform under a third force −→F3 given by.
A5ˆi−ˆjB−5ˆi−ˆjC5ˆi+ˆjD−5ˆi+9ˆj
STATEMENT -1 : Net force on a particle w.r.t an inertial frame does notdepend on the velocity of the intertial frame. ,
STATEMENT - 2 : If net force on A is →FA and net force on B is →FB then force on A w.r.t B is (ven(F)A−→FB) .
STATEMENT - 2 : If acceleration of A is →aA and acceleration of B is →aB then, the acceleration of A w.r.t B is (→aA−→aB)
View SolutionA body placed in free space, is simultaneously acted upon by three forces −→F1,−→F2 and −→F3. The body is in equilibrium and the forces −→F1 and −→F2 are known ot be 36 N due north and 27 N due east respectively. Which of the following best describes the force −→F3?
View SolutionThree forces →F1,→F2 and →F3 are represented as shown. Each of them is equal magnitude.
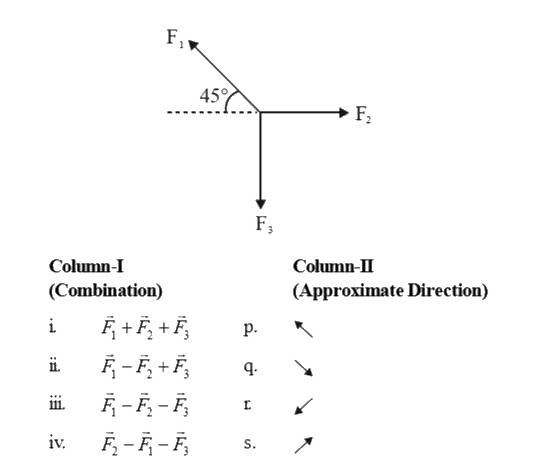
Now match the given columns and select the correct option from the codes given below.
View SolutionAB is a quarter of a smooth horizontal circular track of radius R,A particle P of mass m moves along the track from A to B under the action of following forces:

−→F1=F (always towards y-axis)
−→F2=F (always towards point B)
−→F3=F (always along the tangent to path AB)
−→F4=F (always towards x-axis)
ColumnI ColumnII(A)Work done by−→F1 (P)√2FR(B)Work done by−→F3 (Q)FR(C)Work done by−→F3 (R)πFR2(D)Work done by−→F4 (S)2FRπ
Select correct alternative :-
View SolutionFour forces act on a particle as shown in the figure such that net force is zero. Then consider following statement :−
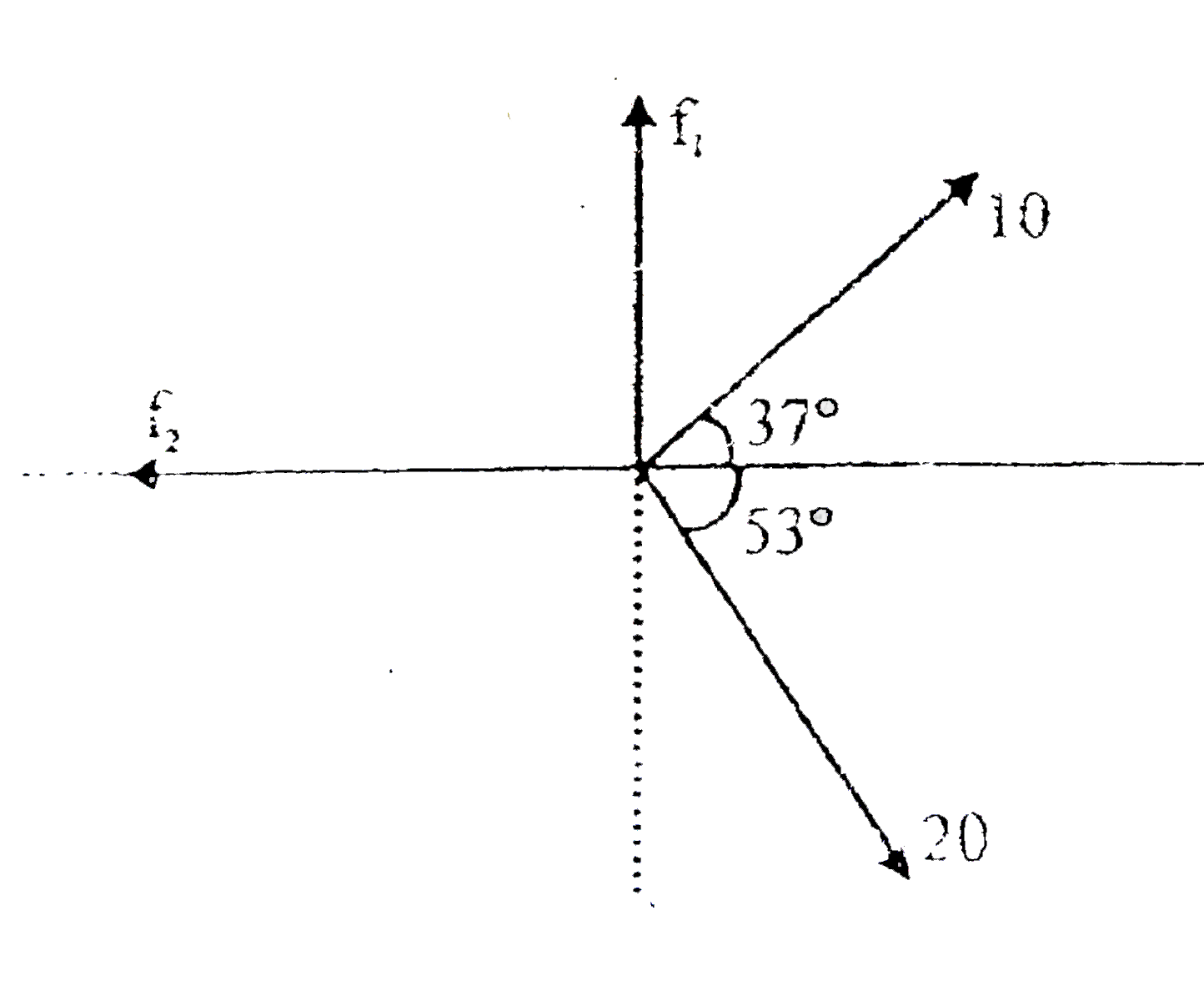
(A) Magnitude of →f1 is 10N
(B) Magnitude of →f1 is 20N
(C) Magnitude of →f2 is 10N
(D) Magnitude of →f2 is 20N
View SolutionA bouy is attached to three tugboats by three ropes. The tugboats are engaged in a tug-0f-war. One tugboat pulls west on the buoy with a force →F1 of magnitude 1000N. The second tugboat pulls south on the buoy with a force →F2 of magnitude 2000N. The third tugboat pulls northeast (that is, half way between north and east), with a force →F3 of magnitude 2000N
a. Express each force in unit vector from (ˆi,ˆj).
b. Calculate the magnitude of the resultant force.
View Solution